-3
$\begingroup$

I am struggling to grasp the concept of partial pressure and vapor pressure.

Consider a sealed container filled with water and a bit of air that is sufficiently dry placed in a room of temperature 25 degrees celsius and pressure of 1atm. The container is initially in thermal and mechanical equilibrium with the surroundings.

The water in the container will spontaneously evaporate until water and steam chemical potentials match (diffusive equilibrium).

However, I don't understand why this process happens.

Before evaporation, the container with air initially starts at state 1 in the below phase diagram. In this state, water, air and surroundings are all in mechanical equilibrium with each other right? Since state 1 is located in liquid region, then all air particles should spontaneously condense to water particles?

Improve this question
$\endgroup$
2
  • 3
    $\begingroup$ This is the phase diagram for water. Not water plus air. Your assumption the air should condense is false. We can still use it to describe the behaviour when air is present if you recognise that, in the gas phase, molecules don't interact much. So the water vapour pressure is independent of the other gases present. $\endgroup$
    – matt_black
    Commented Apr 2, 2023 at 9:47
  • $\begingroup$ Not true an inert gas is an additional component in the phase rule. $\endgroup$
    – jimchmst
    Commented Apr 3, 2023 at 1:25

1 Answer 1

Reset to default
1
$\begingroup$

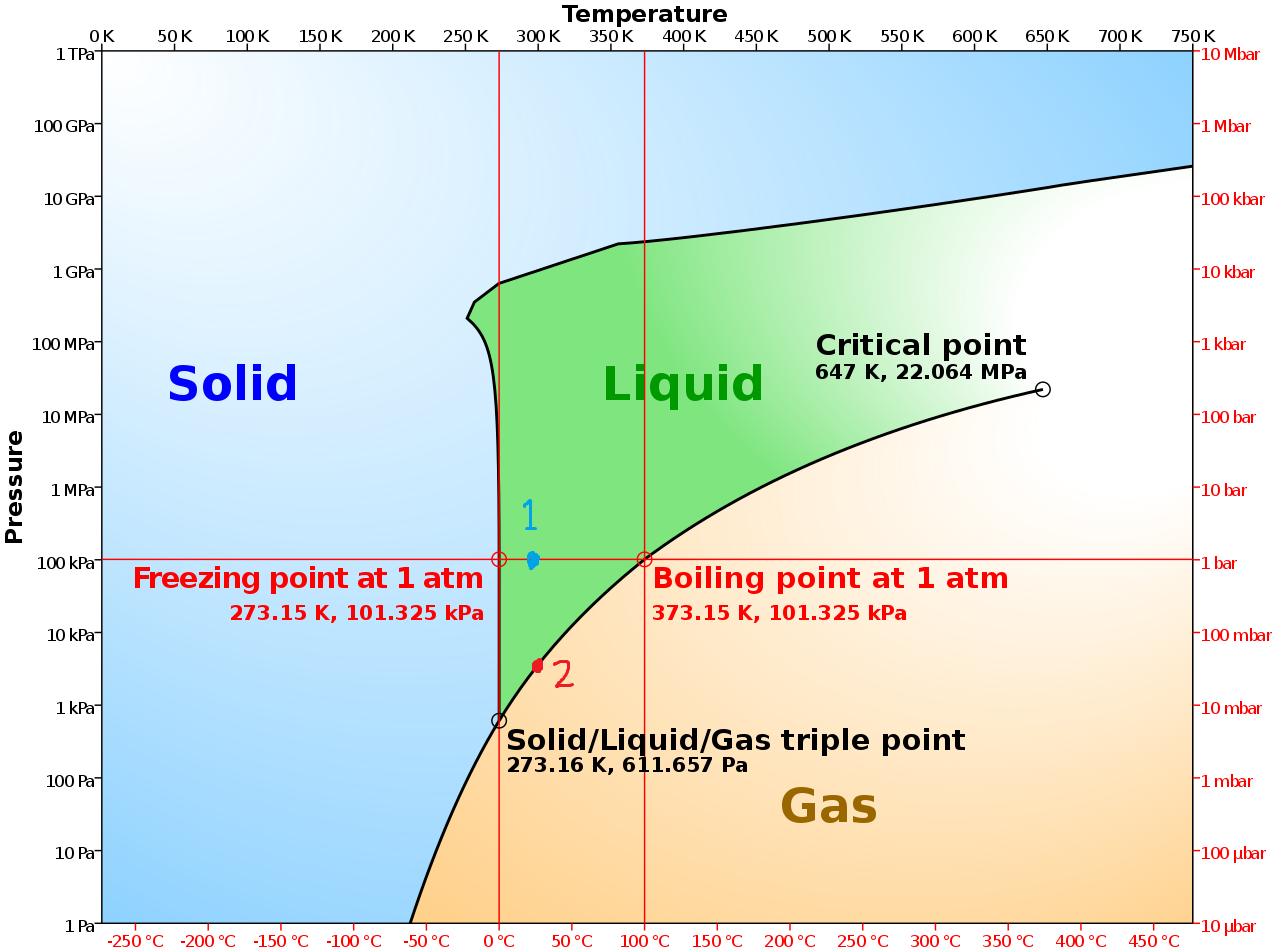
The diagram is for the system without air, so at equilibrium, vapor pressure is equal to total pressure (if there is the gaseous phase).

At $\pu{25 ^{\circ}C}$, all water eventually evaporates, if pressure is kept below vapor pressure at $\pu{25 ^{\circ}C}$, or condenses if pressure is kept above the vapor pressure.

The boiling point refers to the total pressure regardless of the gaseous phase composition.

On the other hand, pressure of gaseous mixture cannot be generally used to determine position on the phase diagram. Water at room temperature would evaporate to ambient air, if water vapor partial pressure is lower than (saturated) vapor pressure, in spite of the total pressure being much higher than vapor pressure.

Total pressure can be used for equilibrium between condensed phases.

Reaction to feedback:

If there is solely water vapor in the gaseous phase of the container, and if external pressure is higher than vapor pressure at the given kept temperature, these processes happen:

  • External pressure would compress vapor until pressures equilibrate.
  • This compression adiabatically heats up the vapor.
  • As vapor is oversaturated, it starts condensing, releasing heat.
  • This may continue until it reaches temperature when vapor pressure equals to external pressure.
  • As heat dissipates to surrounding, vapor keeps being oversaturated and condensing, until there is no vapor left.

With air present, total system pressure keeps mechanical equilibrium with external pressure and liquid water keeps partial vapor pressure equal to (saturated) vapor pressure at the given kept temperature.

If there is initially air in the system with pressure equal to external ambient pressure $\pu{1 atm}$, water will evaporate until it's partial pressure reaches vapor pressure and system will expand to maintain the total pressure equal to ambient pressure (assuming isothermic + isobaric conditions).

Improve this answer
$\endgroup$
3
  • $\begingroup$ At equilibrium, the air in the container is full of water molecules (ie. saturated), right? If water vapor and water coexist in the container, then the pressure of the whole container must be equal to vapor pressure, no? So, at equilibrium, the container is not in mechanical equilibrium with the surroundings since its pressure is less than 1atm? $\endgroup$
    – Ray Siplao
    Commented Apr 2, 2023 at 7:52
  • $\begingroup$ You cannot assume there is and there is not air at the same time. It would be "double thinking" from the novel 1984. // BTW, TD equilibrium consists of thermal, radiative, chemical and mechanical equilibrium. $\endgroup$
    – Poutnik
    Commented Apr 2, 2023 at 8:26
  • $\begingroup$ Answer updated. $\endgroup$
    – Poutnik
    Commented Apr 2, 2023 at 8:47

Not the answer you're looking for? Browse other questions tagged or ask your own question.