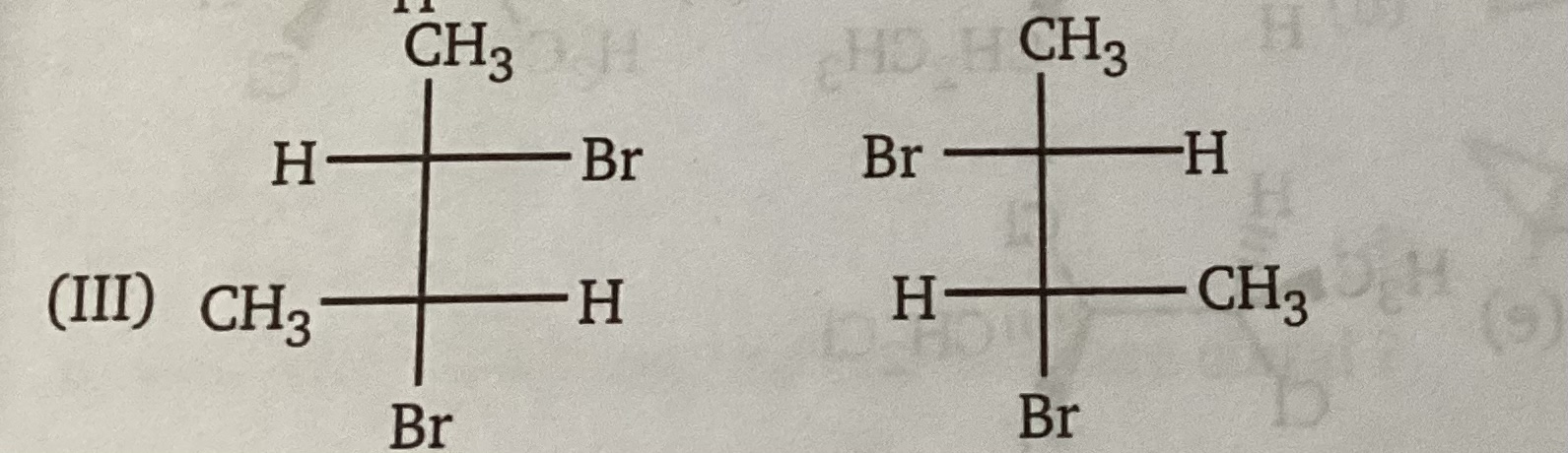Why is this example not considered enantiomer but identical? And as it is identical what would be the enantiomer pair?
-
$\begingroup$ Have you tried R/S configuration? $\endgroup$– Natasha JCommented Jan 24, 2023 at 3:04
-
$\begingroup$ Yes i have . But my question here is why are these identical $\endgroup$– AnonymousCommented Jan 24, 2023 at 5:56
-
1$\begingroup$ Almost a duplicate: chemistry.stackexchange.com/q/13730/72973 $\endgroup$– Karsten ♦Commented Jan 24, 2023 at 14:09
-
$\begingroup$ Regardless if you just started to study organic chemistry, or not; there is nothing wrong to go to the shelf and to pick a real model kit (an example). $\endgroup$– ButtonwoodCommented Jan 26, 2023 at 17:17
1 Answer
Try 3D pin and ball visual model from home available items, creating respective 3D models according to the Fisher projections of your image. Then try to superimpose them, rotating the whole models or the bonds. If you succeed, you will know why. If not, you will know they are not identical.
You can formally rotate the bottom part of molecules around the central $\ce{C-C}$ bond to have the side methyl on the bottom. Then it is clear it is the case of the same symmetry as for optically inactive meso tartaric acid. Both stereogenic centers mutually cancel each other. The molecule has a plane of symmetry and therefore is not optically active, as it's mirror image can be superimposed with the original.
The suggested turning does not change configuration. Mirror images of your case and of meso tartaric acid can be turned around the bonds to become original image, what disqualifies them from being enantiomers.
With bonds properly rotated, the upper molecule half is the mirror image of the lower half. If you mirror the whole molecule, the upper half becomes the lower half and vice versa, leading to the identical molecule. That is revealed if you turn the mirror image by half turn clockwise.
With methyls on top and the bottom, the enantiomers would be those isomers with H and Br atoms alternating sides. They form mirror twins that cannot be superimposed. Again, they have the same symmetry as optically active isomers of tartaric acid.
If I consider flipping the molecule that would result in every pair of enantiomers being identical.
I have suspicion you take the charts as 2D flat structures, what is wrong. Fischer projection on your image is convention to display 3D bond structure, with the vertical bonds arranged in a way of bending top and bottom away from you, while horizontal bonds are stretching toward you. Like if there was a horizontal wooden log behind and you tried to wrap the molecule around it. Remember four C bonds are not rectangular in a single plane, but are pointing to the corners of a tetraedr.
-
$\begingroup$ The answer of the question is that these are identical. My answer was that these are enantiomers but it is wrong, so what I am not understanding is that how are these identical. If I consider flipping the molecule that would result in every pair of enantiomers being identical. $\endgroup$ Commented Jan 24, 2023 at 8:17
-
$\begingroup$ Sorry, I still don’t get it. Just as you said these are 3D arrangements , if I turn this into a wedge and dash structure to visualise it in 3D space I am still getting two different molecules which are mirror images. How are they identical. I am sorry I really don’t understand. $\endgroup$ Commented Jan 24, 2023 at 8:56
-
$\begingroup$ Imagine a ball, laid on a table. Imaging joining 4 other balls by 4 pins, pointing up, down, left, right. that is how it is drawn on your image above. But the reality is like if you lift this flat model by hands and force up and down pins to point little down and left / right pins little up. Follow also the Fischer projection link. $\endgroup$– PoutnikCommented Jan 24, 2023 at 9:01
-
$\begingroup$ Properly rotated, the upper molecule half is the mirror image of the lower half. If you mirror the whole molecule, the upper half becomes the lower half and vice versa, leading to the identical molecule. $\endgroup$– PoutnikCommented Jan 24, 2023 at 9:16
-
2$\begingroup$ I see it now, Thank you. What I was doing wrong was trying to match upper half to upper and not considering rotation of the molecule. $\endgroup$ Commented Jan 24, 2023 at 9:24