Relation between thermodynamic reversible process and reversible reaction

Question

I know it seems to be a weird question. But for long I have been thinking whether there is any relation between thermodynamic reversible process and reversible reaction. Do they have any connection and if so how?

Answer 1

To complement other answers, I provided here definitions extracted from official IUPAC sources:

reversible process

A definition comes via the concept of entropy (Ref. 1):

Quantity the change in which is equal to the heat brought to the system in a reversible process at constant temperature divided by that temperature. Entropy is zero for an ideally ordered crystal at $\pu{0 K}$. In statistical thermodynamics $$S=k \ln W$$ where $k$ is the Boltzmann constant and $W$ the number of possible arrangements of the system.

The second sentence is a formulation of the third law, while the last is a definition of entropy viewed from the context of statistical thermodynamics. The first sentence describes the thermodynamic entropy. Coupled with the second law expressed as an inequality relation between heat and entropy,

$$\Delta S \ge \frac{q}{T} \tag{const. T}$$

it provides a functional definition of a reversible process (it is a process that maximizes $q$).

reversible reaction

The definition provided by IUPAC is related to microscopic reversibility:

In a reversible reaction, the mechanism in one direction is exactly the reverse of the mechanism in the other direction. This does not apply to reactions that begin with a photochemical excitation.

Such a definition implies thermodynamic reversibility.

References

1. IUPAC. Compendium of Chemical Terminology, 2nd ed. (the "Gold Book"). Compiled by A. D. McNaught and A. Wilkinson. Blackwell Scientific Publications, Oxford (1997). Online version (2019-) created by S. J. Chalk. ISBN 0-9678550-9-8. https://doi.org/10.1351/goldbook.

Answer 2

Reversible reaction

According to Merriam Webster, a reversible reaction is:

a reaction that takes place in either direction according to conditions (as the formation of hydriodic acid by union of hydrogen and iodine or its decomposition into these elements)

For this type of reaction, you would use a double-harpoon to write the chemical equation:

$$\ce{H2(g) + I2(g) <=> 2HI(g)}$$

and would be able to write an equilibrium constant expression

$$K = \frac{[\ce{HI}]^2}{[\ce{H2}][\ce{I2}]}$$

with [] denoting activity or fugacity. If you start with pure reactants, the reaction would go forward (decrease in reactants, increase in products). If you start with pure products, the reaction would go backwards (increase in reactants, decrease in products). Once the reaction has reached equilibrium, concentrations would no longer change.

In any case, reactions in both directions would occur at the particular level. When the reaction is at equilibrium, you just won't know at the macroscopic level and would have to disturb the equilibrium to see macroscopic changes again.

Reversible process

Again according to Merriam Webster, a reversible process is:

an ideal process or series of changes of a system which is in complete equilibrium at each stage such that when the process is reversed each of the changes both internal and external is reversed but with the amount of transferred energy unaltered

Applied to chemical reactions, a reversible process is one where the Gibbs energy is constant throughout (or where the entropy of the universe is not increased by it). We would not expect any macroscopic changes (the process is at equilibrium), which why this is an ideal process (not real). The closest real process is one where the system is near equilibrium, and the increase in entropy of the universe is minimal.

What does IUPAC say?

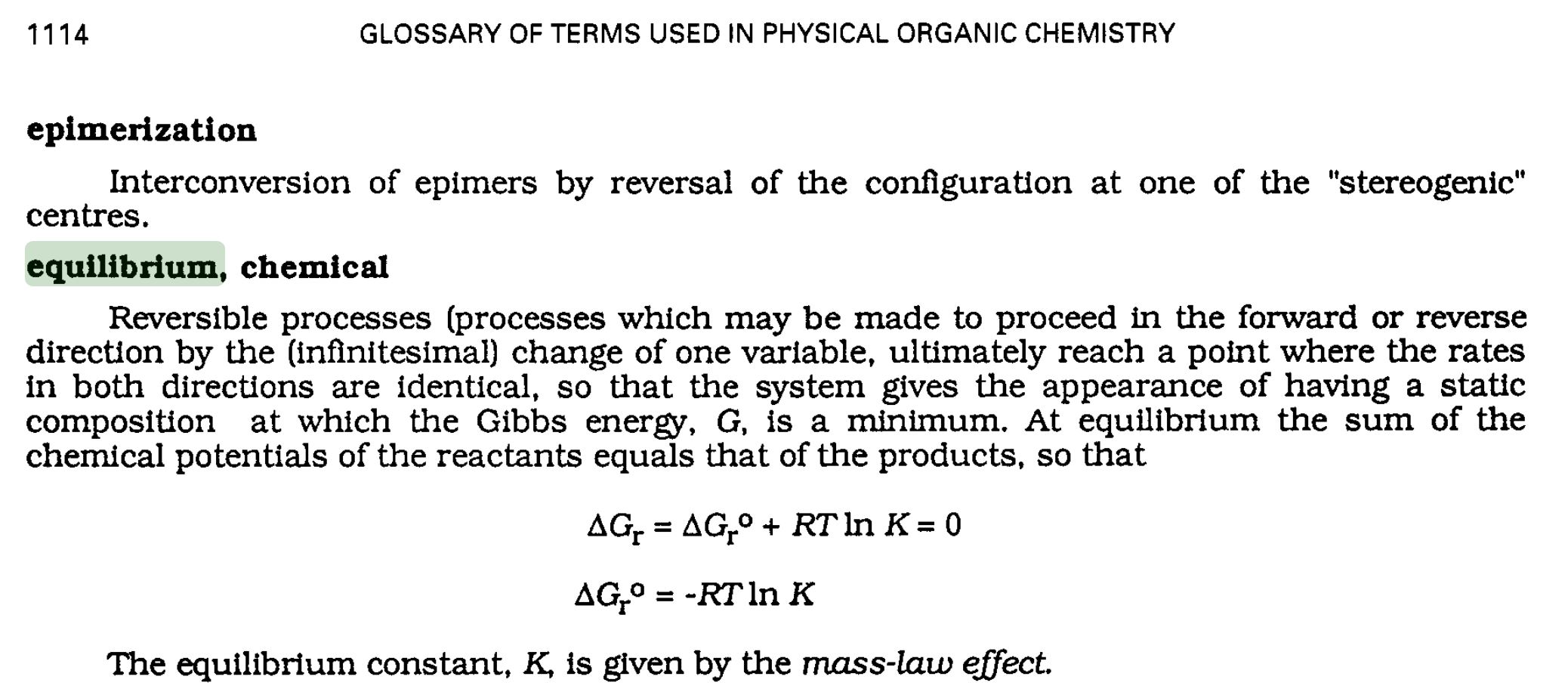
The IUPAC Gold book makes reference to a paper (DOI: https://doi.org/10.1351/pac199466051077) offering a glossary of terms used in physical organic chemistry. In their definition of chemical equilibrium, it says:

While the definition of reversible process matches the one I cite above, and they use the same notation for the equilibrium reaction. However, the connection between reversible process and chemical equilibrium surprises me. When a reaction approaches equilibrium, the Gibbs energy does change, and it will not return to the initial state unless work is done on the system. Maybe the confusion regarding reversible process vs. reaction stems in part from this.

Answer 3

I agree with both the MW and the IUPAC definitions, but perhaps you can help me checking if I am getting this right.

If a reactive system involving a reaction that is reversible in the chemical sense (i.e. it "goes both ways" around its equilibrium (i.e. the activities ( ~ concentrations) of its components can match the value of the equilibrium constant $\mathrm{K(T)}$ ), then as a whole there are changes within the system that satisfy $\Delta{S_{system}} = 0$ (i.e. reversible in a thermodynamic sense) because the reversible reactions within have molar changes of entropy + $\Delta S$ (for the forward reaction, typically non-zero) and $- \Delta S$ (for the backward reactions, with a minus sign in front), with forward and backward reactions at the same rate (dynamic equilibrium).

In a previous reply, I attempted the following wording, which perhaps is more detailed but does not make explicit the distinction between entropy of reaction and entropy of the system:

With $\Delta G = \Delta H - T\Delta S$ for a reversible reaction, where $\Delta G$ is the change in Gibbs energy, $\Delta H$ the enthalpy of reaction and $\Delta S$ the change in entropy (and $T$ and $P$ constant), I think what is going on then for a system where a dynamic equilibrium is established between backward and forward reaction, which could qualify as a reversible process, once the equilibrium is reached. $T$ sets the value of the equilibrium constant and hence $\Delta G$ for these conditions. $\Delta H$ and $\Delta S$ typically are considered to be fairly constant (you could see how $\Delta S$ may in fact be dependent on temperature though, just through different vibration energies of different bonds).

In the special case where $\Delta G = 0$ (when equilibrium constant $K = 1$), with $\Delta G = \Delta H - T\Delta S$, one has to assume that the $\Delta S$ is fully reversible, which I think assumes faultless conversion of $T\Delta S$ to $\Delta H$ and vice-versa, between reacting species.