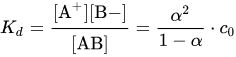In a 20wt% ammonia solution, what are the species in solution?

Question

I have bought a 20wt% ammonia solution. It is described as having a specific gravity of 0.92.

I worked out its Molarity earlier today, and I think it was approximately 10M

My question can I assume that the solute remains effectively as ammonia molecules because the dissociation constant is so small?

In my ammoniated solution I have:

NH3 + H2O and I want to know what concentration of NH4$^+$ and OH$^-$ I have.

So my equation is NH$_3$ + H$_2$O <<<>>> NH$_4^+$ + OH$^-$

I have a dissociation constant of ~ 10$^{-5}$.

So if I assume that from 1 mole of ammonia, x moles of ions form I get

10$^{-5}$ = $\frac{x^{2}}{1-x}$, so x = 3.16 x 10$^{-3}$ i.e.there are 0.003 moles of ammonium ions in solution.

Could someone please check? If this is the case, I am going to ignore their presence

Thank you

Answer 1

Thanks for your question. Your equation and calculations are correct. First, I have to say that the variable in equilibrium equations is concentration. By multiplying concentration into volume of your solution, you can compute the number of moles of the ion or extra. So in your example of ammonia solution, by considering the concentration of ammonia be 1M and equilibrium constant be equals to 1.8 * 10^-5, the concentration of ammonium ion would be calculated 0.00423M and the concentration of remaining ammonia after dissociation would be (1-0.00423= 0.99577M). We can express this amount of dissociation by dissociation degree. I think this is what you are looking for. It is the ratio of dissociated solute to the entire solute that had been dissolved. In the 1M Ammonia solution, the dissociation degree is 0.42% (just a note, in ammonia solution, ammonia doesn't dissociate. Actually, water will dissociate and acts like an acid by giving a proton to Ammonia.) If I understand correctly, your claim was this that, Whenever the dissociation constant of an acid or base be law, the dissociation degree will be law too(most of the solute remain undissociated). That seems to be correct, but it's not! Actually, the dissociation degree not only depends on equilibrium constant, but also to solute concentration. The equation bellow demonstrate the relation between dissociation degree, initial solute concentration and equilibrium constant that is called the law of dilution (https://en.wikipedia.org/wiki/Law_of_dilution).

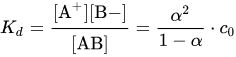
Where the square brackets denote concentration, and c0 is the total concentration of electrolyte and the degree of dissociation of a weak electrolyte is α. Let's see how α percent change by concentration of ammonia solution. I have solved that equation for several concentrations as you can observe below.

11M (~20% weight percent) : 0.128%

10M : 0.135%

9M : 0.142%

8M : 0.15%

7M : 0.161%

6M : 0.17%

1M : 0.42%

0.1M : 1.34%

0.01M : 12.6%

As you can observe the degree of dissociation is increased by dilution of ammonia solution. So, for your propose you have to consider concentration too.

One last thing is, these calculations are only assumptions of what concentration is going to be measured. Divisions may observe when another salt for example is in solution. For more precise computing of the degree of dissociation and extra, you need to consider other possible equilibrium too.

Take care about reactions with ammonia, that is capable to form explosive and or toxic compounds in some circumstances.

A site about degree of dissociation: https://www.nagwa.com/en/explainers/950137956236/