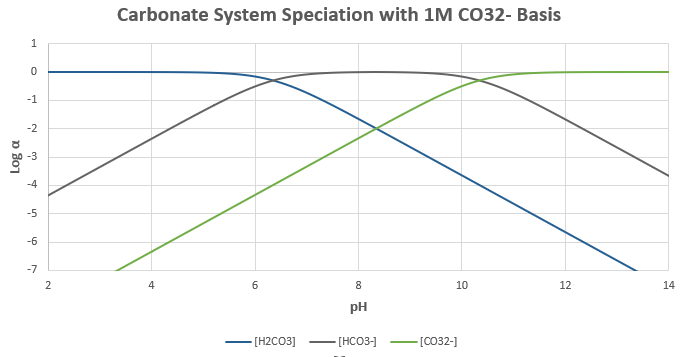
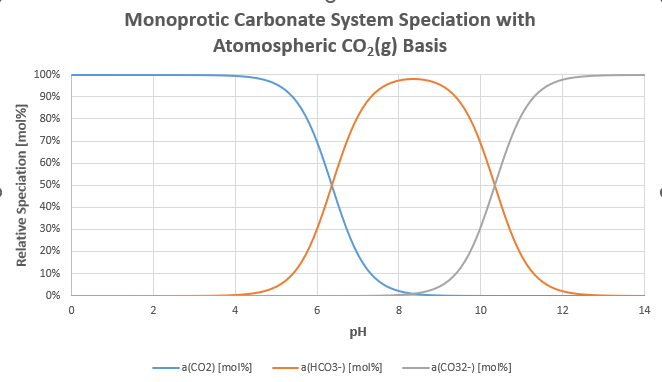
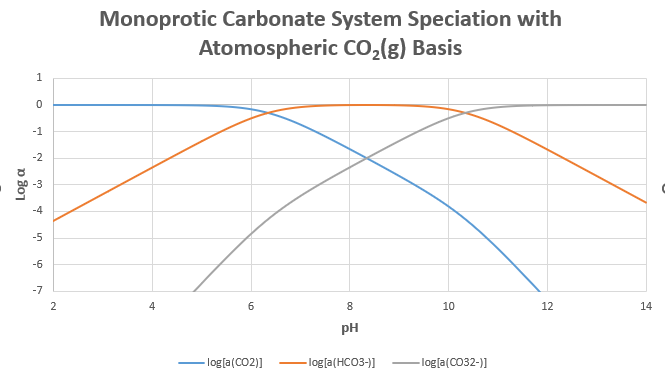
I did Environmental Water Chemistry as part of my undergraduate course where we quantified pH-speciation for the full carbonate system and I got it right according to my tutor. My speciation profile is as seen below:
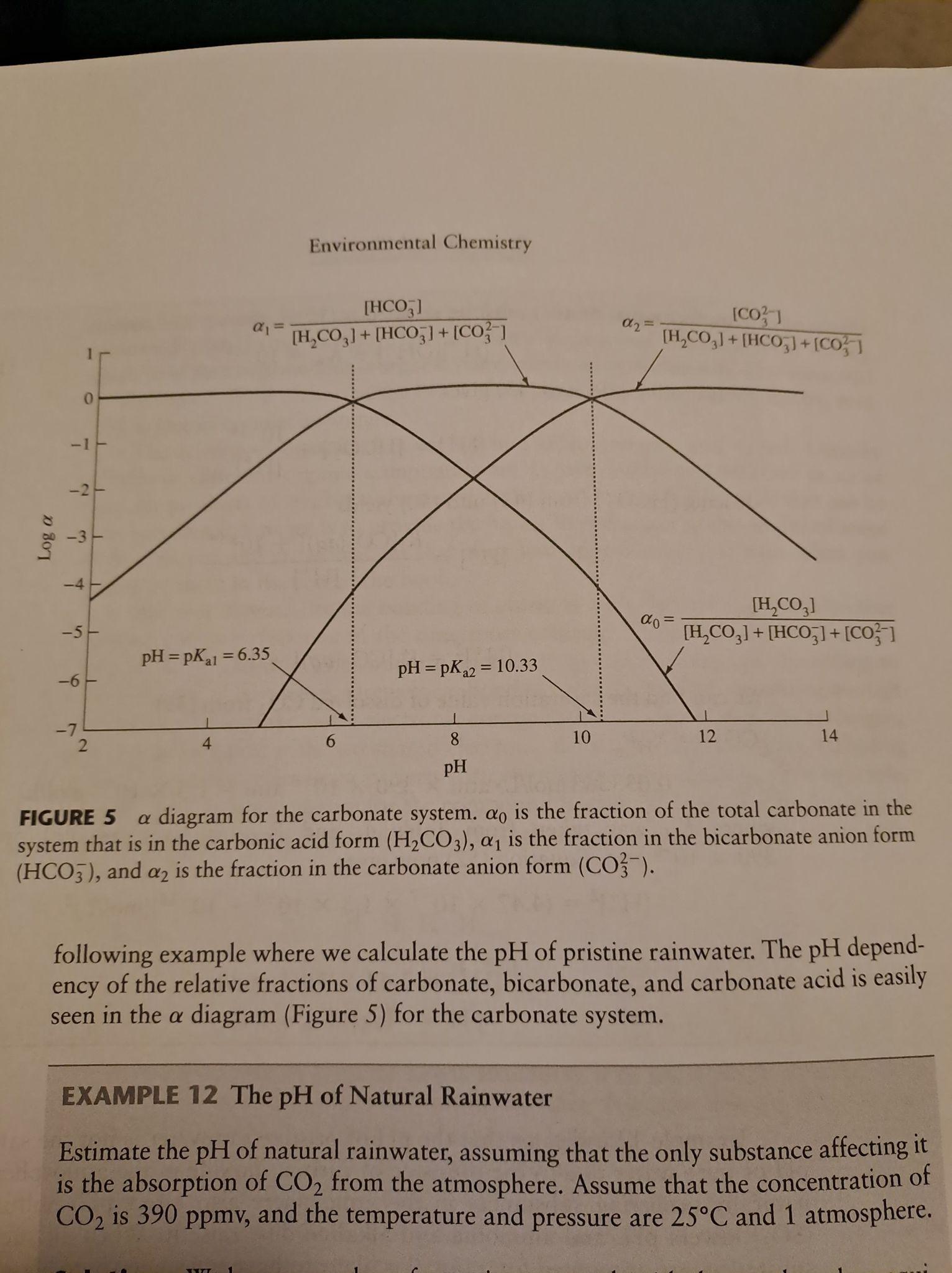
I've used this graph in other applications and have used it as a template with other speciation diagrams (i.e. chlorine and ammonia speciation etc.) but have recently found out that what I did for the carbonate system is actually wrong! I find this topic extremely fascinating and was reading about it in Masters et al. "Introduction to Environmental Engineering and Science" (p. 69) where I found their carbonate speciation plot as seen below:
Note that the carbonic acid trends ("$H_2CO_3$" in mine, "$\alpha_0$" in Masters et al) do not have the same curves, this is especially noticeable at the high pH values where the trend declines to zero in Masters et al but not mine. The carbonate species ("$CO_3^{2-}$" in mine, "$\alpha_2$" in Masters et al) also begin at different points. What's going on?!
Note, as I understand it, the system at 25◦C is defined by the following equations:
• Water-based Ions ($H^+\,and\,OH^-$): Formed from the dissociation of water with changing system pH. $H_2O_{l} \rightleftharpoons H_{(aq)}^+ + OH_{(aq)}^-$
• Carbonic Acid ($H_2CO_3$): Diprotic acid (which can lose two protons or hydrogen ions) formed from aqueous $CO_2$ dissolution. $CO_{2(aq)} + H_2O_{(l)} \rightleftharpoons H_2CO_{3(aq)} \rightleftharpoons H_{(aq)}^+ + HCO_{3(aq)}^-$
• Bicarbonate Ion ($HCO_3^-$): As above reaction, the bicarbonate ion is a protic acid formed from the dissolution of $H_2CO_3$. $H_2CO_{3(aq)} \rightleftharpoons H_{(aq)}^+ + HCO_{3(aq)}^-$
• Carbonate Ion ($CO_3^{2-}$): Formed from the dissolution of HCO−3 (assumed no dissolution from CaCO3(s) if the aqueous solution is in contact with calcaerous waters (i.e. water-bodies containing limestone based corals).
$HCO_{3(aq)}^- \rightleftharpoons H_{(aq)}^+ + CO_{3(aq)}^2-$
The equilibria for all the above reactions can be determined as per the below set of equations respectively:
$[H_{(aq)}^+] [OH_{(aq)}^-] = K_w = 1 \times 10^{-14} mol^2/L^2$
$\frac{[H_{(aq)}^+] [HCO_{3(aq)}^-]}{[CO_{2(aq)}]} = K_1 = 4.47 \times 10^{-7} mol/L$
$\frac{[H_{(aq)}^+] [CO_{3(aq)}^{2-}]}{[HCO_{3(aq)}^-]} = K_2 = 4.68 \times 10^{-11} mol/L$
Using these relationships I derived the trends in my spreadsheet which Ive tried going back to do the logic for but I just can't seem to get the same answer as the book and I don't know why... The trends I put in my spreadsheet were based on the following equation rearrangements (although I know their wrong from cross-referencing with the book but annoyingly don't know how I originally derived them as it was some years ago):
pH vs $H_2CO_3$ Speciation = $LOG(\frac{[H^+][CO_3^2]}{[H^+]+[K_1]})$
pH vs $CO_3^{2-}$ Speciation = $LOG(\frac{[K_2][HCO_3^-]}{[H^+]})$
pH vs $H_2CO_3$ Speciation = $LOG(\frac{[K_1][H_2CO_3^2]}{[H^+]})$ for pH 0 - 8.2 and $LOG(\frac{[H^+][CO_3^2]}{[H^+]+[K_2]})$ for pH 8.2 - 14
I don't understand what's going on and have spent DAYS trying to figure out why and where I've gone wrong... please help!
I've also attached a picture from the previous page of the book for context!