5
$\begingroup$

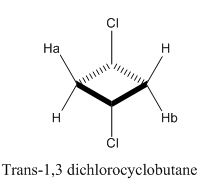
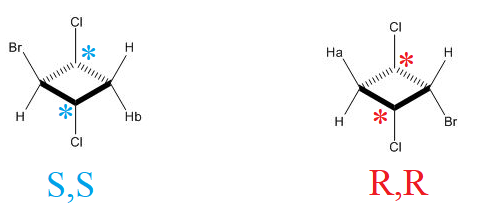
The question is to find the TOPICITY relationship between Ha and Hb in the given compound, (trans 1,3 dichlorocyclobutane) enter image description here

They seem to be in the same chemical environment, so I tried the substitution method, and replaced Ha and Hb with an X* atom in-turn (for convenience, I have used Br as the X* atom ). Then, I used CIP rules to assign priority, and got RR and SS configuration respectively. (See img below) I concluded them to be enantiotopic.

However, I am still confused because I cannot imagine any plane which would be used to show the mirror image relationship between the two.

enter image description here enter image description here

Is my answer and reasoning correct?

If so, kindly demonstrate the mirror plane which makes the two compounds enantiomers.

If not, please explain.

Nb: I couldn't find an axis of symmetry that makes Ha and Hb equivalent. That led me to the conclusion that thay are not Homotopic.

Improve this question
$\endgroup$
2
  • $\begingroup$ I think you're overthinking the substitution method. Remove the a and b labels on the two hydrogen atoms. Then, one at a time, replace each previously labelled hydrogen with H*. How are the two molecules, which have no a/b labels and a single starred hydrogen each, related to each other? That will tell you the stereotopic relationship between the hydrogen atoms. $\endgroup$
    – Zhe
    Commented Apr 5, 2022 at 13:42
  • $\begingroup$ @Zhe I have labelled Ha and Hb just for convenience. I completely understand your point that they are the same. Still I couldn't figure out the answer, until I put forward this question to my professor. However, thanks for pointing out that info to the readers. $\endgroup$
    – Cyclopropanol
    Commented Apr 15, 2022 at 12:29

3 Answers 3

Reset to default
4
$\begingroup$

They are ENANTIOTOPIC.

According to IUPAC Gold Book,

Atoms or groups of a molecule which are related by an n-fold rotation axis (n = 2, 3, etc.) are called homotopic

In the given question, Ha and Hb are not related by an axis of rotation. However, they are related by a center of symmetry. Therefore, they are enentiotopic protons. The following chart is helpful.

enter image description here

Note: Enantiotopic protons are indistinguishable through NMR spectroscopy.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ 10 months later, we have a correct answer... $\endgroup$
    – Karsten ♦
    Commented Feb 18 at 15:05
1
$\begingroup$

What is curious about the bromine-substituted molecule is that it does not matter whether the bromine "points up" or "points down", it is the same molecule:

enter image description here

For example, the top left and the top center molecules are identical, related by a two-fold rotation about the X-axis (which goes from left to right).

[OP] If so, kindly demonstrate the mirror plane which makes the two compounds enantiomers.

The two circled structures correspond to the molecules and orientations shown in the question. As Cyclopropanol's answer states, they are related by a center of inversion. A center of inversion can be realized by a two-fold rotation combined with a mirror plane. The structures on top and on the bottom are related by a mirror plane (in the plane of the paper). So you can take the top circled structure, rotate it 180 degrees about the Z-axis (perpendicular to paper) to arrive at the top right structure, and then apply the mirror plane to get the circled structure on the bottom. Or you can first apply the mirror operation and then the 180 degree rotation.

If you prefer a mirror plane swapping left and right, compare the top left structure with the bottom right structure.

[OP] Is my answer and reasoning correct?

Yes, because the two bromine-substituted structures are enantiomers, the hydrogen atoms are enantiotopic (indistinguishable in the NMR unless you add a chiral co-solvent). I was surprised that you did not label the stereochemistry of the bromine-substituted carbon, but now I understand why (not a chiral center).

Improve this answer
$\endgroup$
1
$\begingroup$

Geometrically, the relationship between $\ce{H_a}$ and $\ce{H_b}$ is through a center of inversion. This is the same as a mirror reflection combined with a $180°$ rotation. Even without an explicit mirror plane, the implicit reflection component associated with a center of inversion is sufficient to distinguish enantiotopicity from homotopicity.

You may want to perform an excercise involving a simple chiral molecule, let us say 2-butanol. Initially place the substituents around the stereocentric carbon so that they have the R configuration. Thus when the molecule is viewed with the lowest-ranking hydrogen atom pointing away from you, the ranking of the other substituents ($\ce{OH > C2H5 > CH3}$) corresponds to a clockwise rotation. Now, instead of reflecting the molecule in a mirror, invert all the substituents through the stereocentric carbon. You must now rotate the molecule so as to get the lowest-ranking hydrogen back to the bottom, and this causes one of the other substituents to slip between the remaining two. The $\ce{OH > C2H5 > CH3}$ order has thereby become counterclockwise; the central inversion acts like a mirror reflection by inverting the chiral orientation.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.