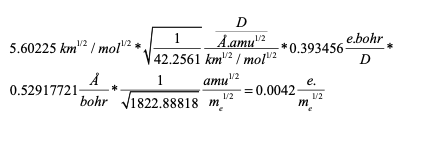0
$\begingroup$

I need to convert dipole derivative output by Gaussian into atomic units. The dipole derivative is given in Gaussian in the weird units $(\mathrm{km/mol})^{1/2}$. I know that

$\pu{1 D^2/(Å^2amu)}$ = $\pu{42.2561 km/mol}$

$\pu{1 D(ebye)= 0.393456 bohr \textrm{-} e}$

$\pu{1 bohr = 0.529 Å}$

$\pu{1 amu = 1822.8818 electron masses}$

I made the conversion (see below), but the value I get is in an improbable range (I know that the answer should be roughly 0.15 or in that order of magnitude based on literature). Is there anything wrong with the unit conversion below? I realize this is a rather dumb question but I would really appreciate if someone could point out any silly mistake I may have made.

enter image description here

Improve this question
$\endgroup$
7
  • 3
    $\begingroup$ Please visit this page, this page and this one on how to format your posts better with MathJax and Markdown. Note that symbol "amu" is deprecated since 1961. $\endgroup$
    – andselisk ♦
    Jan 22, 2022 at 23:15
  • 1
    $\begingroup$ I guess it's a particular kind of funny to call SI units weird. Indeed, it's weird in that context as Gaussian usually adheres to ancient systems of measurement. $\endgroup$
    – Martin - マーチン ♦
    Jan 22, 2022 at 23:29
  • 1
    $\begingroup$ thank you for the suggestions and comments. Gaussian units are indeed weird, if that was your meaning. but can you answer my question or not? In general, I don't get why rather than answering the question, people feel the need to comment on presentation and other superficial things. If you had time to comment, you had time to answer. $\endgroup$
    – poisonDartFrog
    Jan 23, 2022 at 0:51
  • 1
    $\begingroup$ Presentation isn't merely superficial. If folks want to check your work, they want to be able to copy and paste it into whatever program they use. They don't want to have copy it by hand, which is what happens when you put formulas in screenshots. Plus screenshots aren't searchable. Also, the way you wrote the eqns. in your 1st para. makes them less readable. As to the substance of your post, I'm not sure how you get D^2/(Å^2.amu)=42.2561 km/mol. I get D^2/(Å^2.amu) = 6.70 x 10^–13 s^2 amperes^2/kg = 2.61 x 10^25 e^2/kg, where e is an elementary charge.[Informal formatting is OK in comments.] $\endgroup$
    – theorist
    Jan 23, 2022 at 8:24
  • 2
    $\begingroup$ It seems that conversion is designed to account for the non-std units of the Gaussian output. Anyways, this should help: mattermodeling.stackexchange.com/questions/5021/… According to the answer there by Jon Kragskow, "To get the true derivatives in $[\text{D Å}^{-1}\text{u}^{-1/2}]$, you need to divide the reported derivatives in $[\text{km}^{1/2}\text{ mol}^{-1/2}]$ by $\sqrt{42.2561} \text{ km}^{1/2}\text{ mol}^{-1/2} \text{u }^{1/2} Å \text{ D}^{-1}$." So based on that, I get $ 0.862 \text{ D Å}^{-1}\text{u}^{-1/2}$ $\endgroup$
    – theorist
    Jan 23, 2022 at 9:15

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Browse other questions tagged or ask your own question.