I came across this question which I initially ignored believing that the data was insufficient:
Calculate the pressure exerted by one mole of $\ce{CO2}$ gas at $\pu{273 K}$ if the van der Waals' constant $a = \pu{3.592 dm^6 atm mol^{-2}}$. Assume that the volume occupied by $\ce{CO2}$ molecules is negligible.
I saw that I had 2 variables $P$ and $V$ and logically from what I'd dealt with with real gases I concluded that there must not be a unique solution, but the answer (which can be incorrect) mentioned a unique value of $P$.
I tried solving by writing the equation of state:
$$\left(P+\frac{a} {V_\mathrm{m}^2}\right)V_\mathrm{m} = RT$$
By writing it as a quadratic in V we get
$$PV_\mathrm{m}^2-V_\mathrm{m}RT+a=0$$
The discriminant of this quadratic is $D = R^2T^2 - 4aP$.
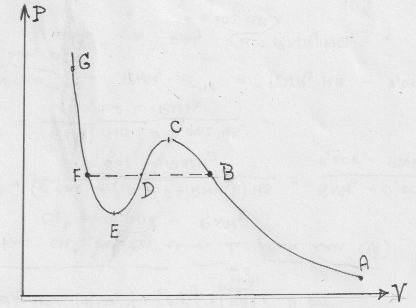
This implies that not all pressures are feasible since $D<0$ for some values of $P$. But for most other values of $P$, $D>0$ which implies that there are 2 values of $V_\mathrm{m}$ for a certain $P$. But looking at the compressibility factor ($Z$) vs $P$ graphs for various gases I found that for a certain $P$ there was only one $Z$ at a temperature, which means there should be only one solution? So the only possibility is that $D=0$ which yields a fixed value of $P$ and hence a fixed value of $V_\mathrm{m}$. That gave me the right answer.
But that seems really wrong since temperature alone shouldn't be able to dictate a unique state and that also implies that volume or a real gas is independent of the volume of container which sounds terribly wrong.
I strongly feel $D=0$ caused this but I don't see why not.
I would like to observe only the gaseous state, i.e, set the temperature above the Critical Temperature.