Considering Gibbs energy or electrode potential to find disproportionation tendency

Question

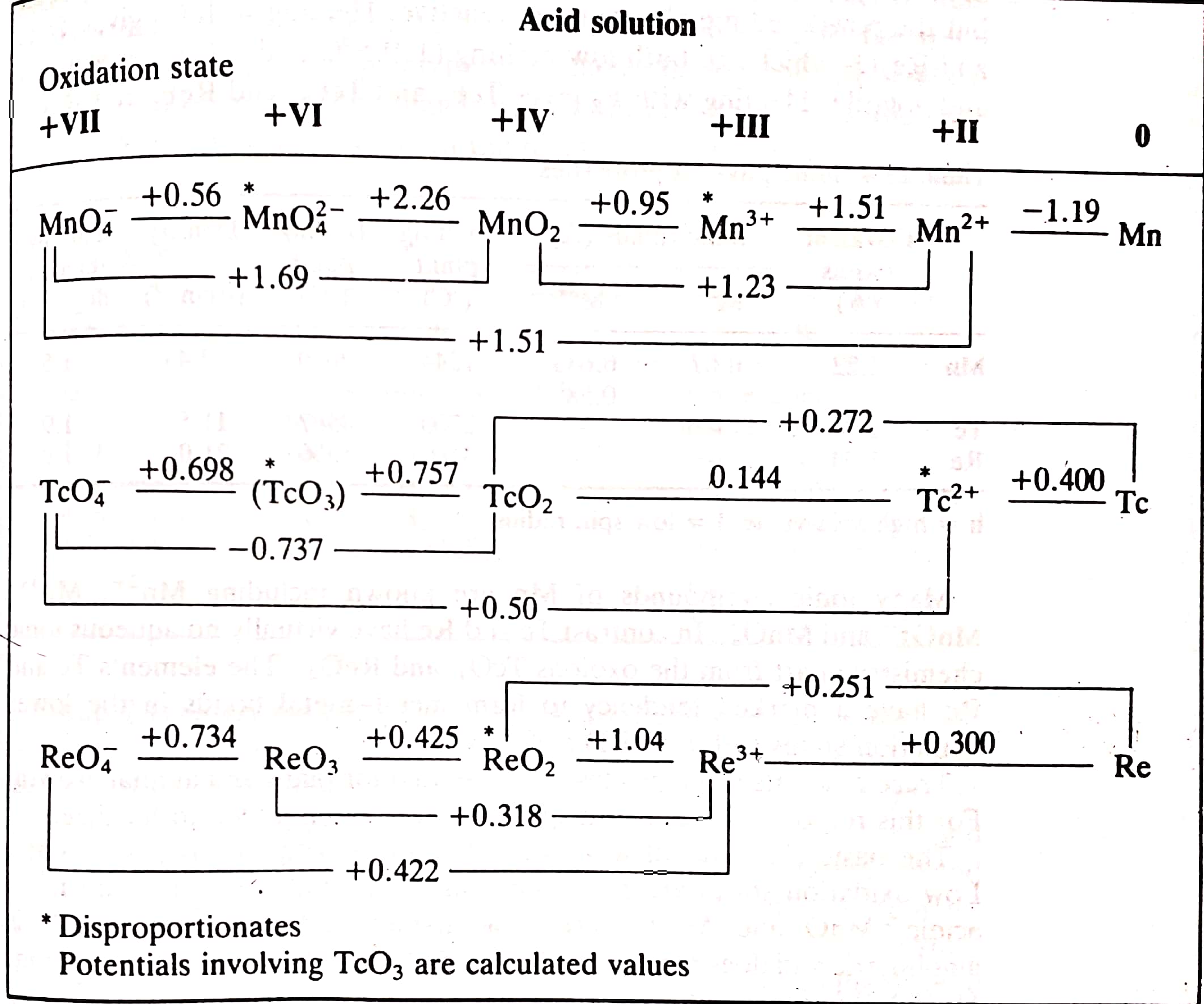
The following data was given (without the asterisks and footnote) asking us to find the oxidation states that would have a tendency to disproportionate -

By tendency to disproportionate, I understood that the disproportionation reaction must be spontaneous, implying change in Gibbs free energy should be negative. As it is related to the given electrode potentials as $\Delta G = -nFE$, I proceeded by solving $\Sigma nE$ should be positive. (I didn't forget to consider the fact that all potentials are reduction potentials, and for disproportionation the oxidation half would have its sign reversed).

However, my teacher later told that in such questions one simply considers the sum $\Sigma E$ and not $\Sigma nE$, for tendency to disporportionate. From the given data, for each such species both $\Sigma E$ and $\Sigma nE$ are positive, so I cannot infer from it whether my approach was correct or my teacher's. Please clarify which method is correct, and in case $\Sigma E$ is correct, then why $\Sigma nE$ doesn't work.

An example of my approach - Consider $\ce{MnO4^2-}$ For its disproportionation, reduction potential for reducing to $\ce{MnO2}$ = $\ce{E1}$ = 2.26 and number of electrons = $\ce{n1}$ = 2. Oxidation potential = $\ce{E2}$ = -0.56 and number of electrons = $\ce{n2}$ = 1. Then the sum $\Sigma nE$ = $\ce{E1n1 + E2n2}$ = 3.96 which is positive. So $\Delta G$ is negative, hence the disproportionation is spontaneous.

My teacher told that $\Sigma E$ = $\ce{E1 + E2}$ = 1.70 is positive, would suffice.

(Normally I would never doubt my teacher, but she didn't reply when I messaged her regarding why my method was wrong, so I still need an explanation.)

Answer 1

Your teacher is correct. I will take the example that you gave in the question. You wish to analyze the reaction: $$3\ce{MnO4^{2-}}\to 2\ce{MnO4^{-}}+\ce{MnO2}...(1)$$ This reaction can be split into two parts, the oxidation half reaction, which forms the anode: $$\ce{MnO4^{2-}}\to \ce{MnO4^{-}}+\ce{e-}...(2)$$ And the reduction half-cell, which forms cathode: $$\ce{MnO4^{2-}} +2\ce{e-}\to \ce{MnO2}...(3)$$ Notice that Gibb's free energy is an extensive property, and hence is additive. So, if original reaction has $\Delta G_1$, since: $$(1)=2×(2)+(3)$$ We have: $$\Delta G_1=2×\Delta G_2+\Delta G_3$$ Which means: $$-\Delta G_1=2×(1×F×E_2)+1×(2×F×E_3)$$ This means that checking the sign of $E_2+E_3$ is sufficient to conclude if $\Delta G_1$ is positive or negative, taking the sign of $E_2$ into consideration, of course. In general, we have the formula: $$E^{°}_{cell}=SRP_{cathode}-SRP_{anode}$$ which could have been used to conclude the same.