Compressibility factor and deviation from ideality

Question

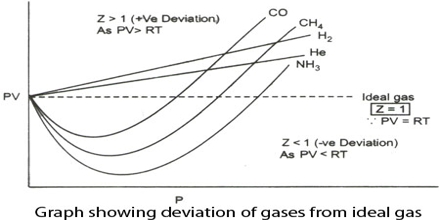
Consider these statements :

1. At Boyle point, real gases show ideal behaviour.
2. Above the Boyle point, real gases show positive deviation from ideality (forces of attraction between the particles are very feeble).
3. Below Boyle point, real gases first show decrease in Z value with increasing pressure, reaches a minimum value, increases again on further increase in pressure.

Questions:

1. What is the actual significance of Z ? (I mean, intuitively )
2. What is meant by positive deviation from ideality? If this is a measure of how Z value increases, I don't get it.

Ideal gases do not have forces of attraction between the constituent particles.

If we consider Z as:

V(real)/V(ideal),

how can a real gas show a positive deviation from ideality? ie. How can a real gas with a small amount of intermolecular force of attraction expand more than an ideal gas with zero intermolecular force of attraction?

3. What is meant by negative deviation from ideality? What happens to a ideal gas when we apply pressure to it? Will we be able to compress it indefinitely But won't there be repulsive interactions even in such gases due to decrease in stability when huge amount of pressure is applied?

Any answer would be greatly appreciated.

Answer 1

Let's start thinking qualitatively.

1. What we call "volume" of a gas is the sum of the "free" volume where the molecules are free to move, plus the volume of the molecules themselves. The collision theory is only valid for a volume which is the free space available to the moving molecules.

2. What we call "pressure" of a gas is the force exerted by the particules when touching the walls of the container. But this force in a compressed gas is not simply due to its kinetic energy due to the temperature. It is diminished if there is an attraction between the particules.

In a gas like $\ce{H2}$, there is practically no attraction between individual molecules. The pressure is due to the collisions with the walls due to particules whose energy is simply proportional to $\pu{T}$. So the total volume is equal to the theoretical value $\ce{V_o = nRT/V}$, plus the volume of the molecules. It increases with the pressure. So the measured $\pu{V}$ is greater than $\pu{nRT/p}$ and $\pu{Z = pV/nRT}$ increases with $\pu{p}$.

In a gas like $\ce{CO2}$ or $\ce{CO}$, the pressure measured on the wall is smaller than the theoretical values coming from the kinetic theory of gases, because the measured pressure is slowed down by their mutual attraction. That is why $\pu{Z = pV/nRT}$ decreased by increasing the pressure

Answer 2

What @Poutnik meant was as follows:

An ideal gas is considered to have very negligible or almost no volume of the molecules it is comprised of. It also has, as you say, zero interaction energy.

But the change in volume of the real gas depends on which of the two factors overpowers the other: (1) Real gas molecules have certain volume (2) Interaction Energy is non zero in between real gas molecules.

Not the volume of an individual molecule but the interaction energy changes with changing parameters of pressure and temperature (Implying that as we change temperature and pressure, the interaction between the molecules is affected). Thus Z is a measure which takes into consideration these factors (pressure, temperature and volume). Intuitively speaking in simple terms, it literally stands by its name: 'Compressibility factor'. If Z<1, then for a fixed amount of pressure applied, a real gas will be better compressed than an ideal gas. Implying that if Z<1 then gas is more compressible (than what it would have been as an ideal gas). On similar lines you can understand what Z>1 means.

Now Z is formulated as Z = PV/RT

Look at the graph above. It shows various gases showing deviation from ideality. The picture is pretty much self explanatory.

Boyles Point/Temperature is a specific temperature for each gas slightly around and at which the gas tends to behave almost ideally. Now this must tell you that the ideality of a gas is also temperature dependent. Boyles Temperature is estimated by

T=a/Rb

where R is universal gas constant; b is the volume excluded(unoccupied) by 1 mole of the gas from the volume if the gas has been ideal; value of 'a' depends upon the attractive forces between the molecules and is directly proportional to it (Thus how the molecules behaves in terms of interacting with fellow molecules is also considered) So now you can see how all the factors play an altogether role in the gas behavior.

[P.S. I wanted to post this in a brief manner in the comment section but couldn't fit in. All required edits are open. I really tried to put it into simple words]