2
$\begingroup$

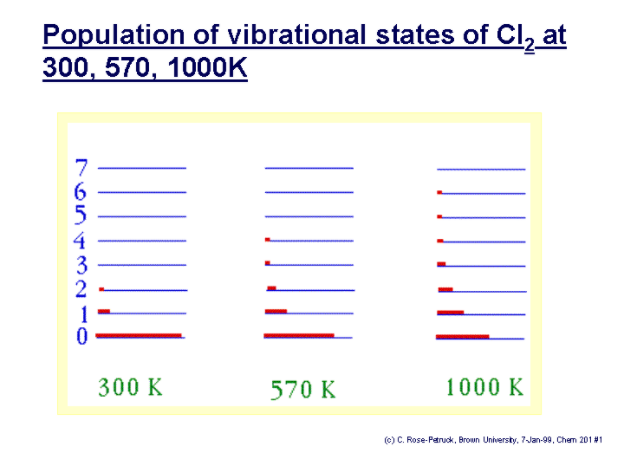
We know that molecules have different energy states (vibrational, rotational, electronic). Using Boltzmann distribution we can find the popoulation of each energy state. If we have only electronic states (e.g. atoms) then we can find the population of the ground, first excited state etc. If we have a molecule (neglecting rotational levels) will the population depend on the population of the electronic state? For example in this diagram Vibrational levels distribution:

                                enter image description here

will the population ($\%$) also depend in which electronic state the distribution is evaluated?

I think that the percentages would not change. For example the ground electronic state in $υ=0$ vibrational state would have the same population with the excited electronic state in $υ=0$ vibrational state. The percentages will be the same while the number of molecules in each state will be different, e.g. in ground state with $υ=0$ we will have $100$ molecules while in the excited state with $υ=0$ we will have $30$ (numbers are just random). Otherwise it wouldn't make sense to say that at low temperatures the most populated electronic state is the ground state.

Improve this question
$\endgroup$
4
  • 3
    $\begingroup$ Well, to be precise one should consider combined electronic-vibrational states over which to perform the Boltzmann distribution calculation. (These are basically products of the electronic and vibrational states, although note that the different electronic states will also have different vibrational spacings, since the bond strength / force constant will be changed by electronic excitation.) But at room temperature, the population of excited electronic states is essentially zero. $\endgroup$
    – orthocresol
    Commented Feb 19, 2021 at 20:46
  • 1
    $\begingroup$ The vibrational frequency and hence energy spacings will generally be different in the ground state compared to bound electronically excited states due to different bonding after electronic excitation. Hence the populations of these levels will be different to the ground state at the same temperature. Of course you have to produce the excited state first and it has to live long enough for thermal equilibrium via collisions with inert species to be produced. $\endgroup$
    – porphyrin
    Commented Feb 20, 2021 at 9:13
  • $\begingroup$ Until "Otherwise it wouldn't make sense to say that at low temperatures the most populated electronic state is the ground state." I follow your reasoning. It does not really matter as we do not normally deal with absorption from higher vib states in the el ground states, thus even less with those within an excited one. Porphyrin/orthocresol (note the heterogeneous phases :)) more or less answered to your question. However, the last sentence that seems to pose trouble, is right the answer. At low T there are no molecules in the excited state, thus no need for looking at the corresponding vib $\endgroup$
    – Alchimista
    Commented Feb 20, 2021 at 13:02
  • $\begingroup$ In the case we want to include translational energy should we treat it as rotational energy with smaller seperation between the energy levels? $\endgroup$
    – Anton
    Commented Aug 29, 2021 at 11:43

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.