How does the pyramidalization effect the bonding angles in a distorted octahedral environment?

Question

This is a follow up on "Generally Octahedral - Meaning?".

Since Dissenter asked in the comments to my answer:

I'm not sure why the $\ce{F_{eq}-S-F'_{eq}}$ bond angles would be compressed, even if the $\ce{S}$ were out of the plane.

Since ron's answer already satisfies the original question about $\ce{BrSF5}$ and I did not want to clutter my answer even further, I am asking it here in a Q&A style.

How does the pyramidalization effect the bonding angles in a distorted octahedral environment?

Answer 1

So we are again talking about $\ce{BrSF5}$ but this post extends to all generally octahedral complexes/ molecules and even quadratic planar geometries. The main requirement is, that the molecule has a $C_{\mathrm{4v}}$ point group. (It may be extended to all non-linear $C_{n\mathrm{v}}\ (n>1)$ point groups.) First of all reconsider the molecular shape again.

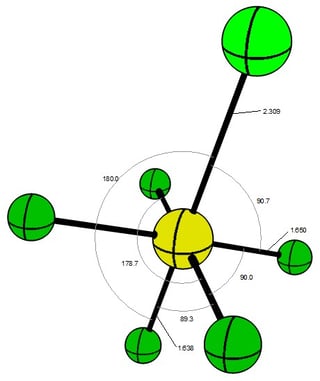
Now admittedly the degree of pyramidalization is more than minimal, with less than $1^\circ$ deviation from the plane. However, this has a slight effect on the equatorial $\angle(\ce{F-S-F'})$ bond angle, purely to symmetric reasons.

Basic trigonometric considerations are enough to understand this phenomena. Consider the following scheme, where the peak of the pyramid is represented by $\mathbf{\ce{Y}}=\ce{S}$ and the base is span by $\mathbf{\ce{X_{eq}}}=\ce{F_{eq}}$, $\mathbf{\ce{X'_{eq}}}=\ce{F'_{eq}}$, $\mathbf{\ce{X''_{eq}}}=\ce{F''_{eq}}$ and for completeness $\mathbf{\ce{X'''_{eq}}}=\ce{F'''_{eq}}$.

So we have some basic quantities, that we can address, but in the end we can derive the connection of the two angles without caring about the other quantities, only knowing the point group.

• $a$ is the $\mathbf{d}(\ce{F_{eq}\cdots{}F'_{eq}})$ distance
• $d$ is the $\mathbf{d}(\ce{F_{eq}\cdots{}F''_{eq}})$ distance
• $s$ is the $\mathbf{d}(\ce{S\cdots{}F_{eq}})$ distance
• $h$ is the height of the pyramid and it refers to the out of plane shift of $\ce{S}$ compared to the $\ce{F_{eq}\cdots{}F'_{eq}\cdots{}F''_{eq}\cdots{}F'''_{eq}}$ plane
• $h'$ is another virtual quantity, the angle bisector of $\angle(\ce{F_{eq}-S-F'_{eq}})$
• $\alpha$ refers to the bonding angle $\angle(\ce{F_{eq}-S-F''_{eq}})$
• $\beta$ refers to the bonding angle $\angle(\ce{F_{eq}-S-F'_{eq}})$

From Pythagoras's Theorem we can easily derive the following connection (square in the scheme): \begin{align} && d^2 &= a^2 + a^2\\ \therefore && d &= \sqrt{2}\cdot a\tag{1} \end{align}

So now we are going to use some trigonometric functions for the angles (look at the triangles): \begin{align} && \sin\left(\frac{\alpha}{2}\right) &= \frac{\frac12d}{s}\\ \therefore && s &= \frac{\frac12d}{\sin\left(\frac{\alpha}{2}\right)}\tag{2} \end{align}

\begin{align} && \sin\left(\frac{\beta}{2}\right) &= \frac{\frac12a}{s}\\ \therefore && s &= \frac{\frac12a}{\sin\left(\frac{\beta}{2}\right)}\tag{3} \end{align}

Let's derive the connection between the angles by equalizing $(2)$ and $(3)$: \begin{align} && \frac{\frac12d}{\sin\left(\frac{\alpha}{2}\right)} &= \frac{\frac12a}{\sin\left(\frac{\beta}{2}\right)}\\ \therefore && \sin\left(\frac{\beta}{2}\right) &= \frac{a}{d}\sin\left(\frac{\alpha}{2}\right)\\ \text{with (1)}\implies && \sin\left(\frac{\beta}{2}\right) &= \frac{1}{\sqrt{2}}\sin\left(\frac{\alpha}{2}\right)\\ \equiv && \sqrt{2} &= \frac{\sin\left(\frac{\alpha}{2}\right)}{\sin\left(\frac{\beta}{2}\right)}\tag{4} \end{align}

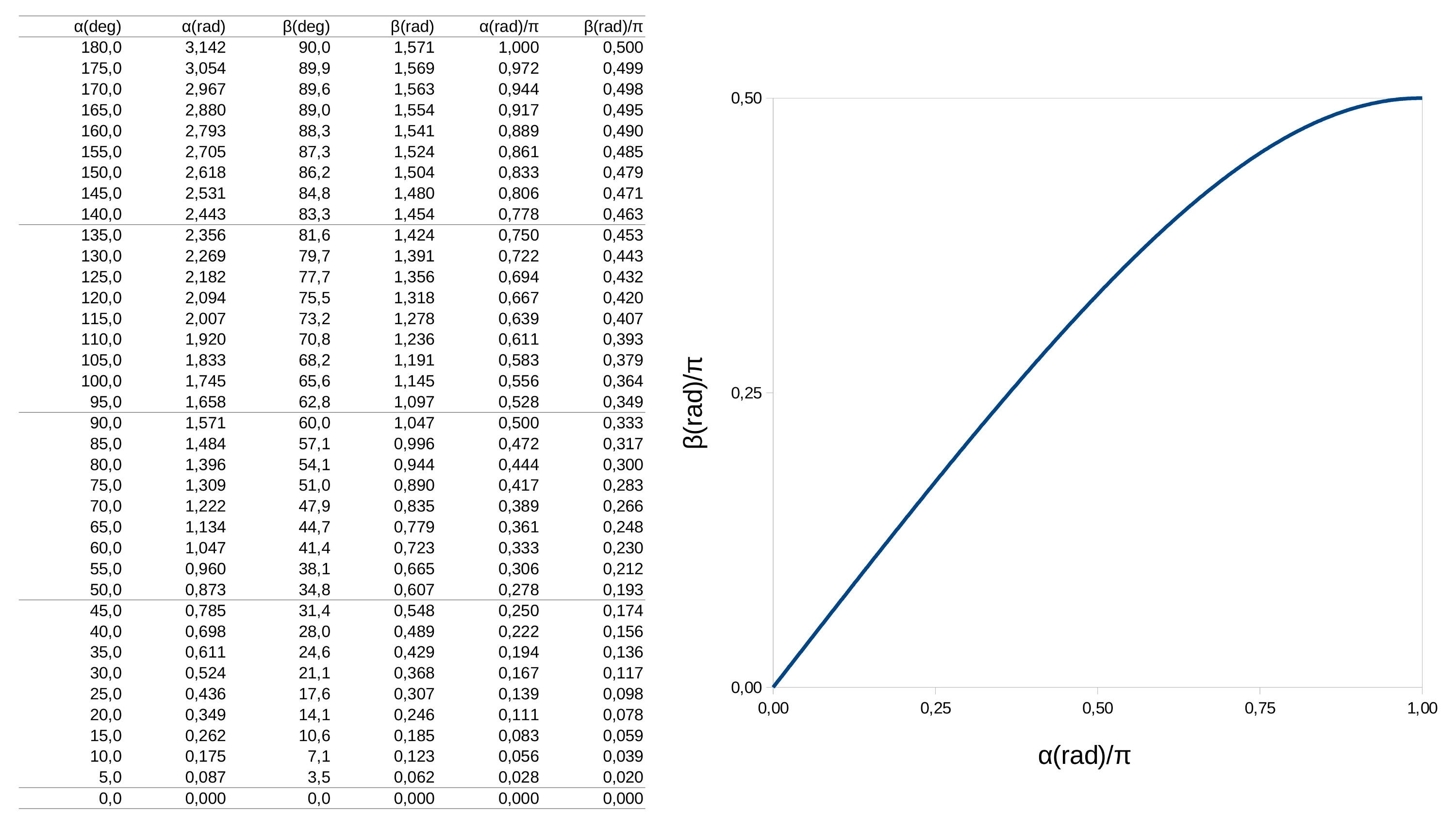
From this you can see, that the degree of pyramidalization is codependent on the equatorial bond angles. When the pyramidalization increases, $\alpha\to0$, then the equatorial bond angle has to decrease, $\beta\to0$, i.e. a constant ratio.

In the special case of $\alpha = 180^\circ$ this simplifies to $\beta=90^\circ$. In all other cases you can derive the degree of pyramidalization from the equatorial bond angles and vice versa.

Transformation of $(4)$ yields a function that displays the equatorial bond angle in dependence of the pyramidalization, with $\alpha\in~]0^\circ;180^\circ]$ or $\alpha\in~]0;\pi]$. $$\beta = 2\cdot\arcsin\left[\frac{1}{\sqrt{2}}\cdot\sin\left(\frac{\alpha}{2}\right)\right]$$

---

Addendum

The out of plane height can also be derived from Pythagoras's theorem (look at right triangle): \begin{aligned} && h^2 &= s^2 + \left(\frac{d}{2}\right)^2\\ \therefore && h &= \sqrt{s^2 + \left(\frac{d}{2}\right)^2}\\ \text{with (1)}&& h &= \sqrt{s^2 + \frac{1}{2}a^2}\\ \end{aligned}

This is usually a value that is given for domed complexes, so it is nice to know how to find it.