How do I find miller indices for a plane whose intercepts are fractions of the lattice constant?

Question

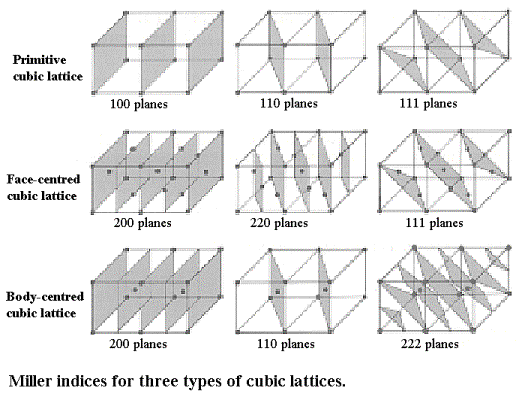
[I'm talking with respect to cubic lattices alone.]

For instance, if a plane has $x,y,z$ intercepts $a/2,a/2,a/2$ (where $a$ is the lattice constant) the miller index would be $[2\space2\space2]$. The book I'm referring to says that for fractional intercepts, the indices do not have to be reduced to the smallest whole numbers(hence $[2\space2\space2]$).

But, miller indices are supposed to represent a set of parallel planes and I can't think of any other plane with the index $[2\space2\space2]$ Then I saw this:

The image(3rd row, 3rd image) shows the set of $[2\space2\space2]$ planes. I'm guessing that one of them has intercepts $a/2,a/2,a/2$. What are the intercepts of the other planes, and how are they all $[2\space2\space2]$?

Or, if my method of calculation is wrong, how else do I calculate miller indices for fractional intercepts?

PS: I've only just started to learn this concept, so it's possible that my understanding of miller indices is fundamentally flawed.

Answer 1

The plane closest to the origin has the intercepts you mentioned. The rest have intercepts that are integer multiples of this. In the figure, the origin is on the bottom left of each cell. As the figure shows, the [1 1 1] planes correspond to every second of the [2 2 2] planes because the latter is a multiple (by 2) of the former.

Higher indeces are associated with a lower distance of the planes and with a higher resolution (i.e. larger Bragg angle) of the corresponding reflection.