3
$\begingroup$

I am trying to understand the International Tables for Crystallography.

How can I identify the origin in the image on the left?

Without knowing the origin, I cannot identify the position of the symmetry elements. In the International Tables for Crystallography (Vol. A) it appears that the origin is at the "$(\overline{3}$ m 1) at $\overline{3}$ 2/m c" position. What does the $(\overline{3}$ m 1) element and the $\overline{3}$ 2/m c element mean? Where is the reference center really located?

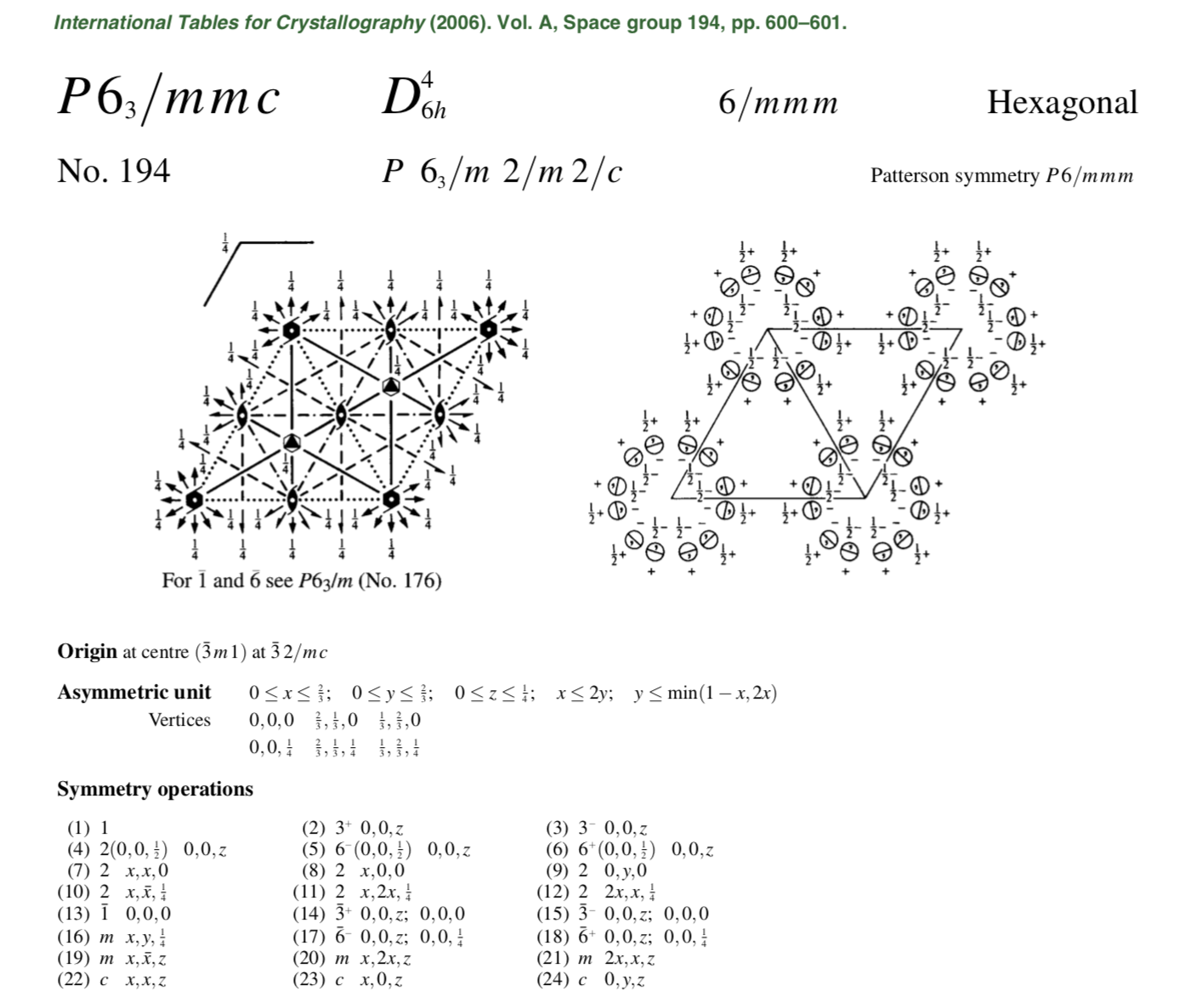
enter image description here

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ You picked a fairly difficult one. I'd suggest starting with some of the more symmetric ones and parsing from there. The times I've used the tables it took a few exercises to build up from some of the simple cubic-type lattices to the ones I wanted. $\endgroup$
    – Jon Custer
    Commented May 13, 2020 at 22:44
  • $\begingroup$ @JonCuster Thanks for the reply. I've seen some simpler ones and I wanted to know the origin of this to confirm my guess. I think the origin is the $6_3$ axis that is in the upper left corner, at a vertex. That's right? Why do two positions appear: one with parentheses and one without parentheses? $\endgroup$
    – Jose Marin
    Commented May 14, 2020 at 1:38

1 Answer 1

Reset to default
5
$\begingroup$

For centro-symmetric space groups such as this one, the origin coincides with the center of inversion. You can see this specifically for this group if you look at symmetry operation (13): $$\overline{1} \mathrm{\ at\ } 0,0,0$$ Of course, there an an infinite number of origins in an infinite lattice because of the translational symmetry. In the tables, the origin is in the plane of the paper on any of the unit cell vertices shown, so upper left corner would be fine.

$(\overline{3}\ m\ 1)$ is a complicated way of saying inversion center. "$\overline{3}$" points at us, "$m$" is along the axes b or c, and "1" is the third direction (in the a b plane, 30 degrees rotated away from a or b or a+b). The next set of three symmetry elements $\overline{3}\ 2/m\ c$ are also in those three directions, and tell us how they are oriented with respect to the origin (they all go through it). The table listing all symmetry operations (and the list of transformations of a coordinate $x, y, z$ given here) confirms this.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ There are a few centro-symmetric space groups with multiple settings; for those, the second setting has the inversion center at the origin. The space group in question is not one of those, though. $\endgroup$
    – Karsten ♦
    Commented May 14, 2020 at 15:35
  • $\begingroup$ Thanks for the reply. Although you have explained, I did not understand why two directions appear (one with parentheses and one without parentheses). I didn't understand what you meant by "how they are oriented with respect to the origin". Could you explain otherwise please? When you say that 1 in $\overline{3}\,m\,1$ refers to the diagonal, is the diagonal you consider to be [1 1 0] or are you considering the bisector: [1 -1 0]? The direction $\overline{3}\,m\,1$ belongs to the trigonal group, so I am a little confused with the choice of axes for each index. $\endgroup$
    – Jose Marin
    Commented May 14, 2020 at 16:44
  • 1
    $\begingroup$ @JoseMarin "how they are oriented with respect to the origin": A two-fold axis along c could go through the origin (i.e. 0 0 z) or not (i.e. 1/2 0 z or 1/2 1/2 z). Same for a mirror plane (e.g. 0 -y -z would be through the origin, and 1/4 -y -z would not). As for the exact formatting on the statement about the origin (i.e. with/without parentheses), I don't know. The third direction (I misleadingly called it diagonal) is 30 degr rotated from a, b or the bisector. The directions a, b, and bisector are all equivalent because of the 6-fold screw axis, so the third direction is not any of those. $\endgroup$
    – Karsten ♦
    Commented May 14, 2020 at 17:28
  • $\begingroup$ So in $\overline{3}\,m\,1$, $\overline{3}$ is parallel to z, m is perpendicular to a, b, or diagonal: a+b ([1 1 0]) and 1 (identity element) is the direction that makes 30º with a, b or diagonal (a+b) axis, right? I don't understand the part of indexes with or without parentheses. In the case of the axis of rotation, you are right, it is necessary to say the axis for which it is parallel and the point of application. But in inversion center, it is usually indicated the position where it is, because in this case, as it is not an axis, I don't understand why 2 sets of indexes are needed. $\endgroup$
    – Jose Marin
    Commented May 14, 2020 at 17:50
  • 1
    $\begingroup$ @JoseMarin I don't think 2 sets of indices are needed. It would suffice to say that the origin is at the center of symmetry (but this does not appear in the short or long form of the space group symbol). I suspect this is a quick way of making a statement about the relative location of the symmetry elements, but as I said, just a guess. $\endgroup$
    – Karsten ♦
    Commented May 14, 2020 at 18:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.