The atomic masses of gold and platinum are 196.96657 u and 195.084 u respectively, meaning that (on average) an individual gold atom is heavier than an individual platinum atom.
At the same time, the van der Waals radius of gold is 166 pm, whereas that of platinum is 175 pm. So an individual gold atom is smaller than an individual platinum atom.
Given these data, how is it possible that platinum metal can actually be denser than gold (21.5 g/cm3 vs 19.3 g/cm3)?
Since OP is still in the high school, I'll try to explain it simply as possible using mathematical manipulation (hoping OP is more familiar with mathematics than chemistry).
Both gold and platinum consist of same crystal packing called body-centered cubic, which is illustrated in following diagram:
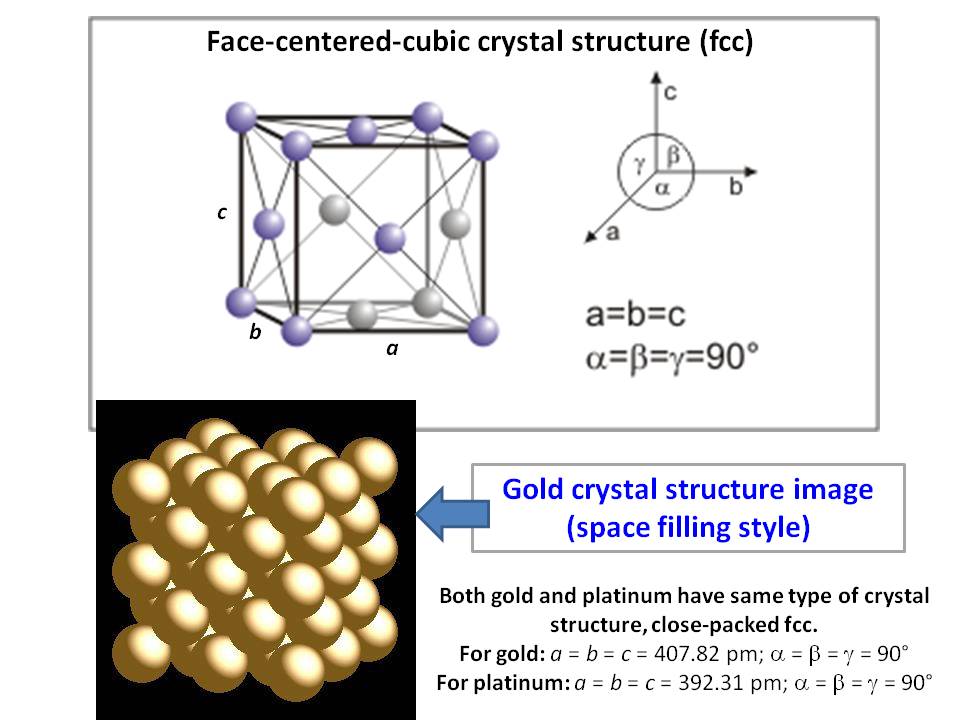
Crystal studies of both gold and platinum has revealed that the lengths of their cubic unit cells ($a$) are $\pu{407.82 pm}$ and $\pu{392.31 pm}$, respectively. If you inspect closely, you would realize that each of eight corner atoms shares with eight unit cells while each of six face-centered atoms shares with only two unit cells. Thus, total atoms per unit cell $= 8 \times \frac{1}{8} + 6 \times \frac{1}{2} = 4$. Keep in mind that $a$ is depend on the atomic radius of each metal and how closely they packed against each other against some concerning forces (e.g., van der Waal's). For example, the experimental finding has been reported that the closest $\ce{Au-Au}$ separation is $\pu{288.4 pm}$ while that of $\ce{Pt-Pt}$ is $\pu{277.5 pm}$. These values imply that the metallic radius of gold is $\pu{144.2 pm}$ and that of platinum is $\pu{139 pm}$. This would cover the quote "the radii of the atoms and the bond lengths within the crystal lattice."
Based on the value of $a$ and number of atoms per unit cell for each metal, you can calculate the molar volume of each metal ($V_\mathrm{Metal}$):
$$V_\ce{Au} = a_\ce{Au}^3 \times \frac{1}{\pu{4 atoms}} \times N_A \\ = (\pu{407.82 pm})^3 \times \left(\frac{\pu{1 cm}}{\pu{10^10 pm}}\right)^3 \times \frac{1}{\pu{4 atoms}} \times \pu{6.022 \times 10^{23} atoms\:mol-1} = \pu{10.21 cm3mol-1}$$
and similarly,
$$V_\ce{Pt} = a_\ce{Pt}^3 \times \frac{1}{\pu{4 atoms}} \times N_A \\ = (\pu{392.31 pm})^3 \times \left(\frac{\pu{1 cm}}{\pu{10^10 pm}}\right)^3 \times \frac{1}{\pu{4 atoms}} \times \pu{6.022 \times 10^{23} atoms\:mol-1} = \pu{9.09 cm3mol-1}$$
Once we know the molar volume of each metal, we can calculate the density ($d$) by using $d=\frac{\text {molar mass}}{\text {molar volume}}$:
$$d_\ce{Au} = \frac{\pu{196.97 gmol-1}}{\pu{10.21 cm3mol-1}} = \pu{19.29 gcm-3}$$
$$d_\ce{Pt} = \frac{\pu{195.08 gmol-1}}{\pu{9.09 cm3mol-1}} = \pu{21.46 gcm-3}$$
Therefore, it is true that $d_\ce{Pt} \gt d_\ce{Au}$.
Relevant resources:
