Electrochemistry - Understanding Diffusion Potential

Question

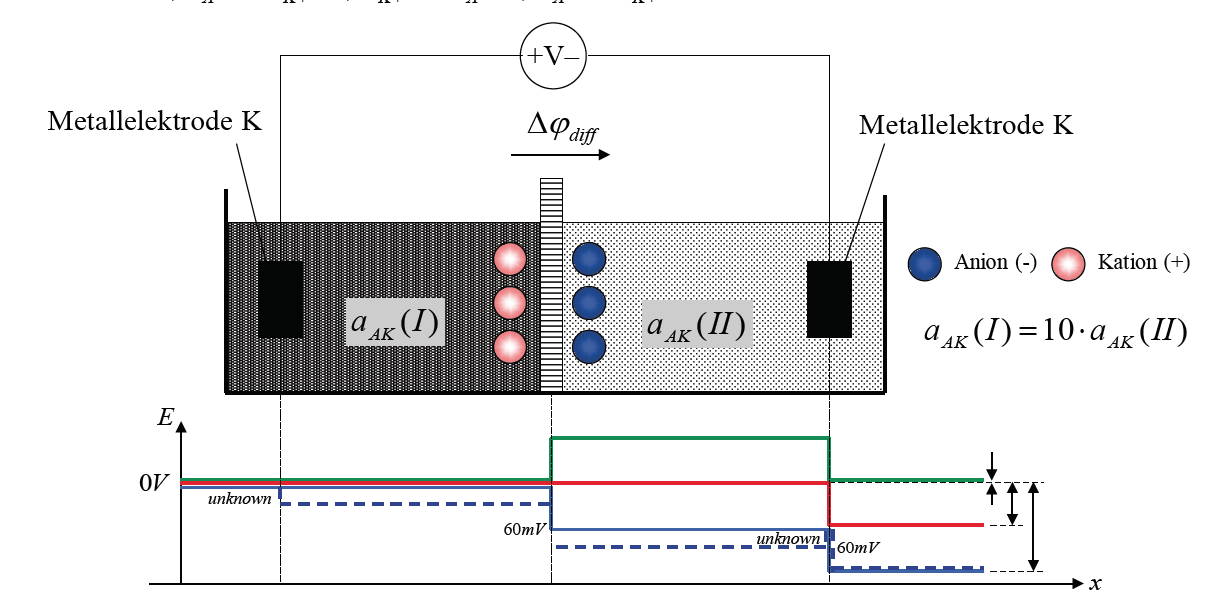
I have a big problem trying to wrap my head around this for several hours and I cannot seem to make sense of it. I have to link this scheme with the 3 colored potential profiles on the bottom of the picture. Considering the electrolyte AK, which splits into A- and K+, that it is 10x more concentrated in compartment I (left) and that the 3 different cases are the assumed:

1) Mobility of A- >>> than mobility of K+ 2) Mobility of K+ >>> than mobility of A- 3) Both ions have an equal mobility

Now, I know that in the 1) case, anions will diffuse through the membrane and work toward balancing their concentration. However, this will generate a potential difference because K+ cannot move and compartiment I will be left with a higher potential. Now, I can imagine that if we set our reference (left electrode) at an arbitrary number, let's say 0, then we can assume compartiment II being negative potential in respect to I. This leads to the constant value of the Nernst eq. for same metal electrodes and electrolyte with 10x concentration difference of 59 mV. Since II is considered lower potential VS I in case n°1, should I assume that the potential difference at the membrane's level is -59 mV (green profile)? And another +59 mV between the electrodes because of Nernst equation, which would bring to a final measured potential of 0V. Is this a legit reasoning?

Thank you in advance.

Answer 1

Let's discuss the problem in a qualitative way. In such a cell, the metal will get dissolved in the right-hand side of the cell, where the concentration is low, so that $[M^{z+}]$ increases in this compartment, which becomes the anode. In the left-hand side, the ions $[M^{z+}]$ are discharged and deposited as a metal layer on the electrode, which is the cathode.

The only problem is what is going on in the solutions. If the anion $A^-$ has a high mobility, it will flow from left to right through the membrane to compensate the chang of concentration of the metal ion. And after some time, the two solutions will have the same intermediate concentrations, and the cell will not deliver any current.

But if the ion $A^-$ has a low mobility, the cation $[M^{z+}]$ has a higher mobility and will easily cross the membrane from right to left. It will take a much longer time for the concentrations to be equalized in the two compartments. The reason is that a much smaller amount of ions $A^-$ will cross the membrane from left to right. But at the end, the two solutions will have the same concentrations.