4
$\begingroup$

One standard definition of equilibrium in beginner chemistry is that for a reversible reaction, (dynamic) equilibrium has been achieved when the rate of the forward and backward reactions are equal. We also define the equilibrium constant for a given reaction in terms of a ratio involving the concentrations of products and reactants at equilibrium.

Consider adding a small amount of solid $\mathrm{AgCl}$ to a sample of pure water, where the amount added is small enough that the solution does not become saturated. The associated reaction is

$$\mathrm{AgCl}(\text{s}) \rightleftharpoons \mathrm{Ag^{+}}(\text{aq}) + \mathrm{Cl^{-}}(\text{aq}), $$

with $K_{\mathrm{sp}}\approx 1.77\times 10^{-10}$. After the added amount of salt has dissolved, the concentrations of all species are constant since all of the $\mathrm{AgCl}$ has dissolved and if any solid $\mathrm{AgCl}$ is produced by the reverse reaction, it will be shortlived and will dissociate back into ions almost instantly.

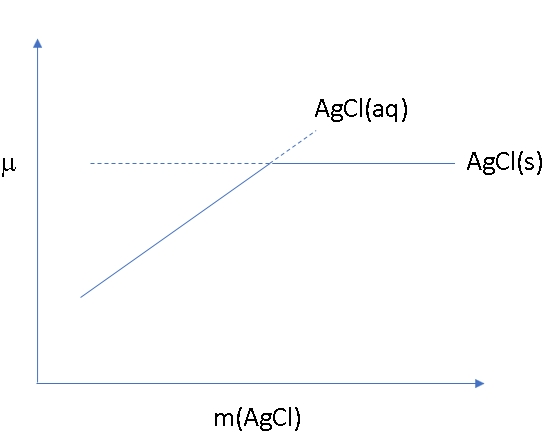
Is it not therefore true that the forward and backward reaction rates are equal at this point? By the simple definition of equilibrium given above, we would then conclude that the system has reached equilibrium. On the other hand, the reaction quotient $Q$ is less than the equilibrium constant $K_{\mathrm{sp}}$ since the saturation point has not yet been reached, and therefore the system has not reached equilibrium.

I am seeking an explanation of why this apparent contradiction arises – I expect it may be due to the basic definition of chemical equilibrium simply being inadequate, and that for equilibrium to be 'properly' achieved (in the sense of the equilibrium constant), the forward and backward reaction rates must approach each other smoothly rather than abruptly as they do in this example due to the $\mathrm{AgCl}$ running out before saturation is achieved. Or perhaps at equilibrium, the reaction rates are necessarily equal, but the converse is not true. You can assume that I'm familiar with thermodynamics and statistical mechanics.

Edit: The equilibrium constant here is calculated subject to the constraint that both a solid and an aqueous phase must coexist as pointed out in Charlie Crown's answer. This is also alluded to in Buck Thorn's answer where they note that there is no equilibrium with the solid below the solubility limit, and in a comment by Karl below their answer where they state that there is no dynamic equilibrium when the concentration of the solid is zero. Hence the apparent contradiction arises here because the static equilibrium achieved before the solubility limit has been reached is not the same as the dynamic equilibrium which $K_{\mathrm{sp}}$ refers to.

Improve this question
$\endgroup$
2
  • $\begingroup$ I would say a complex [AgCl(aq)] is partially formed, in equilibrium with ions. No AgCl(s) is formed, as AgCl(aq) concentration is not high enough. $\endgroup$
    – Poutnik
    Commented Nov 24, 2019 at 7:16
  • $\begingroup$ It doesnt matter why the forward and backward reaction have the same rate. That is the only proper definition of chemical equillibrium, all others are corollaries for certain situations. Obviously the maximum solubility is irrelevant here, as you are well below! $\endgroup$
    – Karl
    Commented Nov 24, 2019 at 8:50

3 Answers 3

Reset to default
3
$\begingroup$

Proper definitions of chemical equilibrium will not involve reaction rates whatsoever. Thermodynamics does not care about time. Chemical potential is the work required to form a molecule in solution, irregardless of the time it takes. Statistical Mechanics says chemical potential is the work required to move a molecule from infinitely far away (ideal gas since there are no interactions at infinity) into the solution.

Also, Free Energy is entirely independent of the equilibrium constant, but the equilibrium constant is not independent from Free Energy. At equilibrium, Free Energy will be at a minimum. Throw out definitions that use equilibrium constants. If you so wish, you can calculate the equilibrium constant from the free energy.

\begin{equation} K = \exp^{-\frac{\Delta G^0}{RT}} \end{equation}

Where $\Delta G^0$ is the ideal gas free energy reaction. If you know the ideal gas free energy change of a reaction, you may calculate K. However, K is a useless thing in reality, or at least, leads to more confusion than it is worth.

For chemical equilibrium, this is the definition at global equilibrium

\begin{equation} \left( \frac{\partial G}{\partial \xi} \right)_{T,P} = 0 \end{equation}

Where $\xi$ is the extent of reaction. In your case the free energy was minimized by the solid dissolving rather than staying a solid, so your system came to equilibrium given the constraint that there was no more solid that could be dissolved. There was also no constraint saying that there needed to be solid present.

Equilibrium and solubility limit are two different things. All equilibrium points are constrained by definition. At the very least you are constraining T and P. When you are measuring solubility you are actually implementing an additional constraint: find the minimum free energy of the system with the constraint that a pure solid and an aqueous phase must coexist - this necessitates there initially being enough solid to be able to meet this constraint at equilibrium.

Equilibrium reactions do not require time, and I have no time for equilibrium constants :)

Improve this answer
$\endgroup$
2
$\begingroup$

You have to look at two things in terms of equillibrium.

  • The pot with the solution, once all AgCl is dissolved and you have stirred it a bit more, is in equillibrium. Obviously. There is no chemical potential gradient, and you have only one phase. By itself, nothing will ever happen again in it.

  • The reaction (dissolution of AgCl in water) isn`t: If you add a bit more solid AgCl, the solution comes to rest at a different concentration!

Improve this answer
$\endgroup$
4
  • $\begingroup$ I see, so the reason why the reaction isn't at equilibrium is that the concentration would ultimately settle on a different value if more reactants were available. It seems the basic definition doesn't pick up on this important point. Would a more precise definition be that equilibrium is achieved for a given chemical reaction when the forward and reverse reaction rates are equal and the concentrations of both reactants and products are non-zero? $\endgroup$
    – Zac
    Commented Nov 25, 2019 at 3:10
  • 1
    $\begingroup$ You are trying to split a hair here. ;) If one side has zero concentration, I say you have no reaction. Why worry about the equillibrium? No solid precipitate, ergo dissolution rate zero, the solution is in equillibrium, ergo the precipitation rate must also be zero, so this cant be a dynamic equillibrium, but is just static. Which is indeed so: The rate of formation of crystallites can only become finite in supersaturation. Small crystallites are less stable thermodynamically. They cannot grow below saturation at all. $\endgroup$
    – Karl
    Commented Nov 26, 2019 at 0:14
  • 1
    $\begingroup$ Indeed, this is what I am trying to do! Perhaps then my issue is that the equilibrium constant for a reaction is defined at dynamic equilibrium, and has nothing to do with any of the static equilibria that may be attained for the same reaction due to a reactant running out before dynamic equilibrium has been achieved. I'm now satisfied that there is enough information between the three answers provided to resolve my initial question. Thanks! $\endgroup$
    – Zac
    Commented Nov 26, 2019 at 2:58
  • $\begingroup$ @Zac Exactly. A dynamic equillibrium constant only makes sense if there is indeed a dynamic equillibrium with two (opposite) equal, constant, and finite reaction rates. $\endgroup$
    – Karl
    Commented Nov 26, 2019 at 20:37
2
$\begingroup$

I expect it may be due to the basic definition of chemical equilibrium simply being inadequate

This is the answer (sort of). In essence, when you are below the solubility limit, the chemical potential of the solid lies above that of the solubilized salt, and there is no equilibrium with the solid (because no solid can form). This is illustrated in the following figure:

You might use this to formulate a microscopic argument such as "there is no thermodynamic force driving accumulation of the solid".

On the other hand, once the solubility limit is reached, the chemical potential of solid and solute become equal (this happens at the intercept of the two lines). Once this salt concentration is exceeded, the concentration of solute remains constant when more salt is added. Any added salt does not lead to increased solute since that would increase the solute potential above that of the solid.

Improve this answer
$\endgroup$
6
  • $\begingroup$ So before the solution becomes saturated there is no solid at all? But this seems contrary to the fact that in any reaction as much as high is the equilibrium constant there is some amount of reactants. $\endgroup$
    – Antonios Sarikas
    Commented Sep 10, 2020 at 13:51
  • 1
    $\begingroup$ @adosar Ksp as written conceals the third "reagent", the solid, which has constant chemical potential. It's better to think of this "reaction" as a phase transition, given that you form a solid with constant chemical potential (at constant p, T). The same would be true of water freezing or boiling at fixed pressure, say. $\endgroup$
    – Buck Thorn ♦
    Commented Sep 11, 2020 at 17:44
  • $\begingroup$ Does the same happen when we have a binary mixture of two liquids? If we add more and more from the first component then there would be a point where we should expect two phases, one with both components and another with only the first component (like when the salt precipitates)? $\endgroup$
    – Antonios Sarikas
    Commented Oct 13, 2020 at 17:19
  • 1
    $\begingroup$ @adosar Yes, this should apply generally. It should be true when for cases of limited solubility or miscibility. $\endgroup$
    – Buck Thorn ♦
    Commented Oct 13, 2020 at 19:40
  • $\begingroup$ "It's better to think of this "reaction" as a phase transition". Strictly speaking is the formation of the solution a chemical reaction? I was searching to see if mixing compounds is considered as a physical or chemical change but there is no clear answer. $\endgroup$
    – Antonios Sarikas
    Commented Oct 13, 2020 at 20:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.