Why do solutions of electron in a box (and in a ring) predict coefficients for LCAO (linear combination of atomic orbitals) in 1D systems?

Question

The solutions to the 1D particle in a box quantum mechanic system are standing waves (zero at both ends of the box) with 0,1,2... nodes for increasing energy (zero for the ground state).

If I look at the LCAO solutions for a linear system, combining AOs of the same shape and energy (e.g. conjugated double bonds, linear array of sodium atoms), the coefficients follow the pattern of a standing wave with 0,1,2... nodes. For example, when I combine 3 AO's in a row, the solutions are +++, +0-, +-+, i.e. zero, one, and two switches of signs as I go to higher energy states. For the state with one node, the node is in the center of the box, corresponding to the middle AO, which has a coefficient of zero. (More examples, i.e. hexatriene and pentadienyl cation, are on slide 126 and 128 of this document.)

The solutions to the particle in a ring system are standing circular waves (same value at 0 and 360 degrees) with 0,2,4... nodes for increasing energy. The animation shows a standing circular wave with 8 nodes:

If I look at the LCAO solutions for a circular system, again combining AOs of the same shape and energy (e.g. p-orbitals in an aromatic system), the coefficents follow the pattern of a circular wave with 0,2,4... nodes. For benzene, the solutions are ++++++, +++--- and +0--0+, +-++-+ and -0+-0+, +-+-+- (see picture):

What underlies the connection between the unbound electron in a box or corral with the formation of covalent bonds of bound electrons?

Answer 1

A short answer is that the functions that satisfy the Schrodinger equation for a system are largely determined by the potential energy part of the function, since that's the part of the Hamiltonian that varies with different scenarios. The particle-in-a-well (= 1D box) is defined by having infinite potential energy outside the walls and a constant finite energy within. That turns out to be a very good approximation of the potential energy function of a pi system or a line of identical metal atoms.

At the end of the line of atoms, an electron faces a steep potential energy curve. Not quite the vertical wall of the 1D box, but pretty close to it. The same is true on the sides, so the system can be treated as 1D. Within the system, the electron moves essentially freely (or exists in a broadly delocalized way) with very little change in potential across the system.

Since the potential functions are nearly identical, the solutions to the Schrodinger equation take the same mathematical form.

Answer 2

I want to add a slightly different but related perspective to the excellent answers already posted: Comparing the Hamiltonian of particle in a box and for Hückel theory. First though, I want to compare boundary conditions and potential (Coulomb) energy.

Boundary conditions

For the particle in a box, the boundary conditions are explicit: The particle has one degree of freedom (1D) and has to be inside the box. For LCAO, there are implicit boundary conditions: The electrons can only be where they would be in the atomic orbitals.

Potential energy

For the particle in a box, there are no Coulomb interaction within the box; this is a free electron in that region. For the Hückel theory in its simplest version (all p-orbitals, all carbon atoms) of LCAO, the Coulomb interaction is encoded in the $\alpha$ parameter (pre-computed, or just given as a conceptual parameter). The two scenarios are very similar: there is a region of low energy (inside the box or near the atoms) and a region of high energy (outside the box or away from the atoms). You can either describe this as "confining potential" (as in the answer by Buck Thorn), or you can describe this as absence of Coulomb term in the Hamiltonian because the boundary conditions (and the choice of basis functions for Hückel theory) take care of the "confining".

The Hamiltonian

For the particle in a box, the Hamiltonian is just about the kinetic energy (no electrostatic interactions), so it is simply the second derivative with some constants:

$$ H = -\frac{\hbar^2}{2m} \frac{d^2}{dx^2}$$

For Hückel theory, the Hamiltonian is not explicitly shown, but the result of $⟨ϕ_i|H|ϕ_j⟩$ are given as $\alpha$ for i=j, as $\beta$ if i and j are neighbors, and are neglected (set to zero) otherwise. The $\alpha$ corresponds to the Coulomb interaction, and its contribution is constant. With the atomic orbitals as basis functions, you might say the electrons are free otherwise (this amounts to saying that the Coulomb interaction is the same around each atom). In a way, the Coulomb interaction is taken out of the equation because all the AO's have the same energy, and the MO's are just linear combinations of them (so there will not be more electron density between atoms for the MO's compared to the AO's - still, the electrons are more delocalized and therefore have lower kinetic energy).

The $\beta$ term roughly corresponds to the kinetic energy. If two adjacent AOs are combined with equal signs of coefficients, the resulting wave function is smoother (has a smaller second derivative) than when the signs of coefficients are opposite. Another way of saying that is that if the coefficients "have a node", the kinetic energy is higher. So this models the kinetic energy part of the Hamiltonian.

The solutions

If the Hamiltonian (or the implicit Hamiltonian) are similar because the only relevant term for finding a solution is the kinetic one, then the resulting wave functions are expected to be similar. One difference, though, is that the particle in a box can have arbitrarily high energies (number of nodes) while the LCAO result has a finite number of energies constrained by the available basis functions.

Answer 3

Attractive Coulombic interactions impose a confining potential, and single electrons, being wave-like in character, will display standing-wave probability densities within such a potential. In a 1-D system, lower energy corresponds to probability density distributions with less nodes, because highly spatially-varying wavefunctions correspond to a higher electron kinetic energy. All of this is explained here in an excellent answer to another post. In the case of an aromatic ring, the location of the nodes can be inferred from the fact that the approximate MOs are constructed from AOs consisting of p-orbitals, which have density centered above the nuclei on the plane above the ring.

In summary,

• the wave character of electrons accounts for the "standing wave" appearance of the density
• the wavefunctions are sensitive to the potential, specifically the location of nuclei, but symmetrical distribution of nuclei (as in a ring or line) will result in nice symmetrical MOs that might make important details less obvious
• the 1D character of the potential accounts for the proportionality of number of nodes and energy

Finally, I should point out that the MO's constructed to generate the solution to the aromatic ring problem are obviously finite in number, as the AOs used to construct them are finite in number (#MOs=#p-orbitals). The same is true in a sense of the sodium row problem (#MOs=#AOs=#1s orbitals). This is absolutely not true for the particle in a box. There you don't need more AOs (or atoms) to construct a new wavefunction, and the position of the nodes is determined by the number of nodes and size of the box, not by the position of any nuclei. The analogy breaks down because you are not constrained by any AOs (or nuclei).

Answer 4

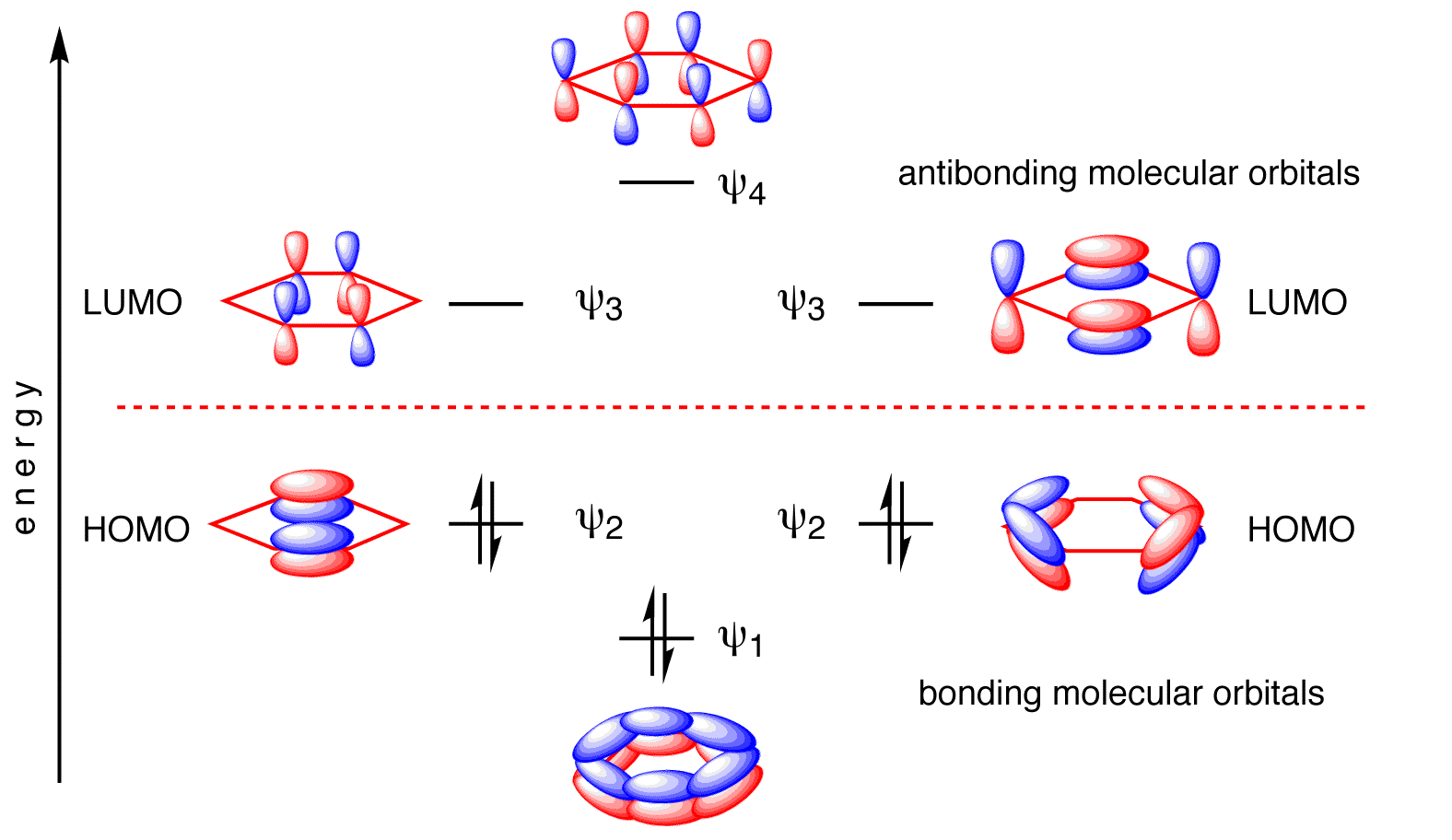
To answer this question it is advantageous to treat a molecule as a graph and use the well known adjacency matrix from graph theory. Here is the wikipedia definition:

For a simple graph with vertex set $V$, the adjacency matrix is a square $|V| × |V|$ matrix $\mathbb{A}$ such that its element $\mathbb{A}_{ij}$ is one when there is an edge from vertex $i$ to vertex $j$, and zero when there is no edge.

Hückel

The Hückel-Hamiltonian $H$ can be written as:

$$ H = \alpha \mathbf{1} + \beta \mathbb{A}$$

Where $\alpha$ is the ionization energy, $\beta$ the overlap between adjacent $p_z$ orbitals, $\mathbf{1}$ the unit matrix, and $\mathbb{A}$ the adjacency matrix.

If you look at the eigenvalue equation, $$H \psi = \lambda \psi $$ it has a solution if and only if $\psi$ is an eigenvector of the adjacency matrix. The eigenvalue $\lambda$ of $H$ is given by the eigenvalue $\tilde{\lambda}$ of the adjacency matrix using the following equation: $$(\alpha \mathbf{1} + \beta \mathbb{A}) \psi = \alpha \psi + \beta \mathbb{A} \psi = (\alpha + \beta \tilde{\lambda}) \psi$$

What we see is that the $\alpha$ is just a constant offset for the energy. All the relevant information about level spacing, degeneracy etc. is contained in the $\tilde{\lambda}$ with a scaling factor $\beta$.

To put this in another way: All relevant properties of the Hückel-Hamiltionian are encoded in the adjacency matrix of a graph/molecule.

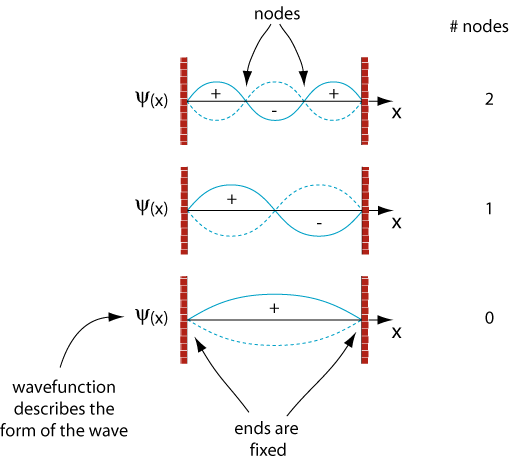
Particle in a box (Free electron network model)

There is a nice paper by Ruedenberg and Scheer 1953 about that topic here. The main idea is, that your 1D-particle-in-a-box solutions on each edge/bond have to be constrained at joint vertices/atoms. These constraints are "derived by intuition" in the cited paper.¹ You want the whole wavefunction, that is piecewise composed of the wavefunctions on each edge, to be continous. Similar to Kirchhoff's Law for electric circuits you want to assert, that the probability current that flows into an vertex/atom flows out. For this reason, the constraints are called Kirchhoff conditions.

If $V$ is the set of all vertices/atoms, $\psi^e$ labels the wavefunction at the edge/bond $e$, and $E_v$ contains all edges/bonds on a vertex/atom $v$. Then you can express your constraints as:

1. Continuity: $$ \forall v \in V: \forall e_1, e_2 \in E_v: \psi^e_1(v) = \psi^e_2(v) $$
2. Flux Preservation: $$ \forall v \in V: \sum_{e \in E_v} (\psi^e)' (v) = 0 $$

Without going into the details of the derivation: for cyclic graphs/molecules your eigenvalues $\lambda$ are given again by the eigenvalues $\tilde{\lambda}$ of the adjacency matrix $\mathbb{A}$. The free scaling factor is then $L$ the edge/bond length (Up to some fixed constants like number literals or $h$.)

Conclusion

For cyclic molecules, it can be proven that the essential properties of the spectrum are given just by the adjacency matrix $\mathbb{A}$ of the molecule in both cases.

The parameter $\alpha$ in the Hückel-Formalism introduces a constant offset, which can be usually ignored for chemistry.

This means that both methods have only one free parameter that scales the spectrum of the adjacency matrix. In the case of Hückel it is the overlap between adjacent $p_z$ orbitals, in the case of the free electron network model it is the bond length $L$.

---

¹ Note that you can rigorously derive general constraints by forcing only self-adjoint/hermitian realisation of the hamiltonian on the graph. This can be found e.g. here. But we are chemists and not mathematicians so let's stick to the Kirchhoff conditions.