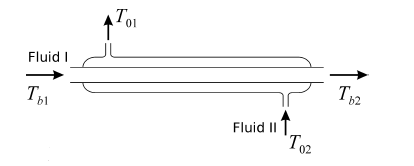
The single-tube heat exchanger is used to increase the temperature of fluid I from $T_{b1}$ to $T_{b2}$. The heating oil (fluid II) used for this task enters at temperature $T_{02}$ and leaves at temperature $T_{01}$.
a) Give the expression that describes the average heat flux from fluid II to fluid I.
I don't really know how to derive the average heat flux but the equation I immedietly think of using is: $$q = U(T_{b1} - T_{b2})$$
Still I am not sure how I should calculate the overall heat transfer coefficient and also I recall being told that when dealing with heat exchangers you should use the logarithmic temperature difference. So the expression should be:
$$q = UΔT_{\ln}$$
where the logarithmic temperature difference is
$$ΔT_{\ln} = \frac{(T_{01} - T_{b1}) - (T_{02} -T_{b2})}{\ln\frac{T_{01} -T_{b1}}{T_{02} - T_{b2}}}$$
And an attempted solution for the overall heat transfer coefficient is:
$$U = h_1 + \frac{k}{R_0\ln(R_a/R_0)} + \frac{h_2R_a}{R_0}$$
but I feel like this isn't correct since it is used when calculating the heat transfer in a heat exchanger and not the heat flux. Is it correct or is the heat flux for a heat exchanger calculated in a different way?
All help is greatly appreciated!