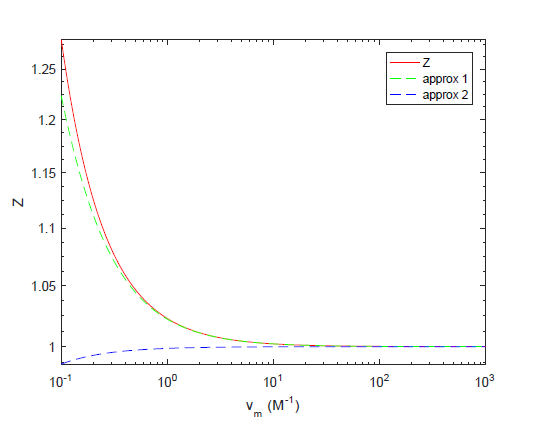
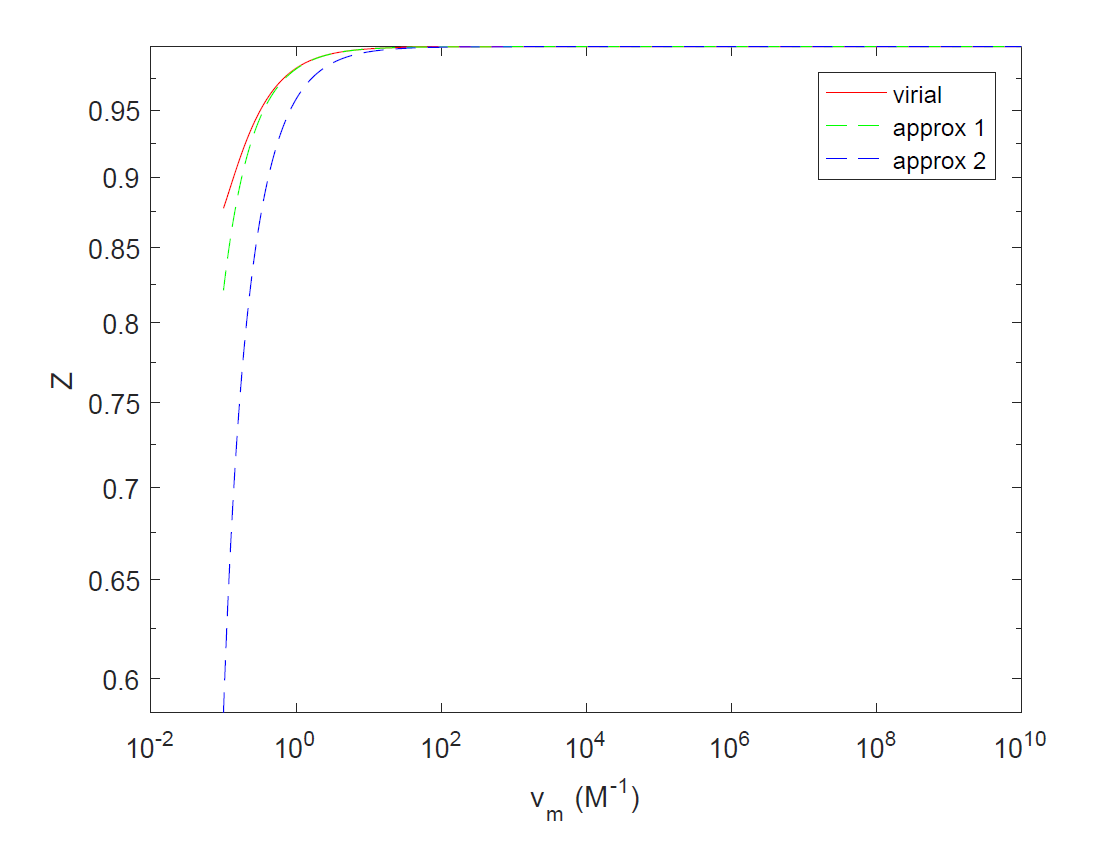
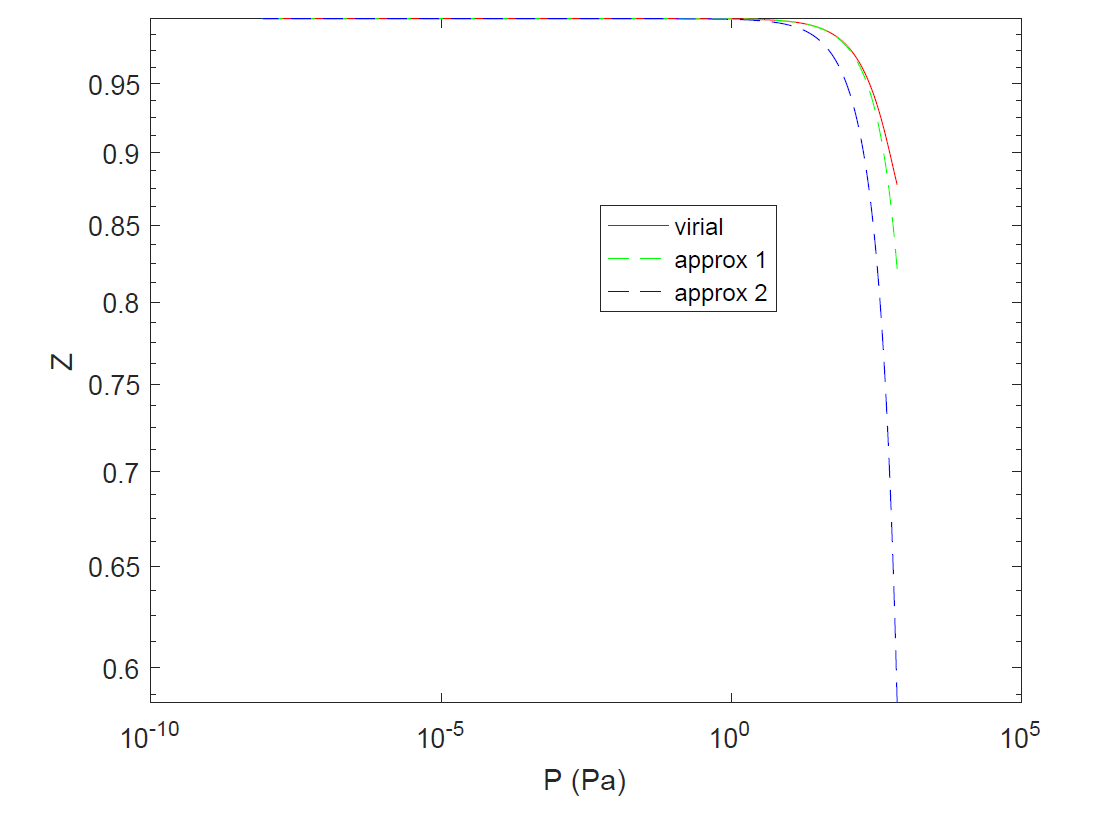
As we all know, the compressibility factor $Z$ of hydrogen and helium is always greater than $1$ at a constant moderate temperature. But, if we look at the virial equation of compressibility factor
$$Z = 1 + \frac{1}{V_\mathrm{m}}\left(a - \frac{b}{RT}\right) + \frac{b^2}{V_\mathrm{m}^2} + \cdots$$
At extremely low pressure $V_\mathrm{m}$ would be extremely large. So, the equation simplifies to
$$Z = 1 + \frac{1}{V_\mathrm{m}}\left(a - \frac{b}{RT}\right)$$
Now, the values of $a$ for hydrogen and helium are extremely small. So, we can take them as zero. The equation further simplifies to
$$Z = 1 - \frac{1}{V_\mathrm{m}}\left(\frac{b}{RT}\right)$$
Now, the value of $b$, $R$, $T$ and $V_\mathrm{m}$ are all positive. Though the value of $b$ for hydrogen is extremely small, still it is positive. So, shouldn't the value of $Z$ at some small pressure be less than $1$?
Any help would be appreciated.