Does a molecule need to be placed symmetrically in the unit cell?

Question

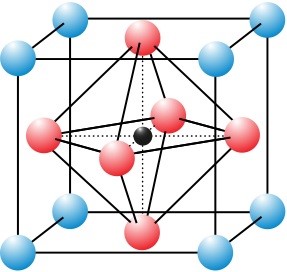
I was watching an OCW by MIT (3.091X) on solid state. My previous notion of lattice point and unit cell got shattered after this example.

Where are the unit cell and lattice points in this picture?

Unit Cell is the smallest group of atoms which has the overall symmetry of a crystal, and from which the entire lattice can be built up by repetition in three dimensions.

Lattice is the arrangement of points in space such that any point us identical to any other.

So using these definitions, the answer is

Green is the unit cell, and red ones are the lattice points.

Previously I used to think that a molecule should be symmetrically placed in a unit cell such that its center of mass lies on the lattice point. But in this example, the center of mass (shown by the yellow-green circle) is way off the lattice point.

My question is, Are there any other similar examples where the center of mass of molecule doesn't coincide with the lattice point or at least doesn't placed symmetrically in a unit cell (like on the face center, body center)?

Answer 1

Don't think of it so much as putting the center mass of an atom on a vertex but as matching the unit cell symmetry to the crystal symmetry. Take the definition from google below, I've bolded key parts:

The smallest group of atoms of a substance that has the overall symmetry of a crystal of that substance, and from which the entire lattice can be built up by repetition in three dimensions.

While the red boxes satisfy the first and last bolded criteria, they fail the second. The red boxes have 2-fold rotational symmetry and inversion symmetry, but the green boxes have 2 mirror planes that the red boxes do not. This lack of mirror symmetry with the red boxes does not have the same overall symmetry of the lattice and therefore the green box is the unit cell.

Think of Sodium Chloride; its unit cell face is represented as:

$\ce{\color{red}{Na}\ Cl\ \ \color{red}{Na}}$
$\ce{Cl\ \ \color{red}{Na}\ \ Cl}$
$\ce{\color{red}{Na}\ Cl\ \ \color{red}{Na}}$

instead of

$\ce{\color{red}{Na}\ Cl}$
$\ce{Cl\ \ \color{red}{Na}}$

Because the first example has 4-fold rotational symmetry where as the second has 2-fold and the first one has 2 mirror planes that the second example does not. Both have inversion symmetry. That said the atoms at edges/corners are centered because that is what allows for the symmetry otherwise the unit cell would be unsymmetrical which does not reflect the symmetry of the lattice.

As an example of a sort of off centered lattice there is perovskite. You can't see it in the image but the black atom is $\ce{Ti^4+}$ and is too large to actually center in the lattice so it will reside in an off-centered configuration (the titanium ion is shifted off-center towards a face). This property of off-centering makes perovskites excellent as dielectric materials for capacitors as the ion can change is off-centering by an electric field.