I have drawn the Lewis structure of the oxalate ion, and I see that two oxygens are double bonded to the carbons. When finding the resonance structures, I said there are 2, but the exam solutions state 4, which was confirmed by internet images such as this:
However, I see no real difference btween structures 1 and 2, and 3 and 4. Are they considered different resonance structures?
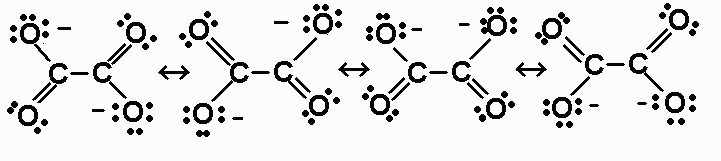
Resonance structures are not real things. As also indicated by rbw, they have weight on the actual compound. Simpler example is ozone.
http://www.wou.edu/las/physci/poston/ch222/pdf/Ch08-s12-part2.pdf
From the first slide second image there;
you can see that the actual molecule is none of the "resonance" structures, and both bonds between leftmost and rightmost oxygen atoms with central oxygen atoms have equal lenght, and it is between 1 bond length and 2 bond length.
The general idea of drawing resonance structures is, after predicting the most appropriate ones, when you superimpose them and average the bond lengths you will be very near to see the real chemical bonds lengths.
This is straightforward with ozone, oxalate or carbonate etc. but things get complex with increasing atoms, though.
And wrapping up and also answering the question you posed, you should look atoms as if they are all unique, like oxygen 1 oxygen 2 oxygen 3 and oxygen 4. Flip the molecule and you will NOT get the other one, this is not like isomerism. It is just to predict the electron cloud density around atoms of a molecule.
Hope this also helps.
If you begin from the skeleton of the oxalate ion and consider the formal charges than you will find these four structures which are different as pointed out by Alchimista. However, you have structures 1 and 2 contributing equally to the whole system and as well as structures 3 and 4. This is supported by a simple symmetry analysis where structure 2 and 4 can be obtained by a 180 deg. rotation along the C-C axis of structures 1 and 3, respectively.
