3
$\begingroup$

The question is:

Ions $\ce{B}$ and $\ce{C}$ react to form complex $\ce{BC}$. If $\pu{15.0 mL}$ of $\pu{1.00 M}$ $\ce{B}$ is combined with $\pu{15.0 mL}$ of $\pu{1.00 M}$ $\ce{C}$, and our initial concentration of BC is $0$, yet the end in equilibrium for complex $\ce{BC}$ is $\pu{0.00450 mol}$ determine the equilibrium constant for this reaction.

Firstly, I converted the total volume $\pu{15.0 mL + 15.0 mL = 30 mL}$ to L and I got $\pu{0.03L}$

Secondly, I used the given $\pu{0.00450 mol}$ and divided it by $\pu{0.03 L}$ and I got $\pu{0.15 M}$ for BC

Thirdly, I did the ICE chart

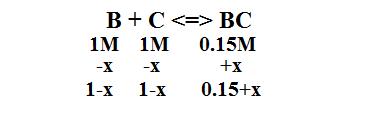
enter image description here

After that, I used the quadratic formula to solve for $x$ and calculate the concentration for each substance, as well as getting the $K_\mathrm{c}$ at equilibrium

enter image description here

I got $1.53$ for $K_\mathrm{c}$ but I am not sure if the whole process is correctly done. Please point out my mistakes

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
2
$\begingroup$

You made a slight error in calculations for B and C. While those concentrations are correct, that is 1 M at 15 mL. When doing RICE tables and equilibrium calculations, we can use concentrations, however I prefer to convert concentration into moles.

We are trying to find $K_c$, and we know that equal moles of the reactant react to give us 0.0045 mol of BC.

$$\ce{B + C <=> BC}$$

\begin{array} {|c|c|c|c|} \hline \text{Initial conc.} & \text{0.015 mol} & \text{0.015 mol} & \text{0 mol}\\ \hline \text{Change conc.} & -x & -x & +x\\ \hline \text{End conc.} & \text{0.015 mol} - x & \text{0.015 mol} - x & 0 + x \\ \hline \end{array}

We know that our change in equilibrium is 0.00450 mol, as that is the end amount of product that we have in our equilibrium calculations.

Accounting for the concentrations, we divide our number of moles by the total volume to get mol/L. 0.5 mol/L, 0.5 mol/L, .15 mol/L.

Assuming that our reaction is at equilibrium:

$$K_c = \frac{[\text{0} + \text{.15 mol/L}]}{[\text{0.5 mol/L} - \text{.15 mol/L}][\text{0.5 mol/L} - \text{.15 mol/L}]} $$

$$K_c = \frac{[\text{.15 mol/L}]}{\text{[0.35 mol/L]}^2} $$

$$ K_c = 1.22 $$

Thanks @Nicolau for pointing out the error in the question and reviewing my work.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thank you for showing the work in how to do this question and pointing out the fact that concentrations can also be used in this case. However, I am confused in how to get 0.015 mol for B and C. Did you divide 0.00450 mol by 0.03L to get 0.015 mol? Moreover, do we need to use the quadratic formula to answer this question? Based on your work, it seems like we do not need to. $\endgroup$
    – Jesse
    Commented Mar 10, 2014 at 0:14
  • $\begingroup$ @Jesse Dear Jesse, for B and C, I divided 1 M by 15 mL to get the number of moles. However, at the end, I needed to convert once again back to concentration, so I divided by the total volume 30 mL and multiplied by 1000 to get mol/L. We do not need the quadratic formula, since we are trying to find K. We know what the end of concentration is in our RICE table, the lower right hand corner. Since we know by reaction stoichiometry that it increased by .15 mol, therefore x is .15 and we plug that into our equilibrium expression. $\endgroup$
    – user3735
    Commented Mar 10, 2014 at 0:22
  • $\begingroup$ Dear Mr. Kwak, thank you once again for the clear explanation! Since 1 M = 1 mol over 1000 mL, and we have 15 mL, so we multiply 1 mol over 1000 mL by 15 mL and we are left with 0.015 mol. Is this correct? I finally get it, thank you! :) $\endgroup$
    – Jesse
    Commented Mar 10, 2014 at 0:44
  • $\begingroup$ @Jesse Exactly. And since we are in moles at the end, we have to multiply by our total volume to get back our concentrations. And thus, we plug those concentrations into our equilibrium expression. It was my pleasure. :) $\endgroup$
    – user3735
    Commented Mar 10, 2014 at 0:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.