From molecular orbital theory, it has no unpaired electrons but can we conclude it is diamagnetic considering the fact that it does not exist?
$\begingroup$
$\endgroup$
1
-
4$\begingroup$ Technically exists: 1, 2, 3 Don't think they managed to measure its magnetic moment though. (Disclaimer: I didn't read through) $\endgroup$– orthocresolCommented Jun 4, 2017 at 10:47
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
Diberyllium is thought to be non-existant. This website explains how diberyllium cannot exist in brief and simple language. According to it:
A simple MO diagram explains why $\ce{Be2}$ must be indefinitely unstable. When the two beryllium 2s orbitals interact they split in two energy levels - one bonding (lower energy) and one antibonding (higher energy) - and when the four electrons of both Beryllium atoms are divided over these two new molecular orbitals(symmetric and antisymmetric, or g and u combinations of the 1s orbitals) ,both levels get fully occupied and there is no net energy gain and therefore no bond.
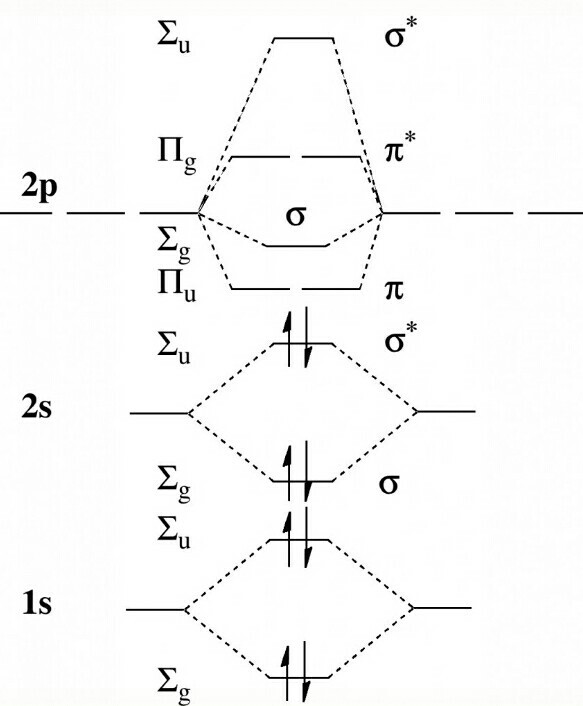
The compound does not exist but that doesn't mean its MO diagram cannot be made. If you look at the MO diagram taken from this site:
You can see that electrons in each energy levels are paired and hence diamagnetic. From that site:
Actually, if a quantum mechanical calculation is performed (at the ωB97XD/6-311G(d,p) level), the bond length emerges as 2.81Å and a vibrational wavenumber of 167 $\ce{cm^{-1}}$ is predicted. Despite the zero bond order, a weak bond IS predicted, and this is the van der Waals or dispersion bond.
You can also check the links @orthocresol sent for more information about diberyllium.
answered Jun 4, 2017 at 17:37
$\begingroup$
$\endgroup$
2s-2p mixing means combining the s and orbitals together with the same plane lying and associated with the same energy which leads to the energy change and the bond order respectively. And From the MOT concept Be2 doesn’t exists as its Border is 0 and in case of para or dia it is diamagnetic.
