0
$\begingroup$

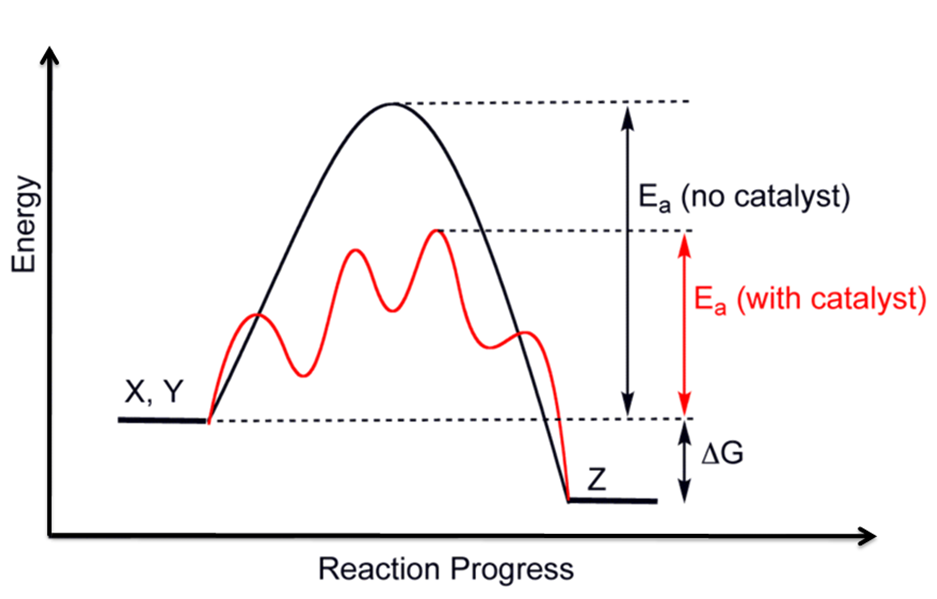
I am looking at the image below from the Wikipedia page for energy profiles in chemistry. I do not understand why the "$E_a$ (with catalyst)" is labeled as it is. I would argue that is not the activation barrier with the catalyst. Shouldn't all of the individual steps need to be considered to get the rate of production of product (or, if one step has an especially high barrier, then that step can be assumed to be rate-limiting and that step's $E_a$ is the barrier with the catalyst)? I suppose in other words, if I do $r = A\exp(-E_a/k_{B}T)$ where $E_{a}$ is the activation energy with catalyst, that will not get me the rate, right?

Activation energy with and without catalysts

For the record, I have taken grad-level courses in chemistry, but I got myself into a position of questioning everything I once learned and here I am.

Improve this question
$\endgroup$
2

1 Answer 1

Reset to default
0
$\begingroup$

Of course, in a strict sense the reaction path using a catalyst should be split into four sub-reactions (all going by the exact image) and each of those sub-reactions should get their own activation energy assigned. Two of the four steps would be endothermic, two exothermic. (Note that it is not necessary for the endothermic steps to occur first; it could be the other way around, mixed or anything.)

However, there is still some truth in labelling ‘$E_\mathrm{a}$ with catalyst’ as such. Even though you have much smaller steps you will still need to add that labelled energy in net terms to overcome the barrier. The principal difference is that it need not be added all at once but can be added stepwise. You could even say that the total activation energy (i.e. adding up all the individual $E_\mathrm{a}$) be even higher, but you regain some of that from the intermediate reactions.

If you decided to supply only enough energy so that a molecule could jump the barrier from intermediate 2 to intermediate 3, that would not make the reaction go. For one, the barrier to get to intermediate to is larger by itself. For two, you would only get that much above the baseline which might be enough to cross the barrier to intermediate one but no further.

Improve this answer
$\endgroup$
7
  • $\begingroup$ I agree with all of that, and the rational for the labeling does make some sense that way (although not in the context of computing a rate). I now have a follow-up question though. If instead of 4 steps I have 100 steps (everything else otherwise the same), with the highest step having the same height as the 3rd transition state shown in the Wikipedia image, will that impact my rate of production of product? Yes, right? $\endgroup$
    – Argon
    Commented Dec 14, 2016 at 2:10
  • 1
    $\begingroup$ @Argon No. The barrier is the largest difference from a stable species (reactant or intermediate) to any subsequent transition state. Having lots of steps doesn't matter because interconversion is fast if the barriers are small. $\endgroup$
    – Zhe
    Commented Dec 14, 2016 at 3:50
  • $\begingroup$ @Zhe Doesn't that implicitly assume I am dealing with a rate-limiting step? I'd prefer not to make the assumption that one step is by and far much larger than the others, just that there is indeed a maximum barrier height. $\endgroup$
    – Argon
    Commented Dec 14, 2016 at 3:58
  • $\begingroup$ It still works because the other steps will be under equilibrium. All you need to assume is that you have enough energy to surmount the barrier. Since energies add, everything just works out. $\endgroup$
    – Zhe
    Commented Dec 14, 2016 at 4:06
  • $\begingroup$ @Zhe Thank you for your replies. I am going to have to convince myself of this. I ask because the question that motivated me to even submit the original question was the following. Is the rate the same between: Case 1) the reaction proceeds in one step with a barrier height that goes to $E_{a}$ compared to the reactant; Case 2) That same reaction is actually two sequential steps, where the highest of the two peaks reaches a height $E_{a}$ compared to the reactant. Everything else is identical. From your answer, it seems the answer is yes, the two cases have the same rate. $\endgroup$
    – Argon
    Commented Dec 14, 2016 at 4:40

Not the answer you're looking for? Browse other questions tagged or ask your own question.