1
$\begingroup$

I am using TINKER package to calculate the external reorganization energy for pentacene crystal. But I have problems with that - I've generated .xyz file of 5 molecules with VESTA and I need to perform the calculation on those five molecules (image below) with periodic boundary conditions (PBC).

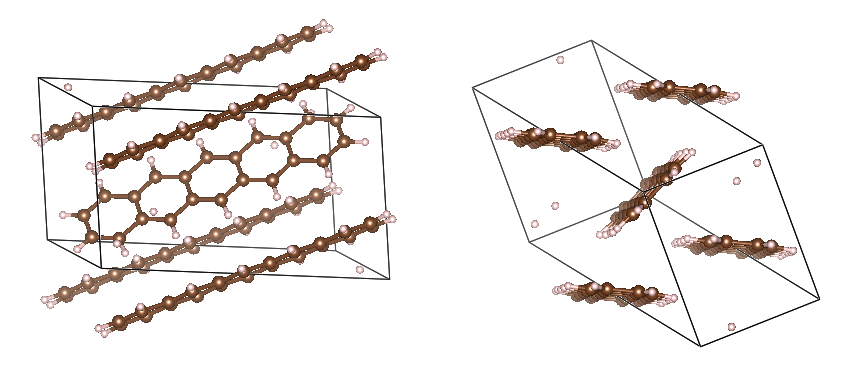
image of crystal generated with VESTA

How can I find the proper size of cell and keep it fixed for calculations? When I use keywords A-axis, B-axis, C-axis and alpha, beta, gamma and assign lattice vectors and angles to them after optimization or minimization for the charged system like that apparently cell gets broken and molecules are distorted out of box:

the same molecules from two sides after optimization

Improve this question
$\endgroup$
4
  • $\begingroup$ I'm not sure I see in your image how the molecules (or cell) are "broken." $\endgroup$
    – Geoff Hutchison
    Commented Sep 9, 2016 at 14:22
  • $\begingroup$ If you have the unit cell above, then you can specify the size of the box based on A, B, C, $\alpha$, $\beta$, $\gamma$ as you indicate. I don't believe that Tinker will change the cell parameters during a run. $\endgroup$
    – Geoff Hutchison
    Commented Sep 9, 2016 at 14:36
  • $\begingroup$ @Hutchison - sorry, maube the word broken is not the best, bus the point is thay molecules are no longer in the box, because the size of the box was set so they fit accuratelly in it. And in the image you can see that they for example are two of them in z axis (the one along the pentacene). $\endgroup$
    – cinnamon
    Commented Sep 9, 2016 at 15:01
  • $\begingroup$ btw, I suggest this site for finding reference structures of simple compounds (follow the link). google.ru/… $\endgroup$
    – permeakra
    Commented Sep 9, 2016 at 20:22

1 Answer 1

Reset to default
3
$\begingroup$

It's a little hard to tell from your second visualization, but to me, it simply seems as if the molecules have shifted somewhat with respect to the periodic box.

A unit cell or periodic boundary "box" is simply a frame of reference. There's no walls. The molecules don't "see" it.

It's merely an indication that there's translational symmetry. In short, in the left-hand view of the second image, the molecule "breaking the box" is also wrapped around into the box by translational symmetry.

I don't know what visualization tool you're using, but if you display more than one unit cell, you'll easily see that the molecules "wrap" around.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.