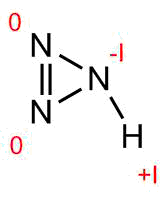
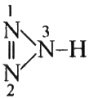
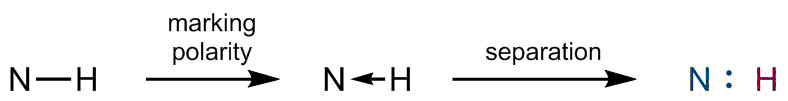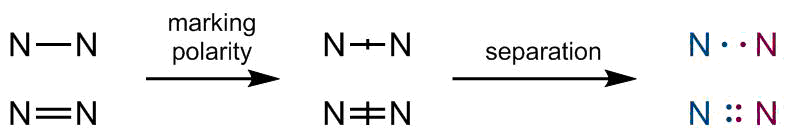6
$\begingroup$

The book asks me to find the oxidation state of nitrogen in the compound below (the structure is not the one given in Wikipedia for HN3):

triangular structure of HN3

To find the oxidation state using Lewis structure, I have to compare the electronegativity of the bonded atoms and assign the bonding electrons to the more electronegative atom. Then, the difference between the number of electrons in element state and bonded state will give the oxidation number.

Here, the bonding electrons of nitrogens bonded with a double bond will stay in between both the atoms will not be assigned to both. The $\ce{N-H}$ bonding electrons will be assigned to $\ce{N}$. What about the two $\ce{N-N}$ bonding electrons?

Improve this question
$\endgroup$
5
  • 12
    $\begingroup$ Throw away the book. This structure is hideously wrong. And anyway, you may assign these oxidation states more or less arbitrarily (just make sure they sum up to 0), so it does not really matter. $\endgroup$
    – Ivan Neretin
    Commented Feb 27, 2016 at 1:49
  • 2
    $\begingroup$ To be fair he doesn't say whether the book calls this hydrazoic acid, or if it's what he is calling this. Greenwood and Earnshaw does (very briefly) mention the above so I assume it is known somehow. $\endgroup$
    – Ian Bush
    Commented Feb 27, 2016 at 8:17
  • 2
    $\begingroup$ The given structure belongs to triazirine, not hydrazoic acid. $\endgroup$
    – aventurin
    Commented Feb 27, 2016 at 12:45
  • $\begingroup$ Ok, how is there triazirene? It's antiaromatic if planar and highly strained. $\endgroup$
    – Oscar Lanzi
    Commented Mar 12, 2020 at 15:12
  • 1
    $\begingroup$ @OscarLanzi I deleted the comment. The compounds are unstable and may have decomposed just by sitting in the comment section for 5 years. (Sorry for the terrible joke). $\endgroup$
    – Nilay Ghosh
    Commented May 21, 2021 at 2:47

1 Answer 1

Reset to default
11
$\begingroup$

This answer uses electronegativities for the calculation of oxidation states as proposed in the Expanded Definition of the Oxidation State by Hans-Peter Loock in 2011.

Comparing Electronegativities

The following table shows an excerpt from Pauling electronegativities ($\chi_{\mathrm{Pauling}}$):

$$\begin{array}{cc} \hline \text{Element} & \chi_{\mathrm{Pauling}}\\ \hline \ce{H} & 2.20\\ \ce{N} & 3.04\\ \hline \end{array}$$

Looking at $\ce{N-H}$ Bond (heteroatomic)

The table shows, that the nitrogen atom is more electronegative than the hydrogen atom. It will therefore attract the electrons more than hydrogen does, and thus polarize the bond. Imagining the gedankenexperiment of an hypothetical heterolytical bond cleavage - presumed by applying pulling force to the atoms, until they get separated - it is assumed, that the electrons are allocated to the nitrogen atom during separation.

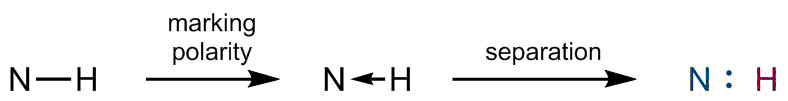
Behaviour for heteroatomic N-H bond

Looking at $\ce{N-N}$ and $\ce{N=N}$ Bonds (homoatomic)

Looking again at the previous gedankenexperiment, now with two identical Atoms bound to each other. Since they have, of course, the same electronegativity, there is no polarization of the bond. It is assumed, that separation would therefore result in an homolytical bond cleavage, allocating always one electron to each of the two nitrogen atoms for each bond respectively.

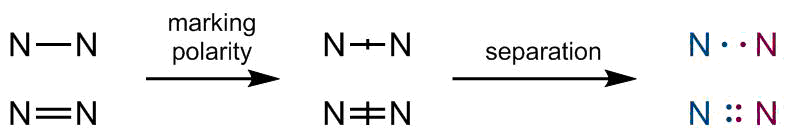
Behaviour for homoatomic N-N or N=N bond

Summing up

Now, having a look on the complete structure of the molecule in question, and applying the previously states rules:

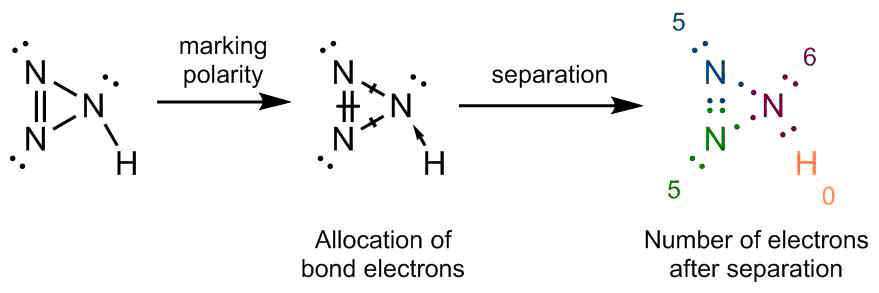
Applying rules to target molecule

Last thing to do is calculating the atoms hypothetical charge after separation, which is to be equatable with the oxidation state:

$$ \text{Oxidation state} = N_\mathrm{i}(\ce{e-}) - N_\mathrm{f}(\ce{e-}) $$

With $ N_\mathrm{i}(\ce{e-}) $ representating the number of electrons in a free atom, and $ N_\mathrm{f}(\ce{e-}) $ the one after separation (One should not forget the lone pairs).

One will end up with the following oxidation states for the different (nitrogen) atoms, with the last entry representating the mean oxidation state of all nitrogen atoms:

$$\begin{array}{cccc} \hline \text{Atom} & N_\mathrm{i}(\ce{e-}) & N_\mathrm{f}(\ce{e-}) &\text{Oxidation state}\\ \hline \ce{H} & 1 & 0 & +1\\ \hline \ce{N-1} & 5 & 5 & 0\\ \ce{N-2} & 5 & 5 & 0\\ \ce{N-3} & 5 & 6 & -1\\ \hline \ce{N} & - & - & -\frac{1}{3}\\ \hline \end{array}$$

And the structure with assigned oxidation states:

The oxidation states of the target molecule

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.