I know that experimentally, the rule has been verified but I am having trouble understanding why it works. I see no reason why equivalent cyclic structures can undergo resonance and be just as stable. Any clarification would be nice.
$\begingroup$
$\endgroup$
5
asked May 6, 2015 at 0:35
-
1$\begingroup$ Look at this earlier answer, the "Edit: Huckel's Rule: Aromaticity - Antiaromaticity" section may provide what you are looking for. $\endgroup$– ronCommented May 6, 2015 at 2:22
-
$\begingroup$ The short version is that "antiaromatic" compounds would have two unpaired nonbonding elections (see the Frost Circle in ron's link), meaning that they would be diradicals. Other "antiaromatic" compounds have electrons in antibonding orbitals, counteracting the "resonance" stability. $\endgroup$– Ben NorrisCommented May 6, 2015 at 2:29
-
1$\begingroup$ Maybe also the first part of this answer of mine which gives some electronic explanation where the stabilization associated with aromaticity comes from might be of interest to you. $\endgroup$– PhilippCommented May 6, 2015 at 5:50
-
$\begingroup$ onlinelibrary.wiley.com/doi/10.1002/jcc.20470/full $\endgroup$– MithoronCommented May 6, 2015 at 18:22
-
$\begingroup$ Do have problem with H. Rule specifically or aromacity generally? $\endgroup$– MithoronCommented May 6, 2015 at 18:26
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
The justification comes from molecular orbital theory. I'm going to analyze the MO diagrams for benzene (a molecule with $4n+2$ π electrons) and an MO diagram for cyclobutadiene (a molecule with $4n$ π electrons). These results generalize for all planar cyclic molecules with π orbitals on every atom.
The above π MO diagram of benzene may be obtained through Hückel theory (see also: Pi molecular orbitals of polyenes). Here, the "zero" of energy (referred to as $\alpha$ in Hückel theory) refers to the energy of an isolated carbon 2p orbital that does not participate in bonding. Hence, any orbitals below this energy are bonding in nature, and orbitals above it are antibonding.
In the benzene MO diagram above, you'll notice that all the molecular orbitals that are being filled by the π electrons are more stable than leaving the carbons unbonded. On top of that, it can be shown that the stabilisation derived from delocalised π bonding in benzene is greater than the stabilisation derived from simply forming three localised C–C π bonds, in conjugation with one another, but not delocalised over the entire ring (e.g. in hexa-1,3,5-triene). This is the condition known as aromaticity.
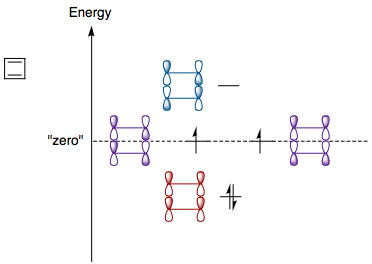
Let's look at the MO diagram for a hypothetical perfectly square cyclobutadiene, for contrast.
As with all molecular orbitals, the most stable orbital is filled first. However, problems start to emerge when going up to next two orbitals. First, unlike the next orbitals in sequence for benzene, these are formally nonbonding within simple Hückel theory.
It can be shown that this situation is less stable than the case for an alternative version of cyclobutadiene, where the double bonds are localised between two adjacent carbons. In practice, cyclobutadiene therefore undergoes a distortion away from a square geometry to a rectangular geometry, which has longer C–C bond lengths for the single bonds and shorter bond lengths for the now localised double bonds (see also: Cyclobutadiene - Jahn–Teller effect or not?). This is the condition known as antiaromaticity.
answered Jun 4, 2015 at 15:19
-
6$\begingroup$ I have corrected the orbital diagram. (cc @Martin-マーチン) However, there is a serious problem with your statement that a diradical is necessarily unstable and the corresponding implication that cyclobutadiene is antiaromatic because of that. Dioxygen itself $\ce{O2}$ is a diradical and is perfectly stable. The antiaromaticity of square cyclobutadiene is not because it is a diradical: it is because delocalised cyclobutadiene is predicted to be less stable than a localised version. So, delocalisation does not necessarily equate to stability! $\endgroup$ Commented Jan 7, 2018 at 2:23
-
$\begingroup$ In general aromaticity arises from a comparison between fully delocalised π bonding vs conjugated but not fully delocalised π bonding, and I have opted to also edit the answer to reflect this important fact. If you don't like it you may roll back. $\endgroup$ Commented Jan 7, 2018 at 2:27