5
$\begingroup$

According to the Wikipedia article Triple point of water, the triple point of water occurs at

The single combination of pressure and temperature at which liquid water, solid ice, and water vapor can coexist in a stable equilibrium occurs at exactly 273.16 K (0.01 °C; 32.02 °F) and a partial vapor pressure of 611.657 pascals (6.11657 mbar; 0.00603659 atm).

Shown in the illustration below (from the Wikipedia article):

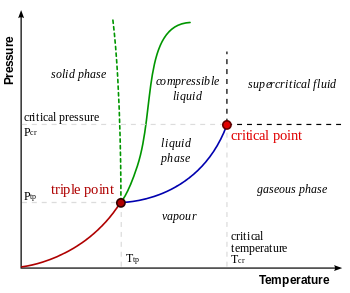
enter image description here

Crucially, to put this into a context, the 611.73 pascals quoted in the article corresponds to the

minimum pressure at which liquid water can exist. At pressures below the triple point (as in outer space), solid ice when heated at constant pressure is converted directly into water vapor in a process known as sublimation.

Potentially useful information to determine if an exoplanet can possibly have water (if all other conditions are suitable).

What are the spectroscopic signatures that differentiate triple state water from water at 1 atm?

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
3
$\begingroup$

The triple point of water is point of dynamic equilibrium between three phases of water. Any small change in Pressure or Temperature will lead to one phase regions or two phase lines on the phase diagram.

The triple point is not a state of matter rather a combination/mixture of three phases . So i very much doubt we can get any unique spectroscopic signatures . We would get a distorted result as this point has three different phases. Also Emission and Absorption spectra depends on temperature and pressure.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Great answer (+1), a question though - would the distorted result be 'characteristic' enough to be differentiated from water at 1 atm? $\endgroup$
    – user15489
    Commented Apr 27, 2015 at 10:39
  • 1
    $\begingroup$ water will have specific peaks at 1 atm and room(or experimental) temperature. But when we have three phases together like this , we will be seeing random peaks (i think). So we can get the spectra of the individual phases by spatial and spectral seperation of components. $\endgroup$
    – Gowtham
    Commented Apr 27, 2015 at 11:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.