Clemmensen reduction of 1,3 diketones

Question

As part of a synthesis I was considering the following reaction:

How selective is the Clemmensen reduction? I know that this reduction favours ketones adjacent to benzene rings but will it also reduce the other ketone, and/or affect any other parts of the molecule. Are there any other methods that would achieve this transformation more effectively.

Answer 1

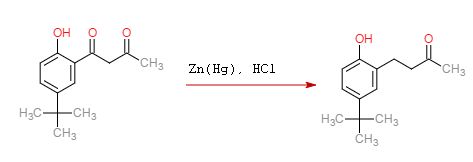
A Clemmensen reduction of a 1,3-diketone probably isn't the best idea. The reduction is likely to proceed via a ketyl radical.

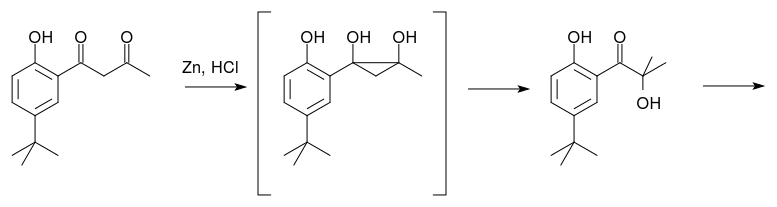
In the case of a 1,3-diketone, formation of a dihydroxy cyclopropane, which undergoes opening/rearrangement cannot be excluded.

This reaction has for instance been observed for dimedone, where 2,4,4-trimethyl cyclopentanone was formed.

EDIT 1

Which other viable procedures exist?

If you insist on using the given starting material, the following might (!) work:

1. regioselective (asymmetric) catalytic hydrogenation of the acetophenone-type carbonyl using a 1,2-diamine-substituted Noyori catalyst

2. Barton-McCombie reaction of the resulting alcohol

If you are rather interested in the product, you might want to rethink the strategy:

The intended product is an arylbutanone, related to the raspberry ketone. A possible retrosynthesis would be:

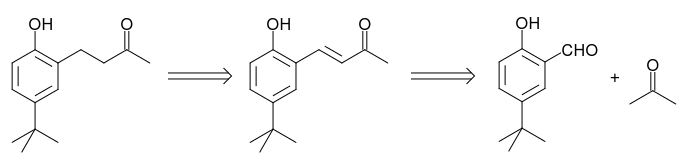
If you get your hands on the necessary benzaldehyde, an aldol reaction with acetone, followed by catalytic hydrogenation might give the right product.

EDIT 2

5-tert.-butyl-2-hydroxybenzaldehyde is commerically available, but not really cheap.

However, 4-tert.-butylphenol is cheap as dirt. In case you need significant quantities of your final product, you might want to consider the synthesis of the benzaldehyde. The substitution pattern cries for a Reimer-Tiemann reaction.