2
$\begingroup$

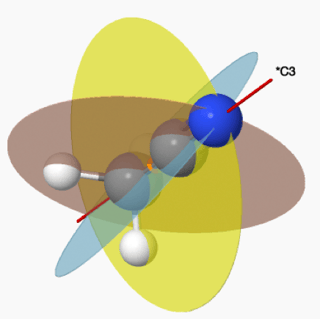
Methyl chloride belongs to the $C_{3\mathrm{v}}$ point group. This is because it has a $C_3$ axis down the $\ce{Cl-C}$ bond.

However what I do not understand is how it has three vertical mirror planes? It has three planes down each of the $\ce{C-H}$ bonds, but don't these bisect a bond making them dihedral planes? Although I'm wrong I can't fathom why it isn't a $C_{3\mathrm{d}}$ point group. I have used a molecular viewer to visualise it but I still get the vertical mirror planes bisecting a bond...

Improve this question
$\endgroup$
2
  • $\begingroup$ Confusingly, C3d groups don't exist in the nomenclature I learned. The "v" subscript is used for C groups and "d" is used for D groups, but the difference between the notations (or for that matter, the exact distinction between a vertical and dihedral reflection) has never been made clear to me. $\endgroup$
    – chipbuster
    Commented Nov 10, 2014 at 20:45
  • $\begingroup$ In principal, the "vertical" mirror planes go along bond axes, and the "dihedral" mirror planes go between bond axes. (Consider, for example an octahedral complex.) In practice, $C_n$ point groups don't have dihedral "d" mirror planes, and plenty of exceptions exist (e.g., ferrocene, where the mirror planes are both "d" and "v" at the same time). $\endgroup$
    – Geoff Hutchison
    Commented Nov 11, 2014 at 3:24

3 Answers 3

Reset to default
6
$\begingroup$

You've correctly identified the $C_3$ axis that contains the $\ce{C-Cl}$ bond. Methyl chloride also has 3 planes of symmetry that contain the $C_3$ axis and each of the $\ce{C-H}$ bonds. Since these planes contain the $C_3$ axis they are referred to as $\sigma_{v}$ planes of symmetry. There are no other symmetry elements in methyl chloride so it belongs to point group $C_{3v}$ (n $\sigma v$ planes plus a $C_n$ axis).

There is no $C_{nd}$ point group. There is a $D_{nd}$ point group which contains a $C_n$ axis, n $\sigma_{v}$ planes (so far methyl chloride fits the description), but also n $C_2$ axis. Methyl chloride does not contain any $C_2$ axes, so it does not belong to the $D_{nd}$ point group.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you very much for your answer. I think I understand now! The $n$ $C_2$ axes would have to be perpendicular to the principal axes right? $\endgroup$
    – RedPen
    Commented Nov 11, 2014 at 13:09
  • $\begingroup$ Yes, that correct. If you want to apply it to some molecules, allene is $D_{2d}$ and the chair form of cyclohexane is $D_{3d}$. $\endgroup$
    – ron
    Commented Nov 11, 2014 at 14:06
0
$\begingroup$

If I remember my symmetry correctly then a dihedral plane by definition is a vertical plane that bisects a perpendicular $C_2$ axis. Since we have a perpendicular $C_2$ axis then I think that would mean you label it $D_n$.

In this case there are no perpendicular $C_2$ axes so I think for that reason they are just vertical planes until such time that you they bisect a perpendicular $C_2$.

I don't know what would be the case if you had $C_n$ on the principal axis and then $m$ perpendicular $C_2$ axes, where $m\neq n$, maybe someone could shed some light? My guess would be that it wouldn't be $D$ but would qualify for the $\sigma_d$ plane.

Improve this answer
$\endgroup$
1
  • $\begingroup$ You can't have $m$ perpendicular $C_2$ axes where $m \neq n$ unless we're talking about a highly symmetric point group, like $I_h$ or possibly $T_h$.. I'd have to think about those. $\endgroup$
    – Geoff Hutchison
    Commented Nov 11, 2014 at 3:26
0
$\begingroup$

The picture shows the vertical symmetry planes along the principal $\ce{C3}$ axis in the $\ce{C_{3{\mathrm{v}}}}$ molecule $\ce{CH3CN}$ which has a $\ce{CN}$ group instead of Cl.

acetonitrile

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.