Why is proton coupling through bonds and how does this affect identical protons?

Question

I had the opportunity to work with a NMR spectrometer and decided to learn some of the theory behind it. After finishing Clayden's chapter on $^{1}\textrm{H}$ NMR (Ch. 11) I'm still left with some questions, mostly concerning coupling.

Proton coupling is described as the interaction between neighboring (2-4 bonds away) protons magnetic field. This can split the signals by a constant amount (the coupling constant), which depend on the environment of the protons but not on the strength of the magnetic field. Ideally creating a binomial distribution when the neighboring protons have the same shift.

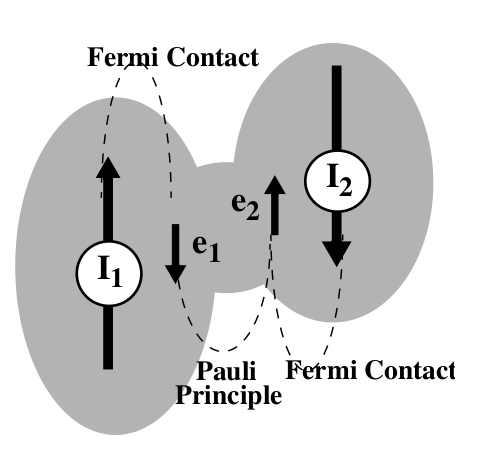
• How does a proton create a magnetic field that influences the neighboring protons (mainly) through bonding and not through space?
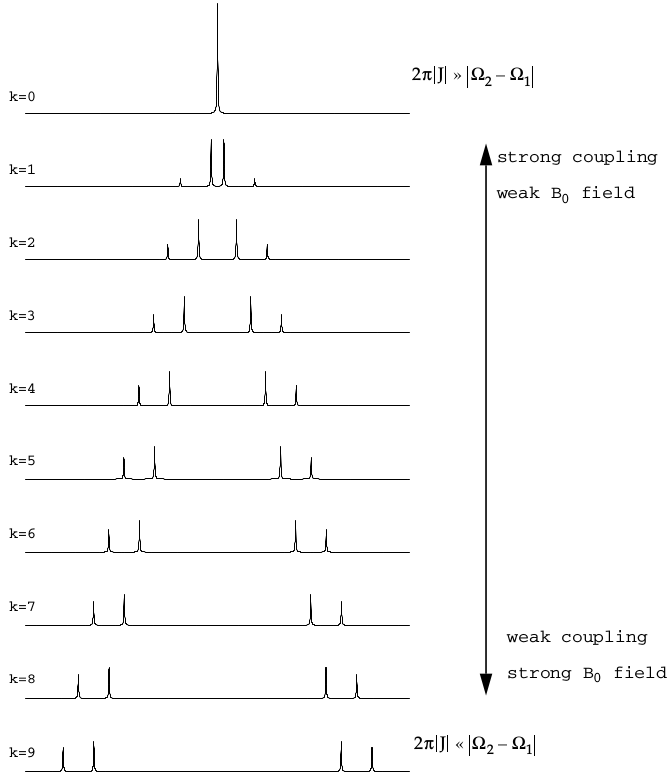
• Why do identical protons not influence each other? Or more generally: why is it that the more identical the influencing protons are with the influenced proton (i.e. $\Delta\delta$ getting equal to/lower than $J$), the more their "outer" signals vanish? Especially since $J$ is a constant, I would expect that for example two doublets would just merge into one doublet and not into only a singlet.

Answer 1

How does a proton create a magnetic field that influences the neighboring protons (mainly) through bonding and not through space?

Through space coupling is known as dipolar coupling and is actually much greater than through bond coupling (J-coupling). However, in low viscosity liquids the chemical shift effects of dipolar coupling are averaged to essentially a single value by molecular rotation. Relaxation effects of dipolar coupling are still observed.

Why do identical protons not influence each other?

For two protons there will be four states $\alpha \alpha , \alpha \beta , \beta \alpha$, and $\beta \beta$. In the general case, each state may have a different energy and there can be four possible one quantum transitions ($\alpha \alpha$ to $\alpha \beta$, $\alpha \alpha$ to $\beta \alpha$, $\alpha \beta$ to $\beta \beta$, and $\beta \alpha$ to $\beta \beta$), each transition having a unique energy and hence 4 peaks (two doublets).

However, when the two protons are equivalent, $\beta \alpha$ and $\alpha \beta$ will be the same energy, $E_2$ let's say. So, letting the energy of $\alpha \alpha$ be $E_1$ and $\beta \beta$ be $E_3$, and given a one quantum transition, the possible energies for transtions are only $E_3$ - $E_2$ and $E_2$- $E_1$. But $E_3$ and $E_1$ are equally spaced above and below $E_2$, so there is only one energy of transition.

For a more-rigorous explanation see: http://www.chemie.uni-hamburg.de/nmr/insensitive/tutorial/en.lproj/energy_levels.html

Answer 2

How does a proton create a magnetic field that influences the neighboring protons (mainly) trough bonding and not trough space?

A proton doesn't interact with another proton by producing a magnetic field per se, but it interacts via the bonding electrons via the Fermi contact interaction. And because the Wikipedia article is kind of dry on this matter, here a figure that very well illustrates what is going on:

This interaction is not the only source for indirect coupling of spins. If we only consider protons, then we're fine. But if you'd like to consider fluorine-fluorine couplings, you also have to take into account the dipolar interaction between the nuclear and electron spins. This dipolar interaction also provides a source for anisotropic $J$ interactions.

---

Why do identical protons not influence each other? Or more general: why is it that how more identical the influencing protons are with the influenced proton (i.e. $\Delta\delta$ getting equal to/lower than $J$), the more their "outer" signals vanish? Especially since $J$ is a constant, I would expect that for example two doublets would just merge into one doublet and not into only a singlet.

Also here, a picture is worth more than a thousand words (I'll spare you the calculation of this spectrum):

The $k$ is defined as follows: $$ k = \left| \frac{\Omega_2 - \Omega_1}{2\pi J} \right|$$

As you can clearly see, the outer peaks start getting smaller the smaller the difference in chemical shift ($\Omega$) of the two spins tends towards zero. This is sometimes called "roof effect".

The intensity of the left- and rightmost lines can be calculated (assuming $J>0$) with the following formula: $$ I_{13} = I_{34} = \frac{1 - \sin(2\alpha)}{4} $$ where $$ 2\alpha = \tan^{-1}\left(\frac{2\pi J}{\Omega_1 - \Omega_2} \right) $$