Are C3 and S3 operations equivalent for C3h point group?

Question

I'm currently an undergraduate student studying inorganic chemistry. The $C_\mathrm{3h}$ point group contains the symmetry operations $\{E$, $C_3$, $C_3{}^2$, $\sigma_\mathrm h$, and $2\,S_3\}.$ I'm a bit confused since I think performing an $S_3$ to a molecule in the $C_\mathrm{3h}$ point group is just similar to performing a $C_3$.

Is this because of one of the properties of a mathematical group is having closure? Meaning, performing a $\sigma_\mathrm h \cdot C_3$ has no other equivalent symmetry operation in the $C_\mathrm{3h}$ point group, therefore, making $S_3$ a symmetry operation of the point group? Or have I understood this differently?

Answer 1

$C_\mathrm{3h}$ is a rare point group for actual molecules. The most commonly shown example is boric acid in this conformation:

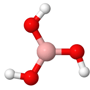
I'm a bit confused since I think performing an $S_3$ to a molecule in the $C_\mathrm{3h}$ point group is just similar to performing a $C_3$.

If you look at boric acid, it does seem to amount to the same. Just like the $E$ and the $\sigma_\mathrm h$ seem to be the same, because neither moves any atoms (i.e. all atoms lie on the mirror plane, and they are idealized as mathematical points even though they contain electrons, protons and neutrons).

To illustrate the difference, here is a more complicated molecule in a $C_\mathrm{3h}$ conformation:

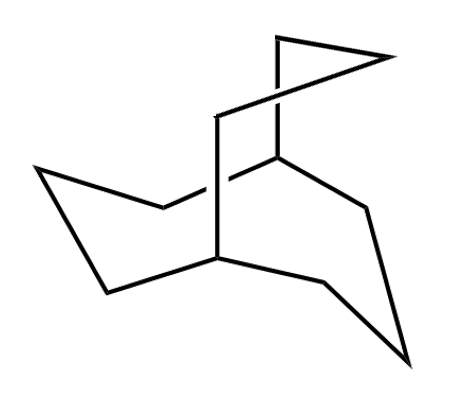
The $C_3$ axis goes through the two bridgehead carbon atoms. Now the $E$ operation does not swap any atoms (it never does), but the $\sigma_\mathrm h$ operation swaps the two bridgehead carbon atoms (and other pairs related by the mirror operation), but not the three carbons "at the tips" because they lie in the mirror plane.

Once you are comfortable with this, you will also see that carbon atom labels get swapped between sides of the mirror plane for the $S_3$ operations, but not for the $C_3$ operations.

Is this because of one of the properties of a mathematical group is having closure?, meaning, performing a $\sigma_\mathrm h \cdot C_3$ has no other equivalent symmetry operation in the $C_\mathrm{3h}$ point group, therefore, makes $S_3$ a symmetry operation of the point group? Or have I understood this differently?

In general, you can either list a minimal set of operations that make up a group (and get the rest by applying operations multiple times and by mixing and matching operations), or list them all. You listed them all. $C_3$ and $\sigma_\mathrm h$ would be sufficient, e.g. applying $C_3$ once, twice or three times yields $C_3$, $C_3{}^2$ and $E$, and combining $C_3$ and $\sigma_\mathrm h$ gives $S_3$. That does not mean the $C_3$ and $S_3$ are the same, they are distinct.

Answer 2

In this D3h molecule ( Co(en)$_3^{3+}$ ) the C3 and S3 axis is shown with the mirror plane. You can see that a C3 rotation moves N5 to N3 but that S3 moves N5 to N6 and so on for the other atoms so these operations are not the same. See molecule-viewer.com for other examples (the site is a bit old looking now but still works ok)

I should add that any symmetry operation in the point group leave the molecule in an indistinguishable state (but not necessarily identical) to that before the operation was performed.