Distance of Orbitals from Nucleus relation with Energy level and penetration power

Question

I have studied that according to Aufbau rule the energy of subshells is dependent on the sum of $ n $ and $l$ values. This would imply that the energy of subshells in a shell varies as

$$ ns \lt np \lt nd \lt nf $$

and this makes sense (intuitively from Bohr model) too as the closer the electron is to the nucleus the lesser energy it would have. And this idea corresponds to penetration power as well where the trend is $$ nf \lt nd \lt np \lt ns $$ as farther the subshell is from nucleus lesser it experiences its force and hence lesser penetration power. Everything made sense until I saw the graphs of Radial Probability Distribution.

and also the expression for average radius of subshell $$ 1/2(3n^2 - l(l+1)) $$ According to these both my conclusions about penetration power and energy levels should be wrong as for greater $l$ value average radius and even most probable radius both are smaller than corresponding values of smaller $l$ values. What is wrong with my reasoning?

Answer 1

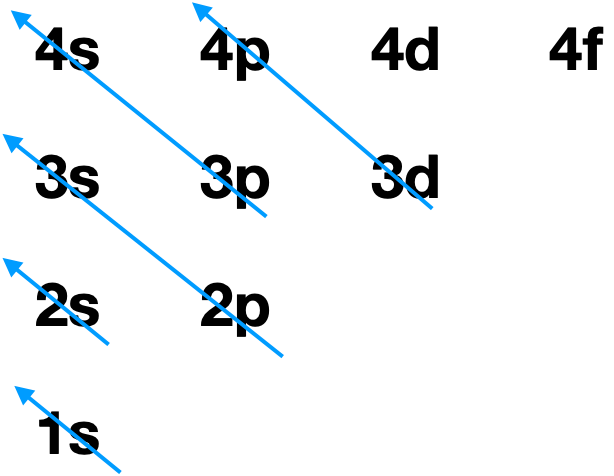
I have studied that according to Aufbau rule the energy of subshells is dependent on the sum of $n$ and $l$ values. This would imply that the energy of subshells in a shell varies as

$$ n_s<n_p<n_d<n_f $$

** Let us try a few examples, from the picture diagram. **

$ 1 S : n=1, l=0; n+l = 1$

$ 2 S : n=2, l=0; n+l = 2$

$ 2 P : n=2, l=1; n+l = 3$

$ 3 S : n=3, l=0; n+l = 3$

Here is a surprise, different from your understanding. For the $ 2 P $ and $ 3 S $ examples, the sum of $n+l$ is equal instead of the increasing of order that you had expected.

$ (n+1)_{2P} = 3 = (n+l)_{3S} $

And this makes sense (intuitively from Bohr model) too as the closer the electron is to the nucleus the lesser energy it would have. And this idea corresponds to penetration power as well where the trend is

$ n_f < n_d <n_p < n_s $

Well that quoted statement needs to be checked carefully. Unfortunately a little bit of background in Quantum Mechanics and the Schrödinger equation are needed for a more intuitive understanding of what is going on.

There are a few different forms of the Schrödinger equation. To fundamentally understand electron-ionization of atoms, the time-independent form is most useful 2 :

The simplest way to write the time--independent Schrödinger equation is $H\psi = E\psi$, however, with the radially-symmetric Hamiltonian operator expanded it becomes:

$\sum_i{(-\frac{\hbar^2}{2m_e} \frac{d^2 \psi_i}{dr_i^2})} + \sum_i{V_i\psi_i} = E_i\psi_i\\ $

The left-hand-most side of the equation is calculated from momentum contributions (of the wave-functions themselves) versus radius. In Quantum Mechanics, the electron can be found anywhere when it is ionized with some probability, but until it is ionized is is found no-where. There is just some probability that it it exists at any particular place to enable wave-function expected-energy calculations. The wave-function, in short, corresponds to a probability distribution for each $i_{th}$ electron.

On the left-side is also a sum of the wave-function multiplied by a potential function that accounts for the energy that the electron has because of the protons at the nucleus or because of other electrons.

To get to understand this potential (labeled as $V$ in the equation), there are a couple of interactions that are important, for the moment I will describe for Helium, for you to generalize to other atoms:

• The potential of the inner-electron due to the two protons in the center of the nucleus and its probability density. (This well is so deep that the wave-function of the inner electron is not much influenced by the outer-electron. For a good first calculation of ionization energy, this inner-electron wave-function can be assumed to not change at all.)
• The potential of the outer-electron including the shielding of the nucleus by going to a value of $e^{2+}$ to $e^{1+}$ because the inner electron has a shielding value of $e^{1-}$. Ironically, for a basic good calculation, the outer-shielded-electron wave-function does not change very much either, and for a first start, this change can also be entirely neglected.
• The interaction of the inner electron and outer electron as a coulomb correction to the Schroedinger model for Hydrogen, which accounts only for a point-source nucleus versus a spread-out expectation of the electric field and the expectation of the outer electron charge density integrated (on the entire volume).
• For this Coulomb interaction of the inner electron and outer-electron, the inner-electron wave-function is that from $e_{2+} hydrogen$ and the outer-electron wave-function is that from $e_{1+} hydrogen$.

Thus regarding:

as farther the sub-shell is from nucleus lesser it experiences its force and hence lesser penetration power. Everything made sense until I saw the graphs of Radial Probability Distribution.

The component of the Schrödinger equation that deals with the nuclear attraction is the fully-shielded atom, because the outer-electron that is ejected for the first-ionization is always out-most. However, the near-field Coulomb energy contribution changes quite a bit as the electrons are pushed further and further from each-other, as is shown in the below diagram.

Outer-most Ionization Energies:

.

Like you stated, we can expect that for higher element-numbers in the Periodic-Table, as a general trend, the outer electron ionization energy is lower because the inner-electron-shielding is more complete.

I have not yet plotted out your diagrams, but instead I think this reference about Hydrogen Wave Functions might be more-complete.

One of the illustrations is that reference 6 is very much easier to understand:

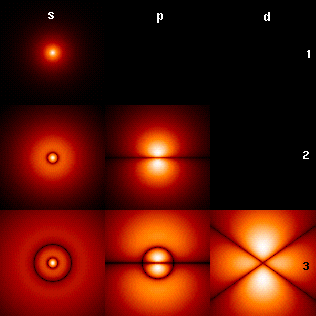
Also, I found for you this reference websites.umich.edu/~chem461/QMChap7.pdf. It also has the linear plots in it with a more detailed explanation about how to calculate the probability distributions which should be more insightful than the previous diagrams you referenced.

From the reference Hydrogen Electron Density Versus Radius R, there is a plot on page 19:

It does seem that the expected radius for the $3 S$ - wave-function is greater than the expected-radius for the $3 P$ - wave-function, which is also apparently greater than the expected-radius for the $3 D$ - wave-function. So the expected trend from your plots seem somewhat confirmed, in terms of expected radius. The problem with your understanding is that expected radius for Hydrogen is not the only thing determining the first ionization potential. Indeed for Hydrogen, only the quantum number $n$ determines potential ionization energy, independent of $l$ and $s$ quantum numbers. The plots you have seem to be similar (in trends) to those for pure hydrogen wave-functions, all with expected energy just from $n=3$ and nothing else, making the ionization energies for the three first ionization energies exactly the same because of other effects, such as distributions versus spherical coordinates (aside from the radial coordinate $r$).

Answer 2

The Aufbau Principle reference is summarized with the following picture:

Keep in mind that the Aufbau principle is founded on 1) the energy levels of Hydrogen, which are only determined by the quantum number $n$. But that is not the entire picture. Because of the shielding effect as well as near-nucleus penetration, there is an additional perturbation that comes in to play.

I have calculated this for Helium, and I discovered that the binding energy taking into account this addition perturbation could be calculated with around $3$ $Percent$ $accuracy$ which I considered a great success. There are two electrons in this model, an inner electron and an outer electron. For Helium, the inner electron is so closer to the nucleus, and even as the radius approaches infinity, it is still inner, meaning that it bears the $2e^+$ charge attraction. And the outer electron, even as the radius approaches infinity is still outer, meaning that it bears $1e^+$, being shielded by the inner electron.

The two spins are opposite for Helium for the inner and outer electron, so there is no major problem with spin interaction. The perturbation comes almost entirely from the effect of the outer electron penetrating into the shielding of the inner at the near field, close to the nucleus.

So the Aufbau Principle also considers this shielding perturbation of energies to arrive at an accurate ordering of shells, which makes it different than the pure Hydrogen calculations that just depend on the quantum number $n$.

There are a couple of other considerations. First of all, when the Aufbau Principle references the $2$ $P$ $Orbital$, it is really a hybridized orbital, which is some combination of $2$ $S$ and $2$ $P$ orbitals (which for idealized Hydrogen have instead equal energy levels). This hybridization pushes the $S-P$ orbital outward, with more focus. This same hybridization effect increases with $S-P-D$ hybridized orbitals and so forth.

Taking account with the VESPR model, which includes this Hybridization-focusing effect of electron bonds, one can see that as that from the order of $1S$ which is spherically symmetrical, going on to $2S, 2P$ which has 4-fold symmetry, that the electrons of that level are able to distance themselves out further from one another in the near field to the nucleus. The Aufbau Principle is a kind of short-hand notation, labeling $2P$ as what is really a $2S-2P$ hybridized orbital that allows for this additional separation. The additional separation in the near-field to the nucleus of the electrons allow for lower interaction energy there.

This is my understanding of how all of this works. I am welcome to additional insight and comments, as I have not yet gone through the calculations for Lithium and so forth, which I estimate are not hard calculations, just tedious ones.

Answer 3

Where did you get this diagrams from?There is something definitely suspicious with your diagrams.The more the wavefunction of a particle changes over r the more energy the particle has.From your diagrams obviously the "3s" wavefunction has more energy than the other two because it has more valleys and troughs which according to the Aufbau principle cannot be true.

Also if you look at some of your diagrams at some points the wavefunction $\Psi(r)$ takes a value $>1$ which isnt possible as well.