11
$\begingroup$

I came across a Frost diagram for cyclic compounds in my book, and all my book had to offer was that it geometrically reproduces the solutions of the wave equation, and can therefore determine the relative energies of each pi molecular orbital.

How, exactly, does a Frost diagram work? My book was of no use.

enter image description here

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ I'm really confused. I'm learning about Frost Diagrams as well in class. But they look completely different than what you just posted. Are these the same? $\endgroup$
    – John Snow
    Commented Sep 27, 2014 at 1:33
  • 3
    $\begingroup$ @JohnSnow I believe it's the same Frost (Arthur Atwater). He has two Frost diagrams. The one discussed here is related to organic MO theory; the one you referenced relates to electrochemistry. $\endgroup$
    – ron
    Commented Sep 27, 2014 at 2:30

2 Answers 2

Reset to default
12
$\begingroup$

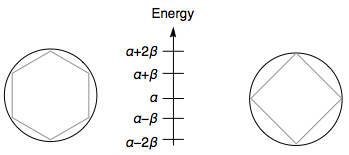
Algebraically, the levels of cyclic polyenes may be derived using simple Hückel theory (see also: Pi molecular orbitals of polyenes). The general result for the energy of the $j$-th level for a cyclic system containing $N$ atoms is:

$$e_{j} = \alpha + 2 \beta \cos\left(\frac{2j\pi}{N}\right)$$

where $\alpha$ is the energy of each carbon $\mathrm p_{\pi}$ orbital before interaction (Coulomb integral), $\beta$ is the interaction energy between two adjacent $\mathrm p_{\pi}$ orbitals (the resonance integral) and $j= 0, \pm 1, \pm 2,\ldots, \pm \frac{N - 1}{2}, +\frac{N}{2}$ for even $N$, and $j= 0, \pm 1, \pm 2, \ldots, \pm \frac{N - 1}{2}$ for odd $N$. The very simple form of this equation leads to a useful mnemonic for remembering the energy levels of these molecules. Draw a circle of radius $2\beta$ and inscribe an $N$-vertex polygon such that one vertex lies at the bottom position. The points at which the two figures touch define the Hückel energy levels. And that is what is called a Frost diagram.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ +1 I hadn't heard of these diagrams until teaching quantum and it was in the book without any derivation. Then I looked up the general result for cyclic polyenes above and it made sense. $\endgroup$
    – Geoff Hutchison
    Commented Sep 26, 2014 at 15:58
  • $\begingroup$ @GeoffHutchison I made a small mistake at the range for $j$ which should be correct now. The Frost circle is indeed a pretty neat example for very useful and pictorial (and historical) chemical rules/mnemonics/concepts that while being very easy give a lot of intuition for how chemical compounds will react and what properties they have. I find it very interesting to discover the actual underlying physical theories and approximations that lead to these rules and to see when they are valid and when they should be used with caution. $\endgroup$
    – Philipp
    Commented Sep 26, 2014 at 22:30
10
$\begingroup$

Frost developed this mnemonic patterning as an extension of the Hückel ($4n+2$) rule. A Frost diagram is usually applied to all-carbon, monocyclic, π systems. It allows one to find the number of molecular orbitals in the molecule's π system and their energetic positions. To construct a Frost diagram, proceed as follows:

Example Frost diagrams

  • Draw a circle and inscribe a regular polygon with a vertex located at the bottom of the circle. The polygon has the same shape as the ring you are interested in. For example, if you are interested in benzene, draw a hexagon; for the tropylium ion, draw a heptagon.
  • Energy-wise, the top and bottom of the circle are defined as $\alpha+2\beta$ and $\alpha-2\beta$ respectively (so the circle has radius $2\beta$); the center of the circle is located at $\alpha$; other points can be interpolated accordingly; the bottom of the circle is at lower energy than the top of the circle.
  • Wherever a vertex of the polygon touches the circle, that is the energetic location of a molecular orbital.

Using benzene as an example, the lowest MO has energy $\alpha-2\beta$; the HOMO is degenerate (2 MO's) and located at $\alpha-\beta$; the LUMO is also degenerate and located at $\alpha+\beta$. Any orbital below the center of the circle is bonding, any orbital at the center is non-bonding and any orbital in the top-half of the circle is antibonding.

Improve this answer
$\endgroup$
8
  • $\begingroup$ But why does this work? $\endgroup$
    – Dissenter
    Commented Sep 26, 2014 at 14:31
  • $\begingroup$ It works because it is an extension of Huckel's 4n+2 rule. Since it is related to Huckel's rule, it has its weak points, just like Huckel's rule. $\endgroup$
    – ron
    Commented Sep 26, 2014 at 14:45
  • 3
    $\begingroup$ Huckel's rule only applies to a small, highly-defined class of molecules. For example, it predicts that cyclooctatetraene is antiaromatic; in fact, the molecule distorts from planarity and is stable. Frost predicts 2 degenerate non-bonding MOs at 0 beta for cyclobutadiene; in fact, the molecule distorts and the MO's split. $\endgroup$
    – ron
    Commented Sep 26, 2014 at 15:18
  • 1
    $\begingroup$ Frost-Muselin method is simply a mnemonic to the solution for the secular equations of this very small set of per-cis annulenes. There is no physical reason that one "puts the polygon on its point" and draws energy levels at the intersections. And to add to the discussion about how Hueckel theory is generally wrong for ground state of highest symmetry planar "anti-aromatic" species: they are ground state singlet even before J-T distortion (violations of Hund's rule in molecules) $\endgroup$
    – Eric Brown
    Commented Sep 26, 2014 at 23:41
  • 4
    $\begingroup$ @EricBrown There is no physical reason that one "puts the polygon on its point" and draws energy levels at the intersections. In which sense do you mean that? The equation for the Hückel energy levels, $e_{j} = \alpha + 2 \beta \cos \frac{2 j \pi}{N}$, gives you that equilateral polygon shape and since the level for $j=0$ is always non-degenerate and the lowest-lying level ($\beta$ is negative) its tip will always be at the bottom. Or do I misunderstand you? $\endgroup$
    – Philipp
    Commented Sep 27, 2014 at 1:56

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.