How to relate the conductance of an aqueous electrolyte to its conductivity

Question

In this answer on Physics Stack Exchange, the author states that conductance $G$ is related to conductivity $\sigma$ by $$G = \sigma\frac{A}{l}$$ where $A$ is the cross-sectional area perpendicular to the direction of electric current and $l$ is the length of the conductor.

How does this relate to the conductivity of solutions such as aqueous electrolytes?

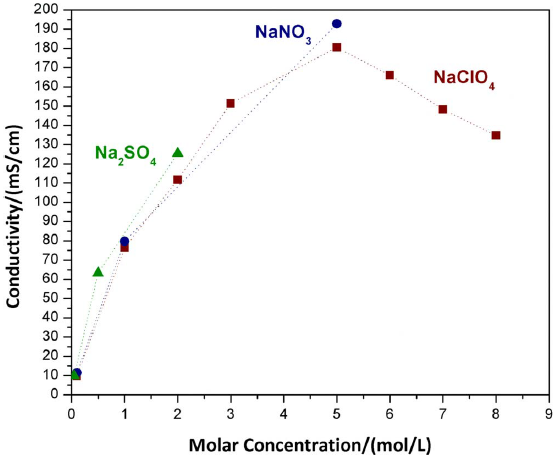
Papers in the literature often give the conductivity of aqueous electrolytes in units of $\pu{S/m}$ or $\pu{mS/cm}$. Here is an example from Wu et al., J. Electrochem. Soc., 2015 where the authors plot conductivity in $\pu{mS/cm}$ versus molar concentration in $\pu{M}$:

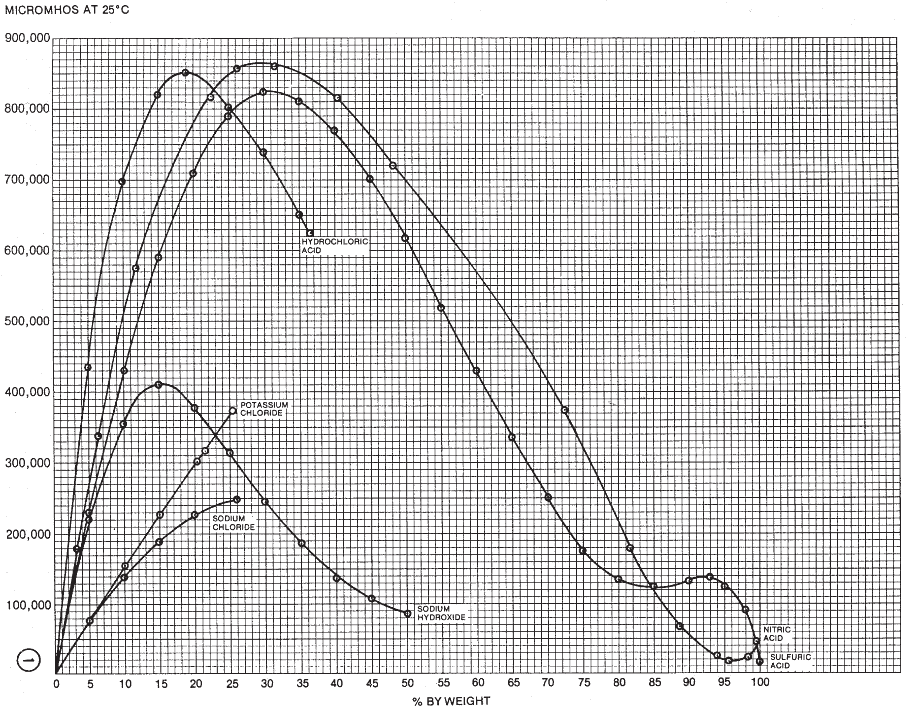
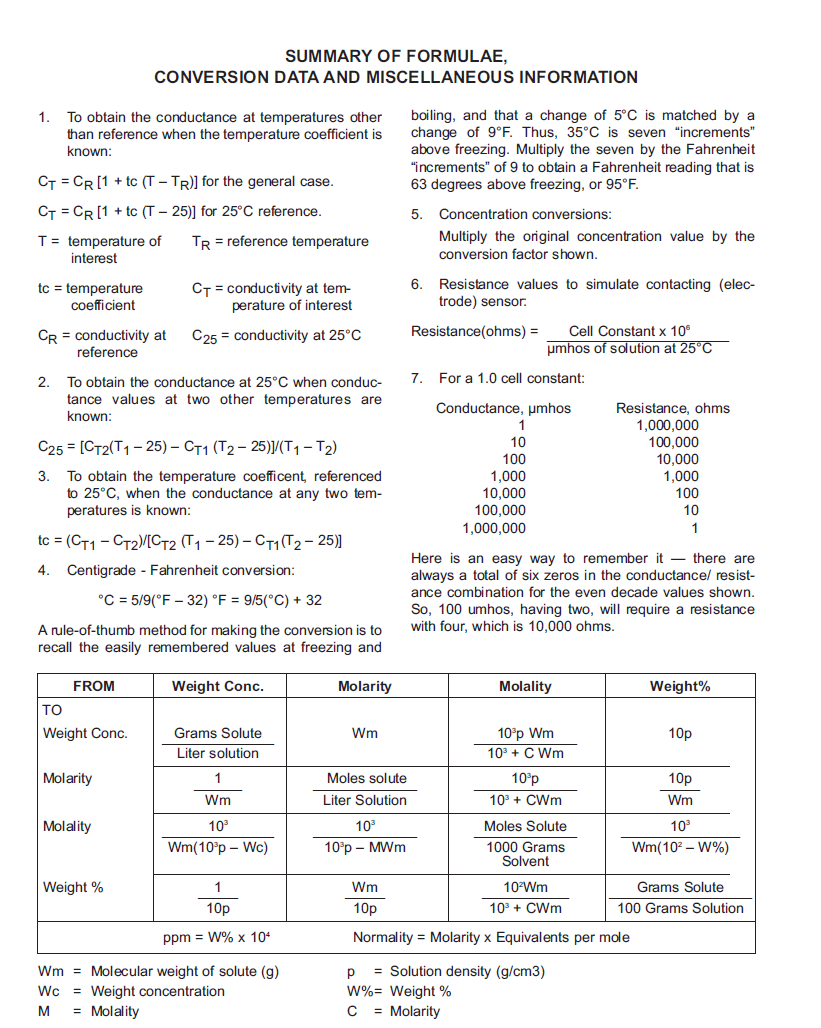
But, in looking for conductivity values for sulfuric acid as a function of molar concentration, I found this report. It states the conductance, rather than conductivity, of aqueous electrolytes (Or so it seems).

On page 7 of that pdf, for example, the authors provide a plot of $\pu{micromhos}$ versus percentage by weight:

I think that $\pu{1 micromho} = \pu{1 S}$. What information would I need to determine the conductivity (in units of, for example, $\pu{S/m}$ or $\pu{S/cm}$) of an aqueous solution of sulfuric acid from the above plot?

Answer 1

The answer is quite simple, if you look at the equation carefully. Conductance and conductivity are related by cell constant, $\displaystyle\frac{\text{Area}}{\text{Distance}},$ where the area is the area of the electrodes and the distance is the distance between the electrodes.

$$G = \sigma\frac{A}{l}$$

In conductivity measurements, the cell constant was either given by the manufacturer or it was determined experimentally by making a certain concentration of $\ce{KCl}$, the conductivity of which was well established and standardized.

In short, you cannot convert micromho to conductivity because you do not know the cell constant. And weight by weight conversion to molarity is not that trivial. You need to know exact density of each solution.

Did you see the end pages of the manual from you posted the old graph, there is a useful chart (for future readers).

Answer 2

In the "Index of Electrolytes" on page 3 of the document at the source of the problem (Ref. 1)

note that the third column is titled "Maximum conductance and point of inflection at 25°C [$\pu{μmhos/cm}$/% by wt.]". However, the entries are given in units of conductivity ($\pu{μmhos/cm}$ or $\pu{μS/cm}$)! The first number for each entry in that column corresponds to the maximum conductivity in units of $\pu{\mu S/cm}$.

For sulfuric acid the entry is

If you look at the plot on page 7, sure enough, the maximum conductance occurs at 30%/wt, when the conductance is 825 mS (unspecified uncertainty but ~$\pu{\pm 3 mS}$) and (according to the table in the Index of Electrolytes) conductivity is 825 mS/cm.

The cell constant therefore has a value

$$C=\frac{\kappa}{G} = \pu{1 cm^{-1}}$$

If you compare values in the Index with those in other plots you'll identify a similar pattern. Therefore, although reported as conductances, the values in these plots can be read as conductivities, the cell constant for conversion between the two values being $ \pu{1 cm^{-1}}$.

References

Note that the source of the data seems legitimate (Ref. 1) and compares well to other (official and unofficial) sources found during a quick web search (e.g. Ref. 3). The plots represent a compilation from various sources specified in the bibliography of Ref 1 and includes International Critical Tables (Ref. 2).

1. Conductance Data For Commonly Used Chemicals. Rosemount Analytical - Emerson Process Management, 44-6039/rev. B December 2010

2. International Critical Tables, Vol. Vl, pp. 230-258; McGraw Hill, 1929.

3. G.W. Vinal, D.N. Craig. Resistivity of Sulphuric Acid Solutions and its Relation to Viscosity and Temperature. Journal of Research of the National Bureau of Standards 13 689-697 (1934).