How to read mutual solubility curves of salts with a common ion?

Question

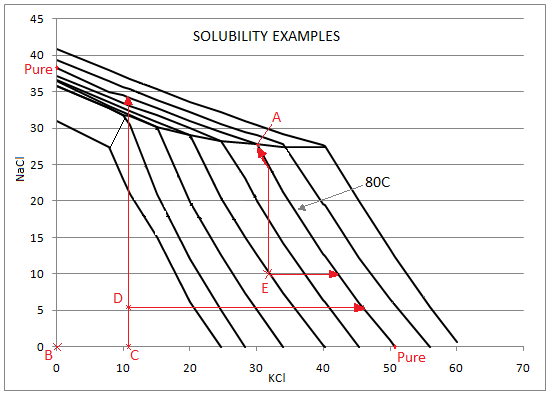
Came across a few graphs that represent the mutual solubility of two compounds in a solvent, for e.g., solubility of $\ce{KCl}$, and $\ce{NaCl}$ in $\ce{H2O}$.

On these particular graphs, I can see $x$-axis as $\pu{g}/\pu{100 mL}$ of $\ce{KCl}$, on $y$-axis as $\pu{g}/\pu{100 mL}$ of $\ce{NaCl}$. And the graph has multiple lines each representing a curve at a specific temperature.

I just wanted to understand how to read these type of graphs:

To me the graphs represent a point wise mechanism. For example, if I pick a spot on the $x$-axis, with $\pu{50 g}$ $\ce{KCl}$ at $\pu{80 ^\circ C}$, does that mean if I dissolve $\pu{50g}$ $\ce{KCl}$ at $\pu{80 ^\circ C}$,then I will not be able to dissolve $\ce{NaCl}$ in it?

Or does it mean if I have a starting condition where I have $\pu{50g}$ $\ce{KCl}$ at $\pu{80 ^\circ C}$, and I start adding $\ce{NaCl}$, then $\ce{KCl}$ will precipitate out at a slow rate till I reach point A at a very rapid rate?

The graph doesn't appear complete, or is at least missing additional info to make sense of it. I want to know what that info is.

Answer 1

My interpretation is:

I assume both cross solubilities are related to the volume of water used for the initial solution, not of the initial solution volume, i.e. both in $\pu{g}/\pu{100 mL}$ of water.

If you start at $x$-axis with the $\ce{KCl}$ solution, you can dissolve $\ce{NaCl}$, climbing upwards until you reach the curve for the given temperature. If you cross it, some $\ce{NaCl}$ will not dissolve, and some $\ce{KCl}$ may precipitate, because of the common ion effect.

The same of the initial $\ce{NaCl}$ solution, starting on $y$-axis, and dissolving $\ce{KCl}$, going to the right, again until you reach or cross the curve.

$\ce{KCl}$ solubility is lower, if some extra $\ce{Cl-}$ ions from $\ce{NaCl}$ are present, because of the solubility product $K_{\mathrm{sp}, \ce{KCl}} = a_\ce{K+} \cdot a_\ce{Cl-}$.

Similarly for $\ce{NaCl}$, $K_{\mathrm{sp},\ce{NaCl}} = a_\ce{Na+} \cdot a_\ce{Cl-}$.

Problem is difficulty with both measuring and predicting ion activities or activity coefficients for concentrated ionic solution. Replacing activities with concentrations could be done only with lot of grains of salt and both eyes closed tightly.

I do not have clear interpretation of the about horizontal edge in the upper chart part. It looks like a kind of "precipitation eutecticum", an analogue to a binary mixture phase chart.

---

Feedback to comments:

If the system is at the cross-saturation curve, and there is excess of one solid salt, then due dynamic nature of processes, this salt dissolves and the other precipitates. The system is moving slightly along the curve, until for each of salts the rates of dissolving and precipitating are equal. This process is slow.

If a cross-saturated solution cools down, both salts precipitate, according to the ratio of kinetic rates of both processes, until the curve for new temperature is reached.

The ratio can be only very roughly estimated from

• the chart position
• the direction of the normal vector of the curve
• the different ion mobility.