3
$\begingroup$

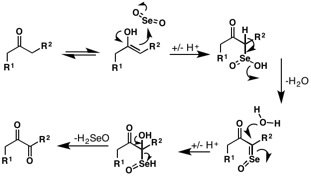
I have searched the whole internet but I could only see examples where the authors of the article took $\ce{R1}$ and $\ce{R2}$ as the alkyl groups around the carbon.
This is regarding the oxidation of carbonyl compounds (I am specifically searching about ketones) using $\ce{SeO2}$.
What I understood was $\ce{C=O}$ got introduced on the alpha-carbon and two hydrogens from that carbon were lost.
I would like to know the product obtained on reacting pentan-2-one with $\ce{SeO2}$. Will the carbon having 3 alpha-hydrogens become $\ce{C=O}$ or will the other one having 2 alpha hydrogens become $\ce{C=O}$?

References:

  1. Selenium dioxide oxidation of ketones and aldehydes. Evidence for the intermediacy of .beta.-ketoseleninic, K. Barry Sharpless and Kenneth M. Gordon Journal of the American Chemical Society, 1976, 98 (1), 300-301 DOI: 10.1021/ja00417a083
  2. https://www.adichemistry.com/organic/organicreagents/seo2/selenium-dioxide-seo2.html
Improve this question
$\endgroup$
2
  • $\begingroup$ See Riley Oxidation $\endgroup$
    – Solid - NMR
    Commented Aug 27, 2020 at 10:51
  • $\begingroup$ You find some insight here. $\endgroup$
    – Mathew Mahindaratne
    Commented Aug 27, 2020 at 16:37

1 Answer 1

Reset to default
3
$\begingroup$

The mechanism of Selenium Dioxide (Riley) oxidation proceeds via the enol tautomer of the ketone (see scheme - image from Wikipedia). If the ketone is assymetric and can enolise to either side then the reaction should give the two di-ketones in proportion to the amount of either enol formed though I cannot find any reference where this has been experimentally demonstrated. In the OP's case of 2-pentanone, a mixture of the two possible products will be formed.

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ why would it not make a double bond as it does for 1,4 diketones? $\endgroup$
    – user98209
    Commented Aug 27, 2020 at 14:34
  • 1
    $\begingroup$ @chemistry.stackexchange.com/questions/33034/…: It's depend on the several factors including structure of starting material, solvent, and pH. You can find an answer to your question here. $\endgroup$
    – Mathew Mahindaratne
    Commented Aug 27, 2020 at 16:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.