I am using the chemistry textbook by Masterton/Hurley (Cengage) as an outline for this answer. It has a side note that reads "Our model for electronic structures is a pragmatic blend of theory and experiment". In the spirit of that quote, there is some experimental data mixed in with the concepts.
Each row on the periodic table has a new shell of electrons
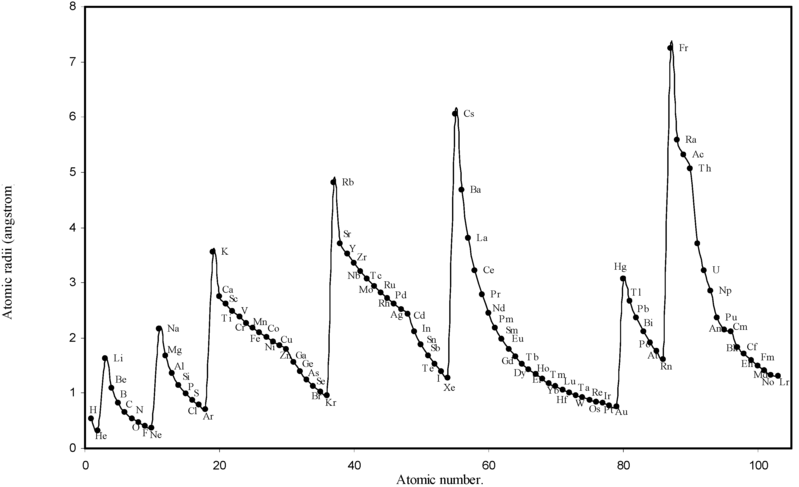 Yes, and you see that in the plot of atomic properties vs. atomic number. For example, atomic radii (image source)increase significantly as you go from noble gas (last element in a period) to group 1 metals (first element with the next shell occupied). The quantum number that defines the shell is the principal quantum number n.
Yes, and you see that in the plot of atomic properties vs. atomic number. For example, atomic radii (image source)increase significantly as you go from noble gas (last element in a period) to group 1 metals (first element with the next shell occupied). The quantum number that defines the shell is the principal quantum number n.
A subshell is e.g. 1s, 2s, 2p, 3s, 3p, etc.
Yes. The periodic table is organized into s-block and p-block (main group elements), d-block (transition metals) and f-block (lanthanides and actinides) according to which subshell is partially filled (or just filled). The quantum number that defines the subshell is the second quantum number, $\ell$.
Are energy levels the same as shells?
This is true only for the hydrogen atom and other one-electron systems ($\ce{He+}, \ce{Li^{2+}}$) in the absence of a magnetic field:
$$ E \propto -\frac{Z^2}{n^2} $$
with $Z$ the charge of the nucleus and $n$ the principal quantum number.
For systems with more than one electron, distinct subshells in the same shell correspond to slightly different energy levels. One method to measure these energy levels is photoelectron spectroscopy, with some data on aluminium 2s and 2p shown below (image source).
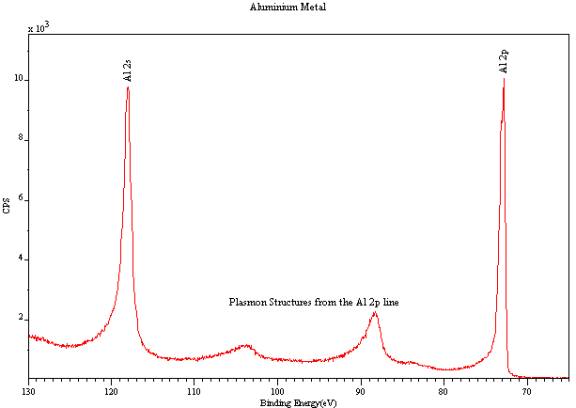
In general, the energy levels depend on the atomic number (number of protons in the nucleus) and the electron configuration (how many electrons are present, and whether they are at ground state or in an excited state). Going from a hydrogen atom to a larger multi-electron atom, the energy levels of distinct shells can be roughly described as an electron bound to a nucleus surrounded by inner electrons with a combined effective charge $Z_\mathrm{eff}$ (smaller than the actual charge of the nucleus because the attraction by the nucleus is offset to a degree by the repulsion by the other electrons):
$$ E \propto -\frac{Z_\mathrm{eff}^{2}}{n^2} $$
For the innermost shells (n = 1), $Z_\mathrm{eff}$ is almost the same as the nuclear charge, whereas for the outermost shells, $Z_\mathrm{eff}$ can be much lower.
For example, comparing 1s electrons across the periodic table, the binding is tighter and tighter as the atomic number increases. On the other hand, the outermost shell (valence shell) in elements of the same group (e.g. Be, Mg, Ca, Sr, Ba, Ra) corresponds to similar energy levels, even though they differ in the principal quantum number. This explains why elements in a group have similar chemical behavior.
Are orbitals the same as subshells?
No, there are seven different orbitals in the f-subshell, five different orbitals in the d-subshell and three different orbitals in the p-subshell (compare size of blocks in the periodic table). The three orbitals in the p-subshell, for example, are either designated (+1), (0), and (-1), or alternatively $p_x$, $p_y$, and $p_z$. For the s-subshell, there is a single orbital, so only in this special case is the subshell the same as an orbital. The quantum number defining the orbital in a subshell is the third quantum number $m_\ell$.
While the different orbitals of the p, d, and f subshells have distinct, sometimes intricate shapes, a filled subshell corresponds to a spherical distribution of electrons.
How do these concepts help to understand chemical bonds?
We study electronic structure because it explains properties and reactivity of atoms and molecules. It might seem that atomic orbitals of hydrogen are of little use in understanding the electronic structure of larger atoms and of molecules. It turns out, however, that they can be used as building blocks for the more complicated systems, both qualitatively (talking about sigma and pi bonds and lone pairs) and quantitatively (computational methods use atomic orbitals as basis functions).
Surprisingly, the changes in electronic structure going from atoms to molecules are moderate, with spherical electron distribution around the atoms explaining most of the electron density observed, for example, in crystallographic studies. The small deviations from this are called electron deformation densities, and they give an experimental view of the subtle shifts in electron density upon bond formation. The picture below shows a contour diagram of the total electron density on the left (near the atoms, there would be many more contour lines, but they are not shown), and the deformation density on the right (lines: higher density than atomic density, dashed: lower density than atomic density, dot-dash: same density as atomic density).
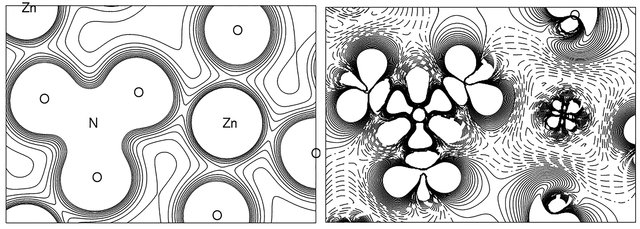 (Image source)
(Image source)



