4
$\begingroup$

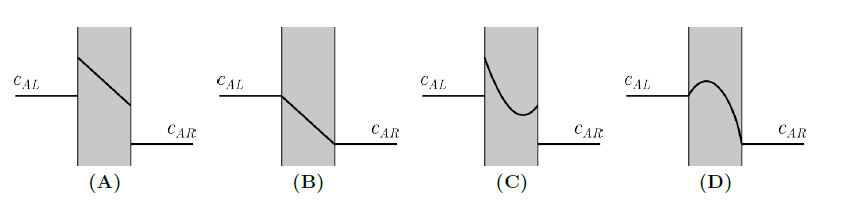
enter image description here

Given this concentration profile, I am to (1) determine at which side substance A will diffuse out of the membrane and (2) chose one of the following profiles below which represents the concentration of a substance A at steady-state.

enter image description here

(1) Diffusion occurs from an area of high concentration to an area of low concentration. Therefore, substance A would diffuse from the left to the right. However, in the question when they ask "at which side will the substance diffuse out of the membrane", should I answer at the right side or that the substance stays within the membrane?

(2) I know that the concentration profile at steady-state should be linear, however I'm wondering what the difference between (A) and (B) is. I assume that (A) is the correct answer to this question since substance A has a higher concentration than the concentrations at the membrane wall and hence the slope should start a little above the concentration at the wall. Is this correct or does steady-state affect the concentration profile of the substance?

Improve this question
$\endgroup$
4
  • $\begingroup$ Is the membrane homogenous? Does the membrane have preferential interactions with the solute compared to the solute:solvent interactions? If the answer is no to both, how about having the solute diffuse out on both sides until a steady state has been reached? $\endgroup$
    – Karsten ♦
    Commented Mar 11, 2019 at 4:25
  • $\begingroup$ @A.K. - Looked at your answer which is much better so I deleted mine. $\endgroup$
    – MaxW
    Commented Mar 11, 2019 at 7:34
  • $\begingroup$ @KarstenTheis - I didn't remember that diffusion according to Fick's law was considered to be a steady state. Since the concentration on both sides of the membrane will be changing until equilibrium, as well as the rate of diffusion across the membrane, I was thinking of that as more of a quasi-steady state. // My imagination got the better of me and I was wondering about something like an ion-pumping biological membrane to get the weird hyperbolic profile. $\endgroup$
    – MaxW
    Commented Mar 11, 2019 at 7:39
  • $\begingroup$ If it is a real system, it probably is quasi-steady state. I don't have a good example of a real steady state system one could actually accomplish, but you can come close by making a membrane with a small cross-section and well-stirred very large reservoirs on both sides. $\endgroup$
    – Karsten ♦
    Commented Mar 11, 2019 at 17:23

1 Answer 1

Reset to default
3
$\begingroup$

(1) determine at which side substance A will diffuse out of the membrane

should I answer at the right side or that the substance stays within the membrane?

Neither, because the concentration is higher in the membrane than either side, the substance will flow out of both sides initially at least. After a while the material will flow from left to right (high concentration to low concentration) and converge towards steady state.

(2) chose one of the following profiles below which represents the concentration of a substance A at steady-state.

First thing - When doing diffusion problems immediately refer to Fick's laws of diffusion.

Fick's first law assumes a steady state system and is given as:

$$J = -c D\frac{dx}{dz}\quad \text{or more simply} \quad J = -D\frac{\Delta C(x)}{\Delta z} \quad \text{or more generally}\quad J = -cD\ \nabla x$$

Where $J$ is flux (mols through an cross-sectional area per time), $c$ is total concentration, $C(x)$ is the concentration at point $x$, $D$ is the diffusion constant, $x$ is the mole fraction and $z$ is position.

For steady state to occur, $J$ must by definition be constant through the entire membrane and thus $-D\frac{\Delta C(x)}{\Delta z}$ must be constant through the entire interface. Since $D$ is already a fixed constant, we can assume $\frac{\Delta C(x)}{\Delta z}$ must also be constant. Thus the plot with a constant slope is the steady state profile which is B.

Q.E.D.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I agree with your analysis and choosing (B), however, the question suggests that diffusion only occurs from one side of the membrane so I think that the question is erroneous as none of the figures will fit this. Also no prohibition mentioned for material diffusing into the membrane. $\endgroup$
    – porphyrin
    Commented Mar 11, 2019 at 17:43
  • $\begingroup$ We aren't told whether the thin film is the same phase (e.g. a porous membrane) or a different phase than the bulk phases L and R. Without that information, it is hard to know if (a) or (b) is the correct answer, isn't it? E.g. if the film was a different phase with a different solubility / activity coefficient for the solute, wouldn't (a) be possible? $\endgroup$
    – Curt F.
    Commented Mar 11, 2019 at 18:21
  • $\begingroup$ @CurtF. You are correct that the underlying assumption of no difference in chemical potential is not trivial. I actually considered this before tendering an answer and it should be remembered that diffusion is ultimately driven by chemical potential not concentration. Part of why I chose to omit it is that the question itself seems a bit simplistic and thus chemical potential/activities would be too far. In answering a question of MaxW's made me realize that what we call diffusion across a membrane is actually effusion a physical process. $\endgroup$
    – A.K.
    Commented Mar 11, 2019 at 19:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.