I am fairly sure the first diagram I drew for carbon dioxide is wrong in terms of showing π bonding. This is because we use a π orbital twice, which isn't possible. The second diagram corrects this by realizing there are two unused p orbitals on the carbon.
EDIT: This is what I got for allene. Again we have an central $\ce{sp}$ carbon and this implies that two p-orbitals are free to form π bonds.
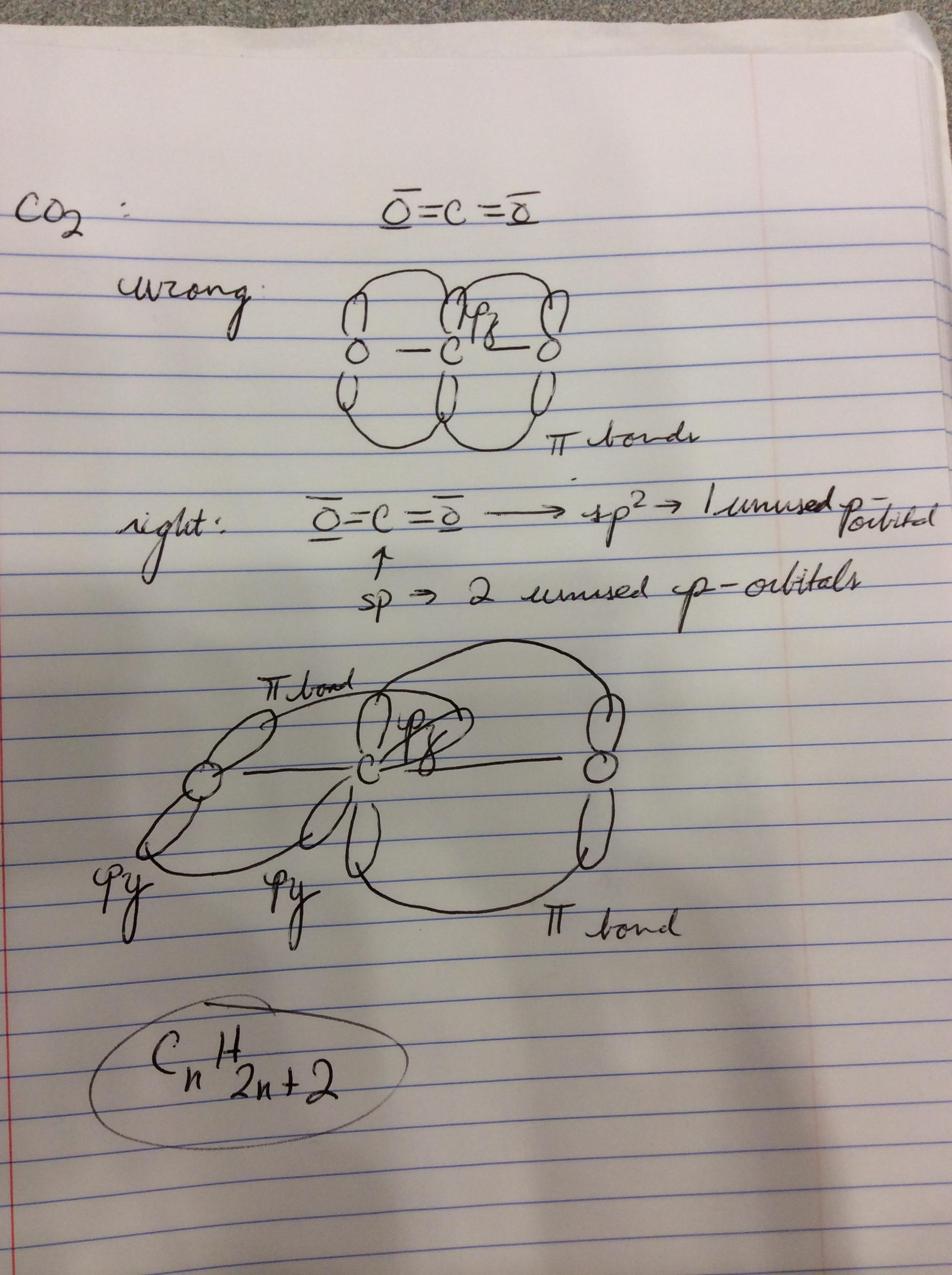
In terms of a simple bonding concept the answer of ron is correct, however due to symmetry constraints it is not possible to distinguish the two $\pi$ systems. The reason for this is, that these $\pi$ orbitals are degenerated and perpendicular to each other.
The molecule is very symmetric and one of only a few with the point group $D_{\infty h}$. That also imposes constraints on how the spatial distribution looks like.
Along the molecular axis ($\ce{O-C-O}$) is a rotational axis of infinite fold, $C_\infty$, as the molecule is linear. Perpendicular to that axis there is a horizontal mirror plane $\sigma_h$ passing through the centre of mass, i.e. the carbon. Additionally there is an infinite number of two-fold symmetry axis $C_2$ lying in that plane, which is the criterium for $D_{xh}$ point group. In line with the main axis there is also an infinite number of vertical mirror planes $\sigma_v$ (which is implicit due to the horizontal mirror plane).
For the sake of convenience we can define an upper and a lower half of the molecule (taking the cartesian $z$ axis as the main symmetry axis). These two halfs are indistinguishable, hence their orbitals must reflect that.
Having a look at the $\pi$-orbitals, clearly shows that (enlarge):
So your first drawing was not too wrong after all. The only thing you forgot is, that there is an identical orbital perpendicular to that.
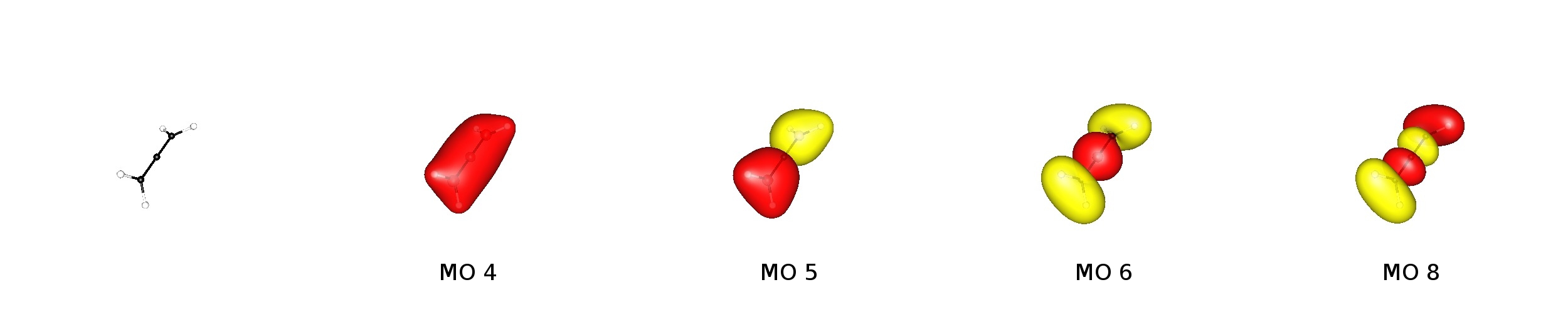
$\ce{CO2}$ is also a nice example to see the boundaries of the hybridisation concept. In this example, due to symmetry, all atoms have to be $\ce{sp}$ hybridised. And that matches all the $\sigma$ orbitals (enlarge).
The allene on the other hand is not linear and has a much lower symmetry. There is the main symmetry $C_2$ axis (for convenience) along the $\ce{C-C-C}$ axis, accompanied be two $\sigma_v$ mirror planes. There are also two diagonal symmetry planes $\sigma_d$ right between them. Perpendicular to the main symmetry axis we have two more $C_2$ axis (also perpendicular to each other). The point group is therefore $D_{2d}$ and has a lot less symmetry constraints.
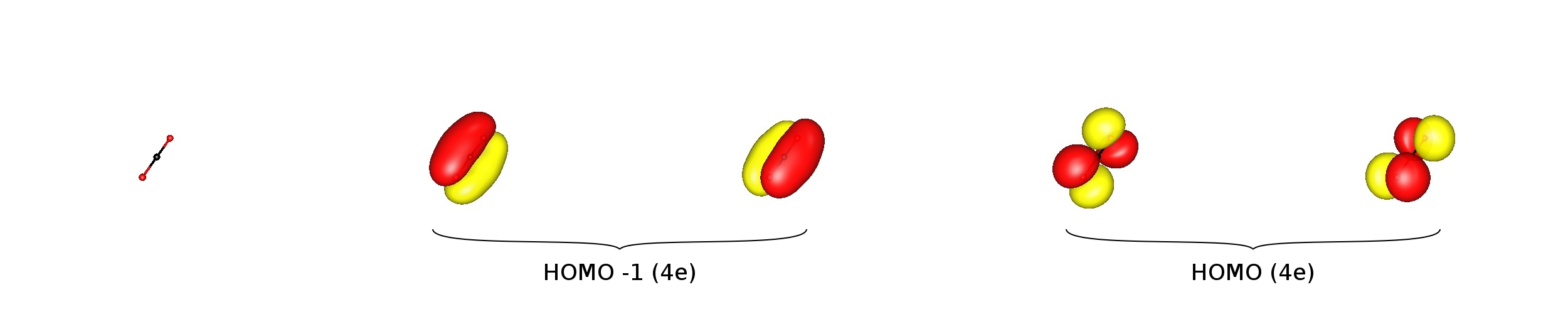
However, the orbitals are actually (surprisingly) quite similar though. We can define $\sigma$ and $\pi$ orbitals accordingly. The biggest distinction is still in the $\pi$-orbitals (enlarge).
The Lack of symmetry and therefore less constraints make is possible, that the orbitals combine in a different manner. The top and the lower half of the molecule does not have to be the same anymore, hence the orbitals are more localised to one bond in particular. A notably thing is, that hydrogens take part in $\pi$ bonding (Hyperconjugation).
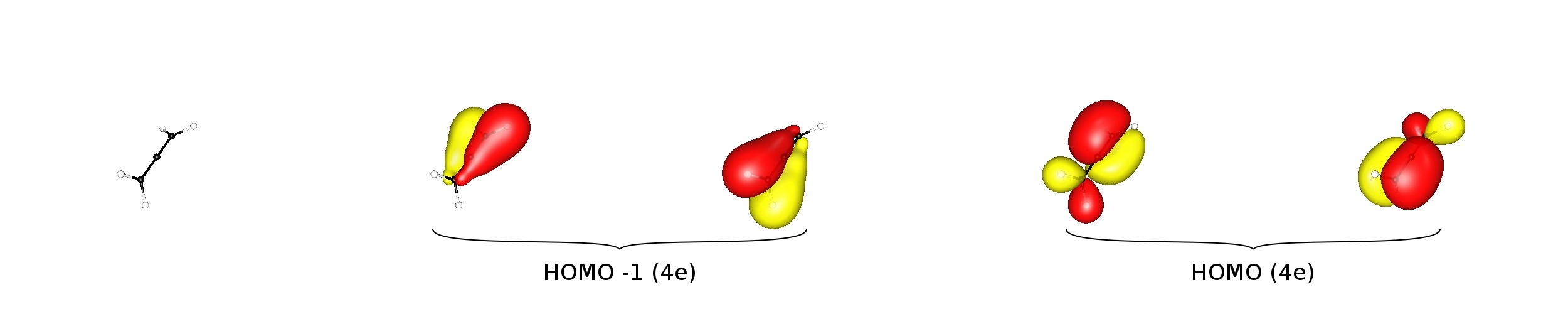
The sigma orbitals are quite the same as in $\ce{CO2}$ (enlarge).
However, the traditional bonding picture of localised orbitals is quite valid here. The central carbon is still being $\ce{sp}$ hybridised and the moieties are $\ce{sp^2}$ hybridised.
Ketene is a combination of the two above, with even lower symmetry ($C_{2v}$). Therefore the conventional bonding picture should hold.
For Oxygen it is usually always good to check, if hybridisation is a good concept to describe the bond. When bonded to more than one partner, the answer is usually yes. If less, then there is a good chance, that hyperconjugative effects outweigh the information gained from the hybridisation concept.
Additional fun note
One could argue that the carbon suboxide ($\ce{C3O2}$) is not too different, but here again you will be surprised, as it has a very shallow bending potential. (crystal structure, vibrations)
I wish to synthesize a new answer by combining Martin and ron's answers. I think the real issue is to ensure that the cylindrical symmetry of the electron density of $\ce{CO_2}$ is preserved: that is, $\ce{CO_2}$ should be like a "rod".
In the image below, I have drawn two "resonance structures" (valence bond configurations) that must be combined in order to generate the cylindrical symmetry of the electron density, while maximizing the conceptual relationship with resonance structures. I have chosen to use a) unhybridized C and b) two ${sp}^2$ fragments for the O's.
A small missive: Of course, multiconfigurational MO theory and multiconfigurational VB theory are equivalent. However, certain problems can be describe most economically in either MO theory or VB theory. This is a case where the electronic structure is probably easiest in MO theory, since it requires only one configuration to have cylindrical symmetry (+1 for Martin's answer).
But your question is about "resonance structures" for $\ce{CO_2}$ (+1 for ron's answer), but the constraints on the density mandate the use of the admixture of two resonance structures. If we use only one resonance structure, it is easy to see from the lone pairs that the density would not be cylindrically symmetric!
(Please note that I am not sure about the sign of the combination, it may be - since we are assuming a singlet state. And maybe I am overthinking it. Perhaps it should be a double-headed arrow for resonance structures.)
Your second drawing is correct. The bonding scheme in carbon dioxide is the same as that in allene and ketene in that the two pi systems are orthogonal. Draw or build a model of allene and notice what effect the orthogonality has on the two planes the hydrogens lie in.
