Why do the melting and boiling points of the noble gases increase when the atomic number increases?

Question

What causes the melting and boiling points of noble gases to rise when the atomic number increases? What role do the valence electrons play in this?

Answer 1

The melting and boiling points of noble gases are very low in comparison to those of other substances of comparable atomic and molecular masses. This indicates that only weak van der Waals forces or weak London dispersion forces are present between the atoms of the noble gases in the liquid or the solid state.

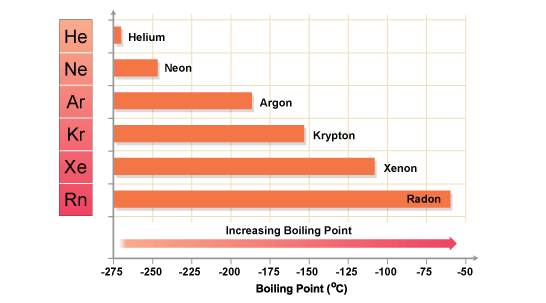
The van der Waals force increases with the increase in the size of the atom, and therefore, in general, the boiling and melting points increase from $\ce{He}$ to $\ce{Rn}$.

Helium boils at $-269\ \mathrm{^\circ C}$. Argon has larger mass than helium and have larger dispersion forces. Because of larger size the outer electrons are less tightly held in the larger atoms so that instantaneous dipoles are more easily induced resulting in greater interaction between argon atoms. Therefore, its boiling point ($-186\ \mathrm{^\circ C}$) is more than that of $\ce{He}$.

Similarly, because of increased dispersion forces, the boiling and melting points of monoatomic noble gases increase from helium to radon.

---

For more data of melting and boiling points of noble gas compounds, read this page

Answer 2

Other answers have mentioned that dispersion forces are the key to answering the question but not how they increase from helium to radon (or let’s take xenon because that’s not radioactive so I feel safer breathing it).

The larger the mass of a nucleus the more protons are in there, and the more protons in a nucleus the more electrons are around the outside. Traditionally, one thought of electrons orbiting the nucleus rather like satellites orbiting a planet on more or less fixed orbits at certain heights. But that picture is wrong. It is much better to consider electrons as waves that completely surround the nucleus. If one were to translate these waves into particles (because of the wave-particle dualism at quantum levels), all one would get would be probabilities of finding specific electron $e$ at specific location $x$.

For a neutral atom that is surrounded by nothing, these probabilities depend only on the wave function, and are inherently centrosymmetric or anticentrosymmetric, leading to a net charge distribution of zero. In a sample of xenon gas, however, other atoms approach the atom we are observing. Consider an atom approaching our xenon atom at from ‘above’ (i.e. perpendicular to our viewpoint). While the atom as a whole is neutral, the could of electrons surrounding the nucleus is negatively charged. This new negative charge changes the potential energy the electrons in our observed atom are perceiving: While we originally had a centrosymmetric potential distribution (decreasing positive charge intensity from the nucleus) we now have a second negative source at 12 o’clock. Therefore, the probability distributions will shift ever so slightly and it will be slightly more likely to detect our electron $e$ at position $y$ below the nucleus rather than at position $z$ above the nucleus. Note that this simplification relies on the freezing of time at a certain moment when the other atom is approaching and a certain controlled environment that I have invented for example purposes.

With the electrons now more likely to be at $y$ rather than $z$, we can say that we have created a spontaneous or an induced dipole. A mild positive charge is now pointing towards the other atom and ever so slightly attracting it. This will, if you advance the time flow by one spec, create another induced dipole in the originally approaching atom plus further in every other atom that is close around. We cannot freeze this picture though. Every infinitesimal change in position, movement direction or rotation will change the entire picture, meaning that our induced dipole is extremely short-lived.

It is only the combination of all these induced dipoles and their slight attraction that attracks the different atoms together. Since they rely on electron distribution changing, the more electrons we have the stronger these induced dipoles can be and the more force can be excersized between atoms. Since the number of electrons loosely correlates with mass (and strictly correlates with nuclear charge), larger atoms are said to display stronger van der Waals forces than smaller ones.

Answer 3

Valence electrons have not much to do with this, as their outer shell is closed. As the other answer mentioned, dispersion forces are the ones responsible for any interaction between these atoms.

The size dependence therefore is directly coming from the size dependence of dispersion forces:

In a very simplistic way, a random charge fluctuation can polarize the otherwise perfectly apolar atoms. This induced dipole moment is then responsible for the dispersion interactions. The polarizibility of an atom increases (easier to polarize) if the atomic number increases, therefore the interactions in nobel gases will reflect this behavior.

Answer 4

As mentioned in other answers the dispersion force is responsible for noble gases forming liquids. The calculation of the boiling points is now outlined after some general comments about the dispersion force.

The dispersion force (also called London, charge-fluctuation, induced-dipole-induced-dipole force) is universal, just like gravity, as it acts between all atoms and molecules. The dipole forces can be long-range, >10 nm down to approx 0.2 nm depending on circumstances, and can be attractive or repulsive.

Although the dispersion force is quantum mechanical in origin it can be understood as follows: for a non-polar atom such as argon the time average dipole is zero, yet at any instance there is a finite dipole given by the instantaneous positions of the electrons relative to the nucleus. This instantaneous dipole generates an electric field that can polarise another nearly atom and so induce a dipole in it. The resulting interaction between these two dipoles gives rise to an instantaneous attractive force between the two atoms, whose time average in not zero.

The dispersion energy was derived by London in 1930 using quantum mechanical perturbation theory. The result is

$$U(r)=-\frac{3}{2}\frac{\alpha_0^2I}{(4\pi\epsilon _0)^2r^6}=-\frac{C_{\mathrm{disp}}}{r^6}$$

where $\alpha_0$ is the electronic polarisability, $I$ the first ionisation energy, $\epsilon_0$ the permittivity of free space and $r$ the separation of the atoms. The electronic polarisability $\alpha_0$ arises from the displacement of an atom's electrons relative to the nucleus and it is the constant of proportionality between the induced dipole and the electric field $E$, viz., $\mu_{\mathrm{ind}} = \alpha_0 E$. The polarisability has units of $\pu{J-1 C2 m2}$, which means that in SI units $\alpha_0/(4\pi\epsilon_0)$ has units of $\pu{m3}$ and this polarisability is in effect a measure of electronic volume, or put another way $\alpha_0 = 4\pi\epsilon_0r_0^3$ where experimentally it is found that $r_0$ is approximately the atomic radii. The ionisation energy $I$ arises because to estimate $r_0$ a simple model of an atom is used to calculate the orbital energy and hence radius and in doing so the energy is equated to the ionisation energy since this can be measured.

As can be seen from the formula the energy depends on the product of the square of the polarisability, i.e. volume of molecule or atom and its ionisation energy, and also on the reciprocal of the sixth power of the separation of the molecules/atoms. In a liquid of noble gases this separation may be taken to be the atomic radius, $r_0$. Thus the dependence is much more complex than just size, see table of values below. The increase in polarisability as the atomic number increases, is offset somewhat by the reduction in ionisation energy and increase in atomic radius.

If experimental values are put into the London equation then the attractive energy can be calculated. In addition the boiling point can be estimated by equating the London energy with the average thermal energy as $U(r_0)=3k_\mathrm{B}T/2$ where $k_\mathrm B$ is the Boltzmann constant and $T$ the temperature. The relevant parameters are given in the table below, with values in parentheses being experimental values:^[1]

$$\begin{array}{c|c|c|c|c|c} \text{Noble gas} & (\alpha_0/4\pi\epsilon_0)~/~\pu{10^{-30}m^3} & I~/~\pu{eV} & r_0~/~\pu{nm} & C_\mathrm{disp}~/~\pu{10^{-70} J m6} & T_\mathrm{b}~/~\pu{K} \\ \hline \ce{Ne} & 0.39 & 21.6 & 0.308 & 3.9~(3.8) & 22~(27) \\ \ce{Ar} & 1.63 & 15.8 & 0.376 & 50~(45) & 85~(87) \\ \ce{Xe} & 4.01 & 12.1 & 0.432 & 233~(225) & 173~(165) \end{array}$$

The fit to data is very good, possibly this is fortuitous, but these are spherical atoms showing only dispersion forces and a good correlation to experiment is expected. However, there short range repulsive forces that are ignored as well as higher order attractive forces. Nevertheless it does demonstrate that dispersion forces can account for the trend in boiling quite successfully.

---

1. Israelachvili, J. N. Intermolecular and Surface Forces, 3rd ed.; Academic Press: Burlington, MA, 2011; p 110.