When is a reaction reversible?

Question

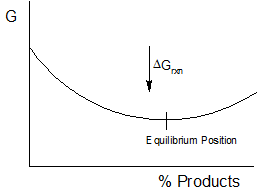
When speaking in thermodynamic terms, when is a reaction reversible?

My attempt: A reaction is reversible when $\Delta\text{S} < 0$, because according to the second law of thermodynamics, "in every natural thermodynamic process the sum of the entropies of all participating bodies is increased" (Wikipedia.org), therefore if a reaction is caused and has negative change in entropy, a reversed reaction should be natural.

However, this is not the answer that is absolutely correct, nor a rigid solution or a rule. Can anyone help me out? (I have searched on the internet, and although thermodynamic reasons are preferred, I do not mind answers to be molar or solution chemistry, as long as a thermodynamic approach is included as well or a statement that there is no apparent thermodynamic rule that can be applied to this question.)

Answer 1

"Reversible" is not binary. Both the forward and backward reactions always occur and the equilibrium system never has zero reactants or zero products. Thus, irreversible reactions are called this not because they cannot be reversed - they absolutely can - but because reversal is impractical. The equilibrium constant may be so skewed toward product that the reactant quantities at equilibrium would be undetectable. The products may be gases that escape solid reactants, which ensures that the system never reaches equilibrium in the open anyway. There may be a lot of side reactions on the products.

A "reversible" reaction just lacks factors like this so that we can have measurable quantities of reactant beside measurable quantities of product at equilibrium. We add some reactant, watch the reaction go forward. We add some product, it goes in reverse. See? It's reversible. There is no exact cutoff in any measure.

Answer 2

To add to @sqykly's excellent answer, the equilibrium constant, which represents the degree to which a reaction may be considered 'reversible', is related to the Gibbs free energy change for the reaction by: $$\Delta G_\text{rxn} = -RT\ln K$$

A very large equilibrium constant, which corresponds to a large negative $\Delta G_\text{rxn}$, essentially means that the reaction goes to completion. A very small equilibium constant, which corresponds to a very large positive $\Delta G_\text{rxn}$, means that the reaction essentially does not occur.

However, as we can see from the graph above, the equilibrium position will always have some amount of reactants and products present because the shape of the curve is the same for all reactions, only with different values. The reaction shown is exergonic (it has a negative $\Delta G_\text{rxn}$) but an endergonic reaction will have the same profile, only the position of 100% products will have a higher Gibbs energy than that of 100% reactants.

A 'reversible' reaction is one which has an equilibrium constant somewhere in the middle and so changing the concentration of reactants or products has a significant effect on the position of equilibrium. Generally, a reaction where $|\Delta G_\text{rxn}| < 30 \mathrm{kJ~mol^{-1}}$, can be considered reversible. At $\mathrm{298~K}$ this corresponds to $5 \times 10^{-6} < K < 2 \times 10^5$. Interestingly, this means that even if the equilibrium constant is less than one (i.e. $\Delta G_\text{rxn} > 0$) then you can still extract useful products from a reaction by removing them as they form.

Answer 3

You're mixing two things - from thermodynamic point of view any process would be reversible when $\Delta\text{S} = 0$ for whole isolated system; which never really happens.

What sqykly is saying is about chemical reactions, which are reversible in the sense that they can be reversed from chemical point of view concerning substrates and products, not energy and entropy.

Answer 4

Another associated issue that often arises is the criterion for a reaction to be spontaneous. The criterion that, at constant temperature, if a reaction is spontaneous, $\Delta G^0$ in going from pure reactants to pure products at 1 bar will be negative is just a rule of thumb which, over the years, has confused students to no end. If you start out with pure reactants and mix them, spontaneous reaction will always occur to some extent. It's just that, if $\Delta G^0$ is positive, the reaction will tend to proceed to a lesser extent when equilibrating, and if $\Delta G^0$ is negative, it will tend to proceed to a greater extent when equilibrating. So, in this sense, every reaction is spontaneous.