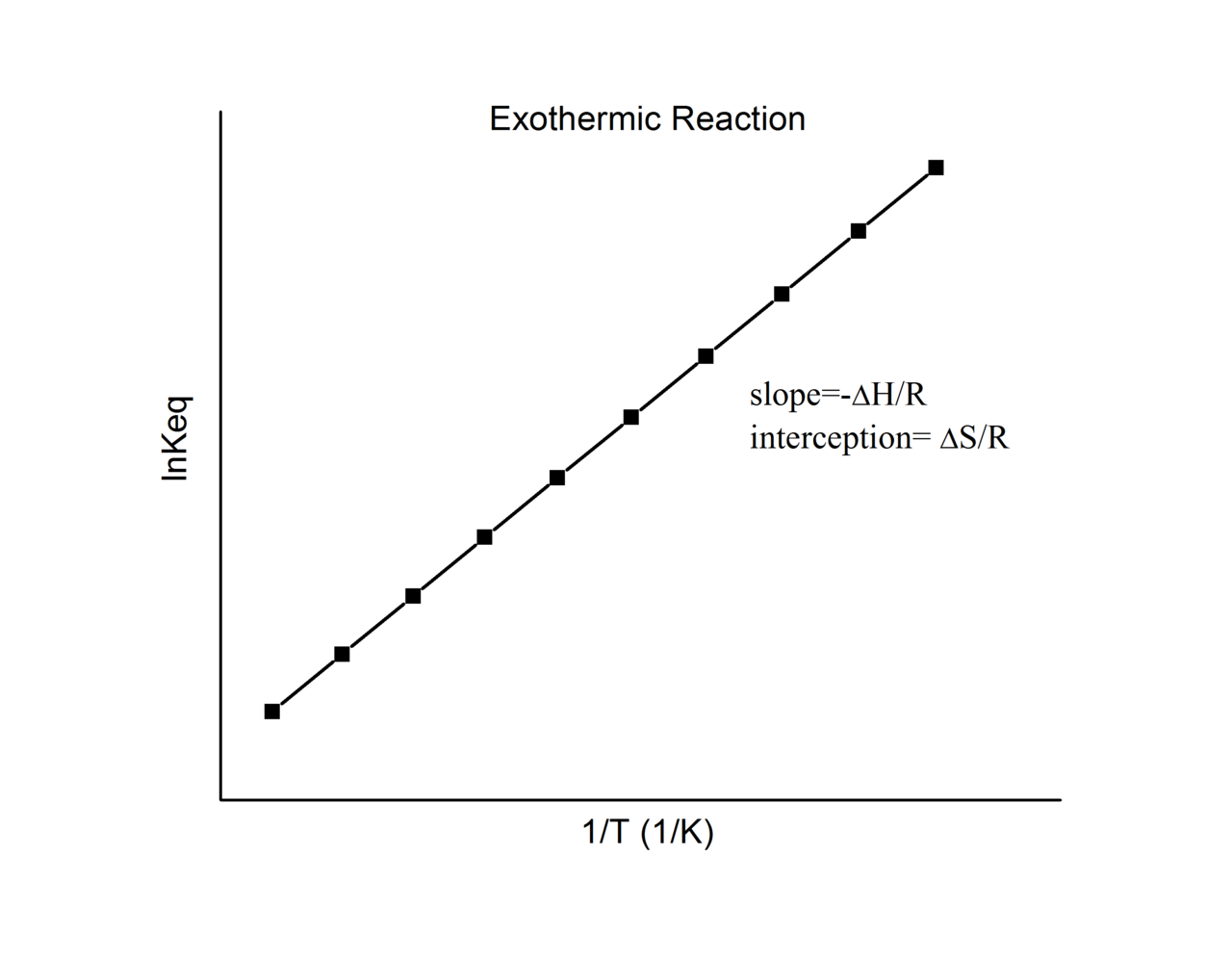
Suppose a reaction had a positve entropy change and a negative enthalpy change.
According to Le Chatelier's principle, the reaction should be less favorable at higher temperatures, as heat is released. The same is applicable when looking at a Van't Hoff plot:
However, the entropy change is also positive, and as
$\Delta G=\Delta H-T\Delta S$
one would expect the gibbs energy to decrease at higher temperatures, therefore increasing the reaction's equilibrium constant:
$K=e^{\frac{-G^{0}}{RT}}$
So what is going on here, why do those two statements contracdictcontradict each other?