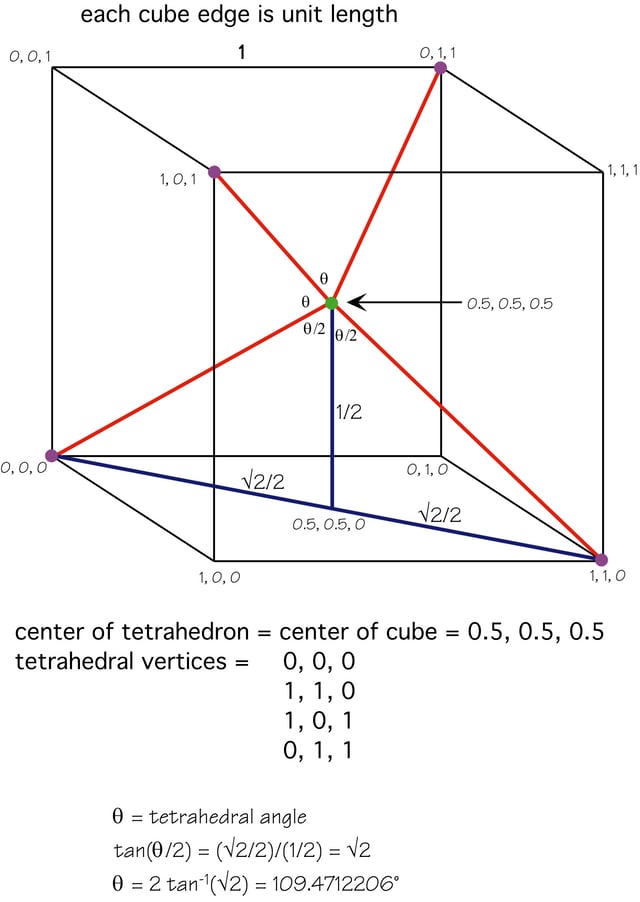
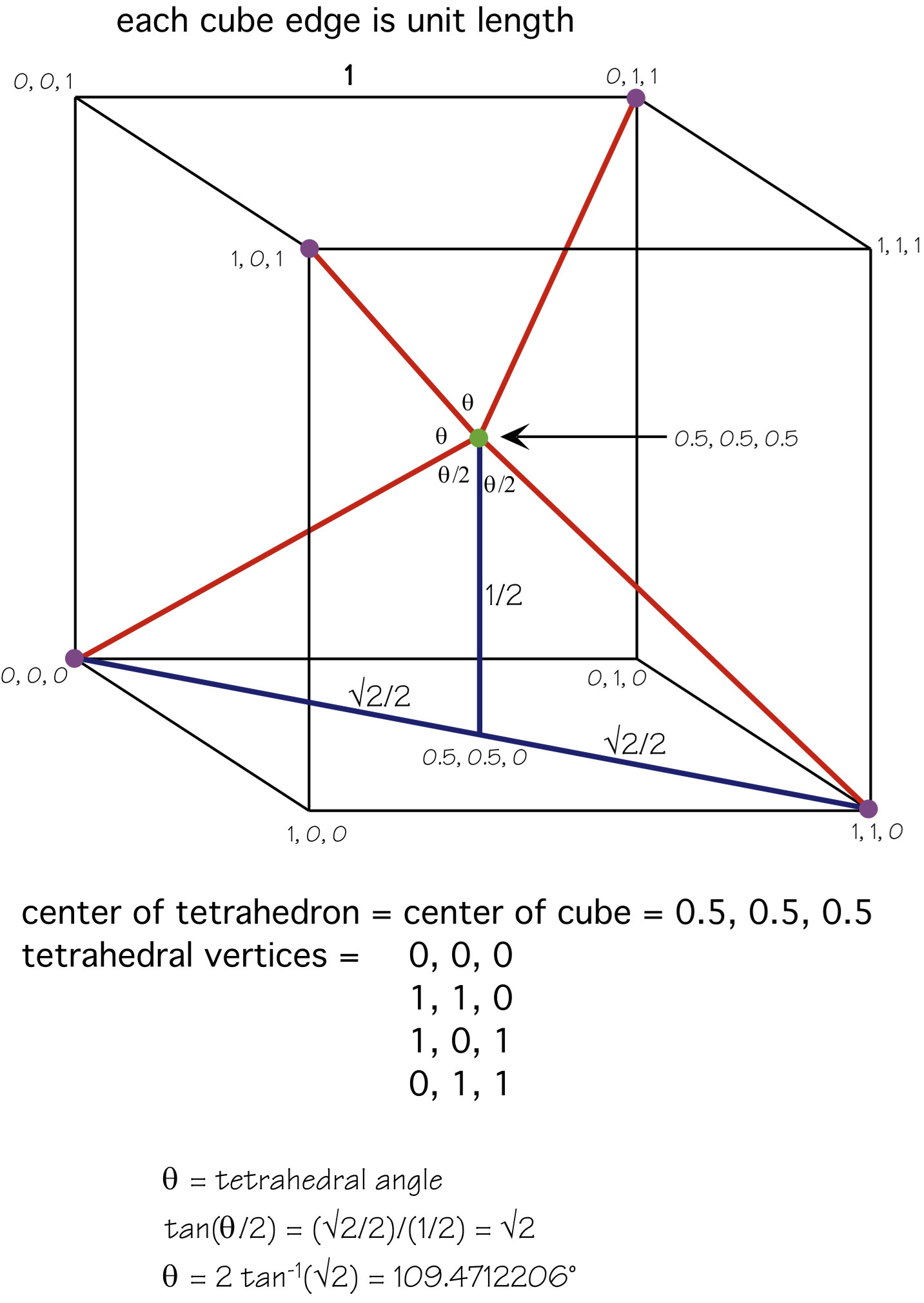
Karsten's answer is excellent, but here is a figure that shows the mathemathicsmathematics involved:
The central atom (green) is at the center of the cube, the four other atoms (purple) are at alternating vertices and the geometry should be clear.
Alternatively, if you orient the molecule so one peripheral (purple) atom is directly "above" the central (green) atom, then each of the other three atoms is just $1 - \theta$ ($\approx 70.52877940 ^\circ$) away from being directly "under" the central atom, so each contributes $\cos(1 - \theta)$ times the bond dipole. This is the downward component of the bond dipole from one of the lower atoms.
But $\cos(1 - \theta) = 1/3$, so this is simply (bond dipole)/3, and there are three of these lower atoms, so the three downward components exactly balance the one upward component.