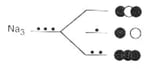
The solutions to the 1D particle in a box quantum mechanic system are standing waves (zero at both ends of the box) with 0,1,2... nodes for increasing energy (zero for the ground state).
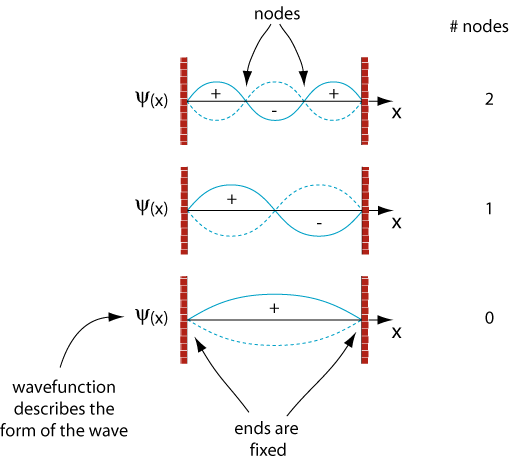

If I look at the LCAO solutions for a linear system, combining AOs of the same shape and energy (e.g. conjugated double bonds, linear array of sodium atoms), the coefficients follow the pattern of a standing wave with 0,1,2... nodes. For example, when I combine 3 AO's in a row, the solutions are +++, +0-, +-+, i.e. zero, one, and two switches of signs as I go to higher energy states. For the state with one node, the node is in the center of the box, corresponding to the middle AO, which has a coefficient of zero. (More examples, i.e. hexatriene and pentadienyl cation, are on slide 126 and 128 of this document.)
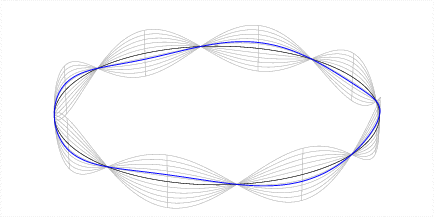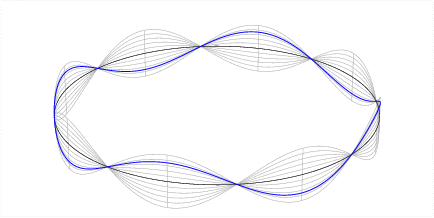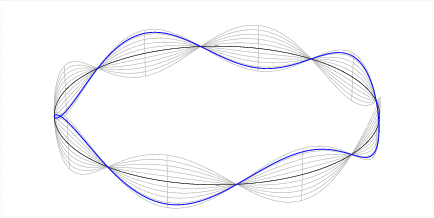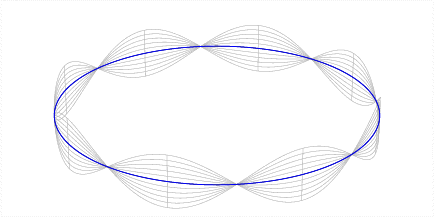
The solutions to the particle in a ring system are standing circular waves (same value at 0 and 360 degrees) with 0,2,4... nodes for increasing energy. The animation shows a standing circular wave with 8 nodes:
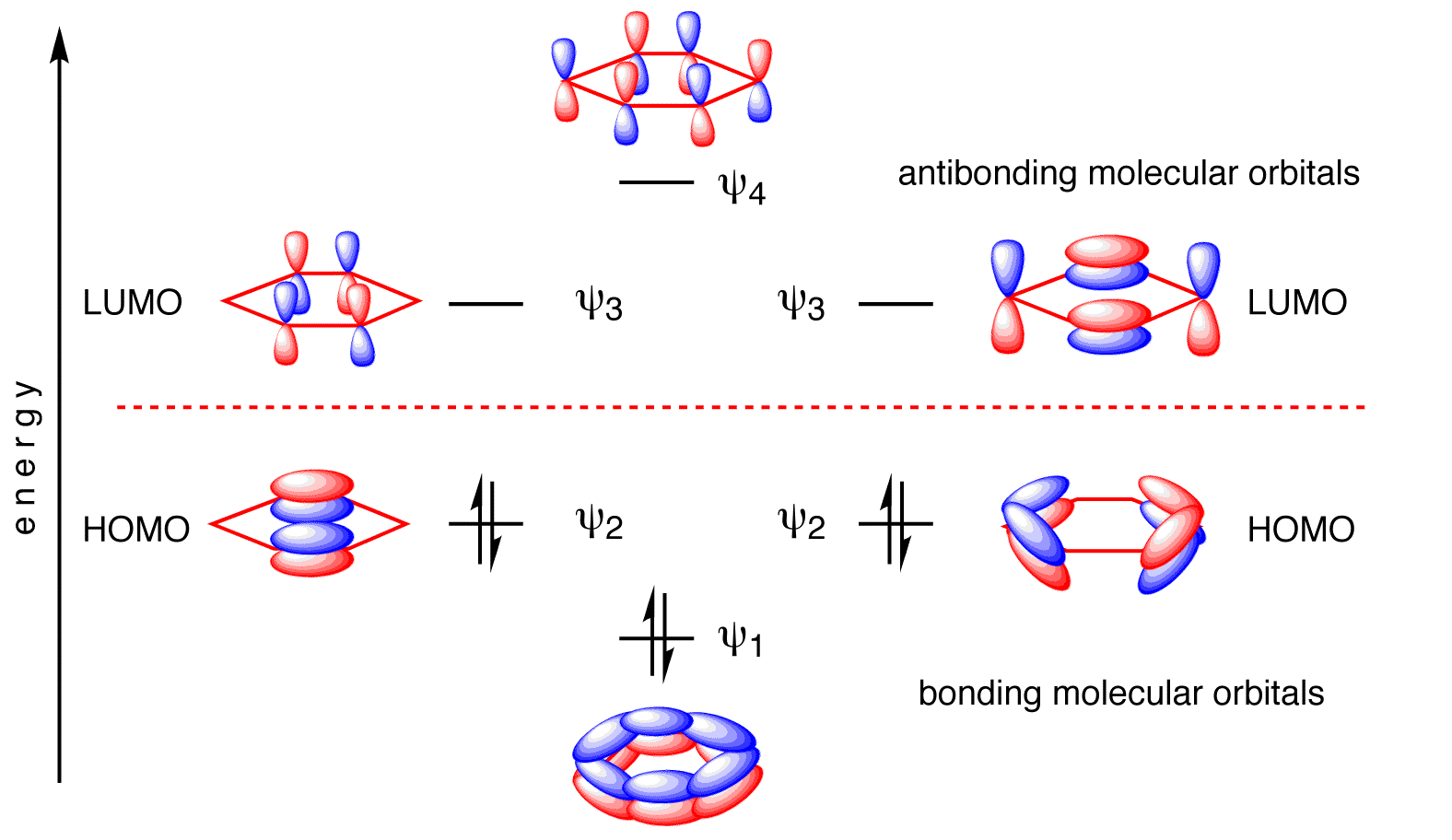
If I look at the LCAO solutions for a circular system, again combining AOs of the same shape and energy (e.g. p-orbitals in an aromatic system), the coefficents follow the pattern of a circular wave with 0,2,4... nodes. For benzene, the solutions are ++++++, +++--- and +0--0+, +-++-+ and -0+-0+, +-+-+- (see picture):
What underlies the connection between the unbound electron in a box or corral with the formation of covalent bonds of bound electrons?